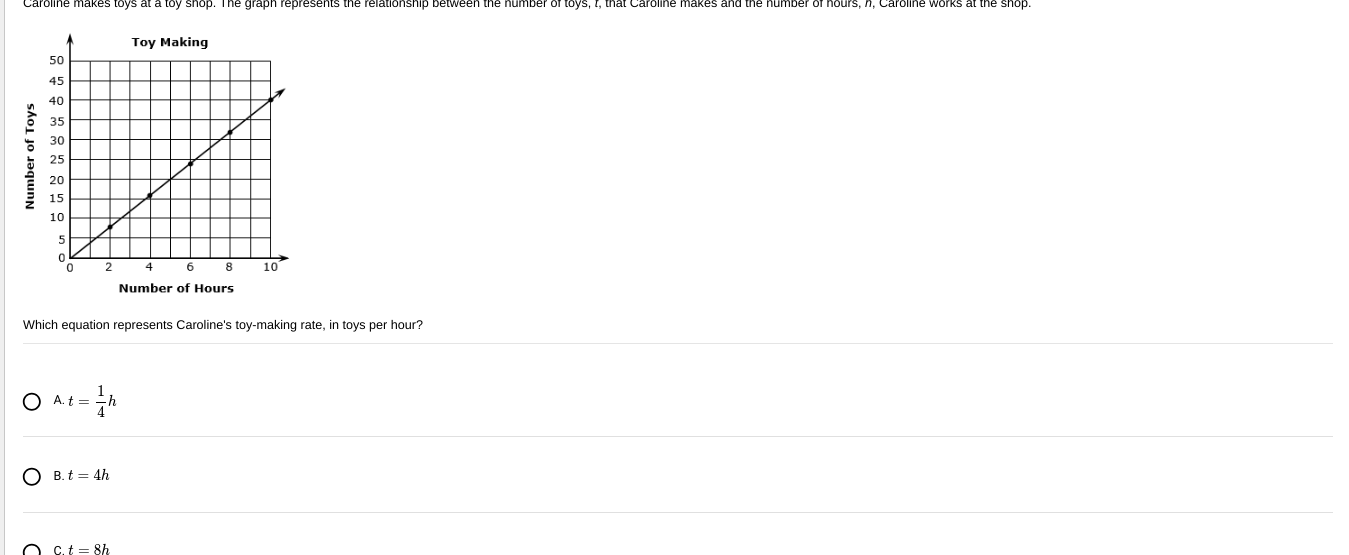
Which equation represents Caroline's toy-making rate, in toys per hour?
Understand the Problem
The question is asking which equation best represents the relationship between the number of toys Caroline makes and the number of hours she works, based on a provided graph. The user needs to determine the toy-making rate in toys per hour.
Answer
The correct expression for Caroline's toy-making rate is \( t = 4h \).
Answer for screen readers
The correct equation representing Caroline's toy-making rate is ( t = 4h ).
Steps to Solve
- Identify the Rates from the Graph
From the graph, you should identify how many toys are made for each hour of work. Check the coordinates: if, for example, at 8 hours Caroline makes 32 toys, then the toy-making rate can be calculated.
- Calculate the Toy-Making Rate
Assume that Caroline makes 40 toys in 10 hours (look for a point on the line in the graph). The toy-making rate can be calculated as:
$$ \text{Rate} = \frac{\text{Number of Toys}}{\text{Number of Hours}} = \frac{40}{10} = 4 \text{ toys per hour} $$
- Express the Rate in Equation Form
Using the calculated rate, you can express the relation between the number of toys $t$ and the number of hours $h$ as:
$$ t = 4h $$
This means that for every hour worked, Caroline makes 4 toys.
- Match with Given Options
Now, compare the found equation $t = 4h$ with the options provided:
- A: $t = \frac{1}{4}h$
- B: $t = 4h$
- C: $t = 8h$
The correct option is B, as it matches the toy-making equation derived from the graph.
The correct equation representing Caroline's toy-making rate is ( t = 4h ).
More Information
This equation indicates that for every hour Caroline works, she produces 4 toys. This is a direct relationship between time spent working and toys made, embodying the concept of a constant rate of production.
Tips
Some common mistakes include:
- Misreading the graph or not identifying the points correctly.
- Confusing the rate with the total output. Instead of calculating the rate, some might miscalculate using the total number of toys instead of dividing by hours.
AI-generated content may contain errors. Please verify critical information