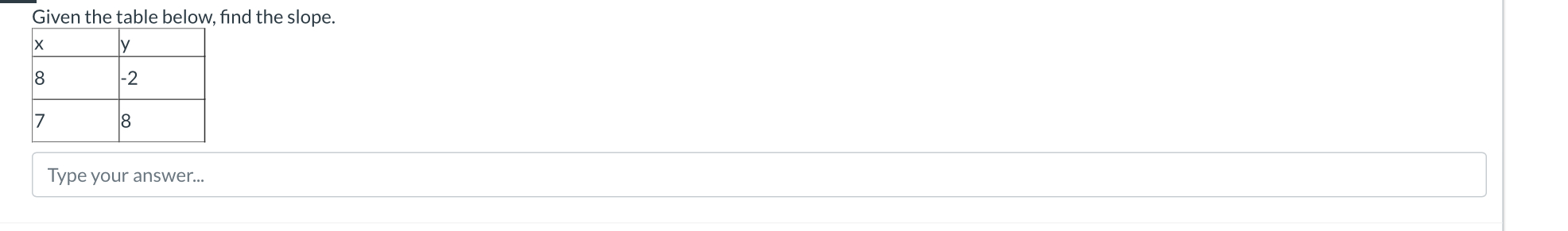
Given the table below, find the slope.

Understand the Problem

The question asks to calculate the slope based on the values provided in the table, which includes pairs of x and y coordinates.

Answer

The slope is $ m = -10 $.

Steps to Solve

Identify the Coordinates

From the table, we have the following two points:

Point 1: ( (8, -2) )
Point 2: ( (7, 8) )

Use the Slope Formula

The formula to calculate the slope ( m ) between two points ( (x_1, y_1) ) and ( (x_2, y_2) ) is given by:

$$ m = \frac{y_2 - y_1}{x_2 - x_1} $$

Here, we will assign:

( (x_1, y_1) = (8, -2) )
( (x_2, y_2) = (7, 8) )

Substitute the Values into the Formula

Plugging in the values into the slope formula:

$$ m = \frac{8 - (-2)}{7 - 8} $$

Simplify the Calculation

Calculate the difference in the numerator and denominator:

$$ m = \frac{8 + 2}{7 - 8} = \frac{10}{-1} $$

Final Calculation

This simplifies to:

$$ m = -10 $$

The slope is ( m = -10 ).

More Information

The slope of a line represents how steep the line is. A negative slope indicates that the line decreases as it moves from left to right. In this case, a slope of -10 means that for every unit moved to the right, the line drops 10 units.

Tips

Confusing the order of points; ensure you use the correct ( (x_1, y_1) ) and ( (x_2, y_2) ).
Forgetting to subtract the values in the correct order in the slope formula.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!