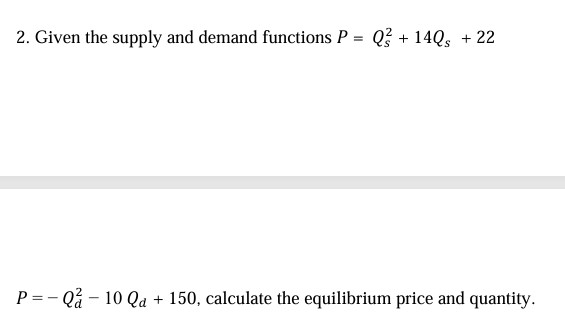
Given the supply and demand functions P = Qs^2 + 14Qs + 22 and P = -Qd^2 - 10Qd + 150, calculate the equilibrium price and quantity.

Steps to Solve

1. Set Supply Equal to Demand

To find the equilibrium price and quantity, set the supply function equal to the demand function:

$$ Q_s^2 + 14Q_s + 22 = -Q_d^2 - 10Q_d + 150 $$

2. Rearrange the Equation

Rearranging the above equation to bring all terms to one side gives:

$$ Q_s^2 + 14Q_s + Q_d^2 + 10Q_d - 128 = 0 $$

3. Assume $Q_s = Q_d$ for Equilibrium

Set (Q_s = Q_d = Q):

$$ Q^2 + 14Q + Q^2 + 10Q - 128 = 0 $$

This simplifies to:

$$ 2Q^2 + 24Q - 128 = 0 $$

4. Solve the Quadratic Equation

Using the quadratic formula (Q = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}) where (a = 2), (b = 24), and (c = -128):

$$ Q = \frac{-24 \pm \sqrt{24^2 - 4 \cdot 2 \cdot (-128)}}{2 \cdot 2} $$

5. Calculate the Discriminant

Calculate (b^2 - 4ac):

$$ 24^2 - 4 \cdot 2 \cdot (-128) = 576 + 1024 = 1600 $$

6. Find the Values of Q

Compute the roots:

$$ Q = \frac{-24 \pm 40}{4} $$

Calculating the two possible values:

• For (Q = \frac{16}{4} = 4)
• For (Q = \frac{-64}{4} = -16) (not valid since quantity cannot be negative)

Thus, (Q = 4).

7. Substitute Q back to find P

Substitute (Q = 4) into either supply or demand function to find the equilibrium price:

Using the supply function:

$$ P = Q^2 + 14Q + 22 = 4^2 + 14 \cdot 4 + 22 = 16 + 56 + 22 = 94 $$