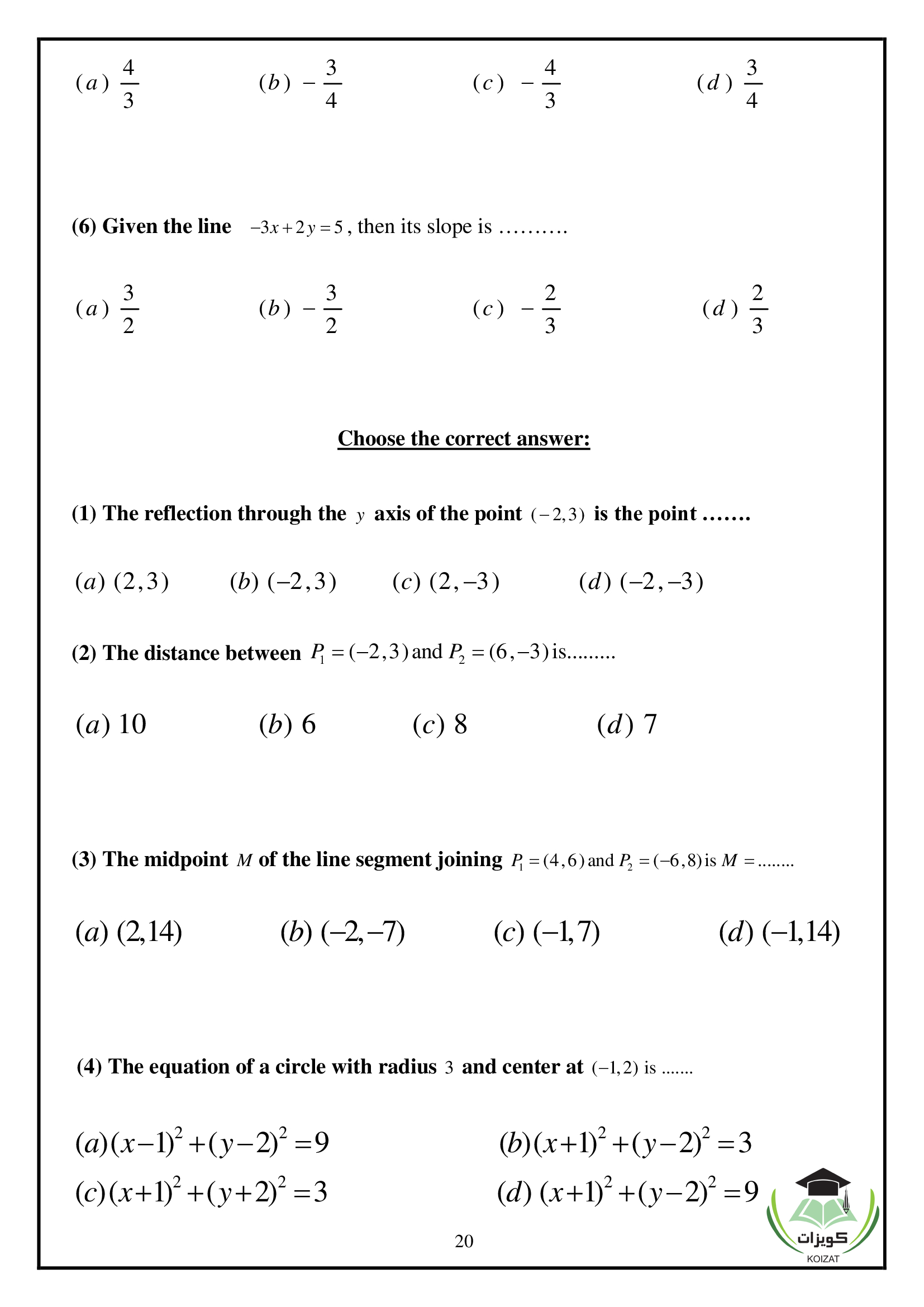
Given the line -3x + 2y = 5, then its slope is; The reflection through the y-axis of the point (-2, 3) is…; The distance between P1 = (-2, 3) and P2 = (6, -3) is…; The midpoint M o... Given the line -3x + 2y = 5, then its slope is; The reflection through the y-axis of the point (-2, 3) is…; The distance between P1 = (-2, 3) and P2 = (6, -3) is…; The midpoint M of the line segment joining P1 = (4,6) and P2 = (-6,8) is…; The equation of a circle with radius 3 and center at (-1,2) is…
Understand the Problem
The question contains multiple math problems involving slopes, reflections, distances, midpoints, and circle equations. Each problem requires applying specific mathematical concepts to choose the correct answer.
Answer
1. \( \frac{3}{2} \) 2. \( (2, 3) \) 3. \( 10 \) 4. \( (-1, 7) \) 5. \( (x + 1)^2 + (y - 2)^2 = 9 \)
Answer for screen readers
-
Slope of the line is ( \frac{3}{2} )
-
Reflection of the point ((-2, 3)) is ((2, 3))
-
Distance between points ( P_1 ) and ( P_2 ) is ( 10 )
-
Midpoint ( M ) is ((-1, 7))
-
Equation of the circle is ( (x + 1)^2 + (y - 2)^2 = 9 )
Steps to Solve
-
Finding the Slope of the Line To find the slope from the equation ( -3x + 2y = 5 ), rearrange it into slope-intercept form ( y = mx + b ): [ 2y = 3x + 5 \implies y = \frac{3}{2}x + \frac{5}{2} ] The slope ( m ) is ( \frac{3}{2} ).
-
Finding the Reflection Through the y-axis To find the reflection of the point ((-2, 3)) through the ( y )-axis, change the sign of the ( x )-coordinate: [ (-2, 3) \to (2, 3) ]
-
Calculating the Distance Between Two Points Use the distance formula ( d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} ): [ P_1 = (-2, 3), P_2 = (6, -3) ] Calculate: [ d = \sqrt{(6 - (-2))^2 + (-3 - 3)^2} = \sqrt{(6 + 2)^2 + (-6)^2} = \sqrt{8^2 + 6^2} = \sqrt{64 + 36} = \sqrt{100} = 10 ]
-
Finding the Midpoint of a Line Segment The midpoint ( M ) of two points ( P_1 = (4, 6) ) and ( P_2 = (-6, 8) ) is given by: [ M = \left( \frac{x_1 + x_2}{2}, \frac{y_1 + y_2}{2} \right) = \left( \frac{4 + (-6)}{2}, \frac{6 + 8}{2} \right) = \left( -1, 7 \right) ]
-
Finding the Equation of the Circle The standard form of a circle's equation is ( (x - h)^2 + (y - k)^2 = r^2 ). Given center ((-1, 2)) and radius (3): [ (x + 1)^2 + (y - 2)^2 = 3^2 = 9 ]
-
Slope of the line is ( \frac{3}{2} )
-
Reflection of the point ((-2, 3)) is ((2, 3))
-
Distance between points ( P_1 ) and ( P_2 ) is ( 10 )
-
Midpoint ( M ) is ((-1, 7))
-
Equation of the circle is ( (x + 1)^2 + (y - 2)^2 = 9 )
More Information
Each problem applies fundamental concepts in geometry and algebra, including understanding slopes, reflections, distances, midpoints, and circle equations.
Tips
- Confusing the signs when calculating reflections, especially with the ( y )-axis.
- Misapplying the distance formula by omitting square roots or forgetting to square differences.
- Incorrectly calculating midpoints by averaging coordinates incorrectly.
AI-generated content may contain errors. Please verify critical information