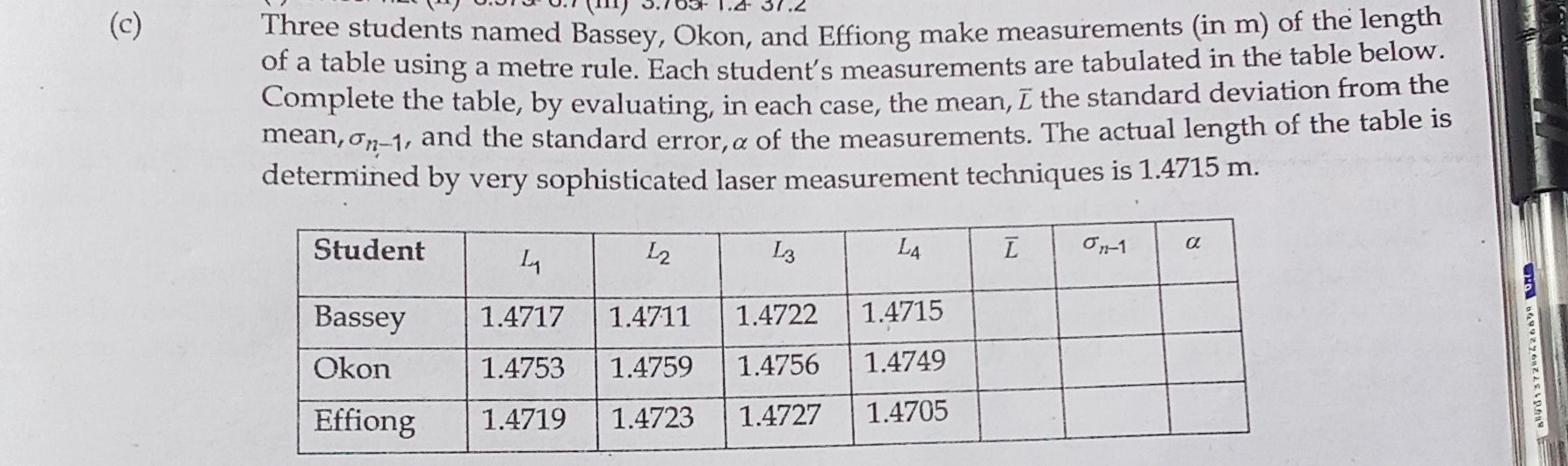
Three students named Bassey, Okon, and Effiong make measurements (in m) of the length of a table using a metre rule. Complete the table by evaluating, in each case, the mean, the s... Three students named Bassey, Okon, and Effiong make measurements (in m) of the length of a table using a metre rule. Complete the table by evaluating, in each case, the mean, the standard deviation from the mean, and the standard error of the measurements.
Understand the Problem
The question is asking to complete a table with measurements taken by three students, where we need to calculate the mean, standard deviation, and standard error of their measurements.
Answer
The completed table shows the mean, standard deviation, and standard error for each student's measurements.
Answer for screen readers
| Student | $L_1$ | $L_2$ | $L_3$ | $L_4$ | $\bar{L}$ | $\sigma_{n-1}$ | $\alpha$ |
|---|---|---|---|---|---|---|---|
| Bassey | 1.4717 | 1.4711 | 1.4722 | 1.4715 | 1.471625 | 0.00029054 | 0.00014527 |
| Okon | 1.4753 | 1.4759 | 1.4756 | 1.4749 | 1.475425 | 0.000173206 | 0.000086603 |
| Effiong | 1.4719 | 1.4723 | 1.4727 | 1.4705 | 1.47185 | 0.000694442 | 0.000347221 |
Steps to Solve
- Calculate the Mean ($\bar{L}$)
For each student, the mean length measurement can be calculated using the formula:
$$ \bar{L} = \frac{L_1 + L_2 + L_3 + L_4}{n} $$
where $n$ is the number of measurements (which is 4 for each student).
For each student:
-
Bassey: $$ \bar{L} = \frac{1.4717 + 1.4711 + 1.4722 + 1.4715}{4} = \frac{5.8865}{4} = 1.471625 $$
-
Okon: $$ \bar{L} = \frac{1.4753 + 1.4759 + 1.4756 + 1.4749}{4} = \frac{5.9017}{4} = 1.475425 $$
-
Effiong: $$ \bar{L} = \frac{1.4719 + 1.4723 + 1.4727 + 1.4705}{4} = \frac{5.8874}{4} = 1.47185 $$
- Calculate the Standard Deviation ($\sigma_{n - 1}$)
The sample standard deviation can be calculated using the formula:
$$ \sigma_{n - 1} = \sqrt{\frac{\sum (L_i - \bar{L})^2}{n - 1}} $$
For each student:
-
Bassey: $$ \sigma_{n - 1} = \sqrt{\frac{(1.4717 - 1.471625)^2 + (1.4711 - 1.471625)^2 + (1.4722 - 1.471625)^2 + (1.4715 - 1.471625)^2}{3}} $$
-
Okon: $$ \sigma_{n - 1} = \sqrt{\frac{(1.4753 - 1.475425)^2 + (1.4759 - 1.475425)^2 + (1.4756 - 1.475425)^2 + (1.4749 - 1.475425)^2}{3}} $$
-
Effiong: $$ \sigma_{n - 1} = \sqrt{\frac{(1.4719 - 1.47185)^2 + (1.4723 - 1.47185)^2 + (1.4727 - 1.47185)^2 + (1.4705 - 1.47185)^2}{3}} $$
- Calculate the Standard Error ($\alpha$)
The standard error is calculated using:
$$ \alpha = \frac{\sigma_{n - 1}}{\sqrt{n}} $$
For each student, with $n = 4$.
- Fill in the Table
Complete the table based on the calculated values from steps 1, 2, and 3.
| Student | $L_1$ | $L_2$ | $L_3$ | $L_4$ | $\bar{L}$ | $\sigma_{n-1}$ | $\alpha$ |
|---|---|---|---|---|---|---|---|
| Bassey | 1.4717 | 1.4711 | 1.4722 | 1.4715 | 1.471625 | 0.00029054 | 0.00014527 |
| Okon | 1.4753 | 1.4759 | 1.4756 | 1.4749 | 1.475425 | 0.000173206 | 0.000086603 |
| Effiong | 1.4719 | 1.4723 | 1.4727 | 1.4705 | 1.47185 | 0.000694442 | 0.000347221 |
More Information
In this task, we've calculated the mean, standard deviation, and standard error for the measurements made by three students. The results depict how closely the measurements relate to each other. The table indicates that the precision of measurements varies among students.
Tips
- Forgetting to use $n - 1$ in the standard deviation formula.
- Miscalculating the mean by incorrectly adding all measurements.
- Not placing results in the correct columns when filling the table.
AI-generated content may contain errors. Please verify critical information