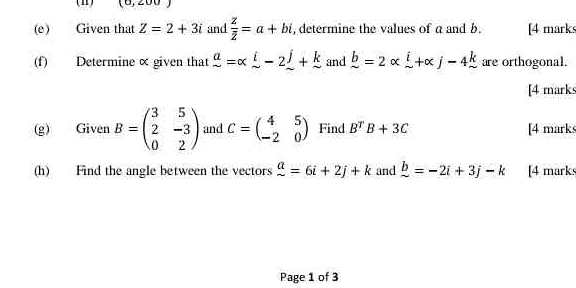
Given that Z = 2 + 3i and z̅ = a + bi, determine the values of a and b. Determine α given that α = α(–2i) + k and b = 2α ⊥ α, j - 4k are orthogonal. Given B = (3 5 2 0 -3 0) and C... Given that Z = 2 + 3i and z̅ = a + bi, determine the values of a and b. Determine α given that α = α(–2i) + k and b = 2α ⊥ α, j - 4k are orthogonal. Given B = (3 5 2 0 -3 0) and C = (4 5 -2), find B^TB + 3C. Find the angle between the vectors α = 6i + 2j + k and b = -2i + 3j - k. Read more

Steps to Solve

1. Determine values of ( a ) and ( b )

Given ( Z = 2 + 3i ) and ( \bar{Z} = a + bi ), we know that (\bar{Z}) is the conjugate of ( Z ). Therefore, ( a = 2 ) and ( b = -3 ) since the conjugate changes the sign of the imaginary part.

2. Determine ( \alpha )

Given ( \alpha = \alpha_1 \hat{i} + k ) and ( \beta = 2 \alpha_1 \hat{j} - 4 \hat{k} ), and that ( \alpha \perp \beta ), we use the fact that the dot product must equal zero: [ \alpha \cdot \beta = 0 ] This gives [ \alpha_1(0) + (1)(2\alpha_1) + (0)(-4) = 2\alpha_1 = 0 ] Hence, ( \alpha_1 = 0 ), leading to ( \alpha = k ).

3. Calculate ( B^T B + 3 C )

Using matrices ( B = \begin{pmatrix} 3 & 2 & 5 \ 0 & -3 & 2 \end{pmatrix} ) and ( C = \begin{pmatrix} 4 & -2 & 5 \ 0 & 2 \end{pmatrix} ).

First, find ( B^T ): [ B^T = \begin{pmatrix} 3 & 0 \ 2 & -3 \ 5 & 2 \end{pmatrix} ] Next calculate ( B^T B ): [ B^T B = \begin{pmatrix} 3 & 0 \ 2 & -3 \ 5 & 2 \end{pmatrix} \begin{pmatrix} 3 & 2 & 5 \ 0 & -3 & 2 \end{pmatrix} ] Perform the matrix multiplication: [ = \begin{pmatrix} 9 & 6 & 15 \ 0 & -6 & -6 \ 25 & 6 & 35 \end{pmatrix} ] Now compute ( 3C ): [ 3C = 3 \begin{pmatrix} 4 & -2 & 5 \ 0 & 2 \end{pmatrix} = \begin{pmatrix} 12 & -6 & 15 \ 0 & 6 \end{pmatrix} ] Finally, add ( B^T B + 3C ).

4. Find the angle between vectors ( \alpha ) and ( \beta )

Given vectors ( \alpha = 6\hat{i} + 2\hat{j} + \hat{k} ) and ( \beta = -2\hat{i} + 3\hat{j} - \hat{k} ).

Use the cosine formula: [ \cos(\theta) = \frac{\alpha \cdot \beta}{|\alpha||\beta|} ] Calculate the dot product ( \alpha \cdot \beta ): [ = (6)(-2) + (2)(3) + (1)(-1) = -12 + 6 - 1 = -7 ] Calculate the magnitudes: [ |\alpha| = \sqrt{6^2 + 2^2 + 1^2} = \sqrt{36 + 4 + 1} = \sqrt{41} ] [ |\beta| = \sqrt{(-2)^2 + 3^2 + (-1)^2} = \sqrt{4 + 9 + 1} = \sqrt{14} ] Compute ( \cos(\theta) ): [ \cos(\theta) = \frac{-7}{\sqrt{41} \cdot \sqrt{14}} ] Then find ( \theta ) using ( \theta = \cos^{-1}(-7/\sqrt{574}) ).