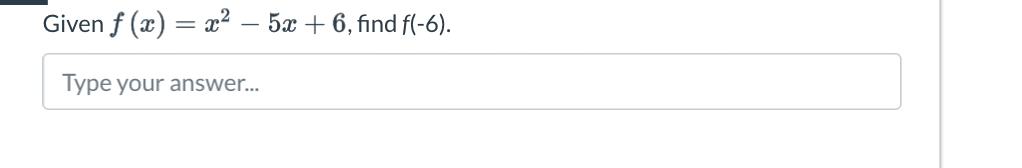
Given f(x) = x² - 5x + 6, find f(-6).

Understand the Problem

The question is asking to evaluate the function f(x) = x² - 5x + 6 at the point x = -6. We will substitute -6 into the function and simplify to find the value of f(-6).

Answer

The value of $ f(-6) $ is 72.

Steps to Solve

Substitute the value into the function

To find ( f(-6) ), we need to substitute ( x = -6 ) into the function ( f(x) = x^2 - 5x + 6 ).

Calculate ( x^2 )

First, calculate ( (-6)^2 ):

$$ (-6)^2 = 36 $$

Calculate ( -5x )

Next, calculate ( -5 \times -6 ):

$$ -5 \times -6 = 30 $$

Combine all parts of the equation

Now, combine the results from the previous steps with the constant term:

$$ f(-6) = 36 + 30 + 6 $$

Simplify the expression

Now, add the terms together:

$$ f(-6) = 36 + 30 + 6 = 72 $$

The value of ( f(-6) ) is 72.

More Information

The quadratic function ( f(x) = x^2 - 5x + 6 ) represents a parabola that opens upwards. Evaluating the function at different points helps us understand its behavior, and substituting negative values can lead to interesting results.

Tips

Forgetting to square the negative number. It's important to remember that ( (-6)^2 ) equals ( 36 ), not (-36).
Miscalculating ( -5 \times -6 ). Be careful with the signs; multiplying two negative numbers results in a positive product.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!