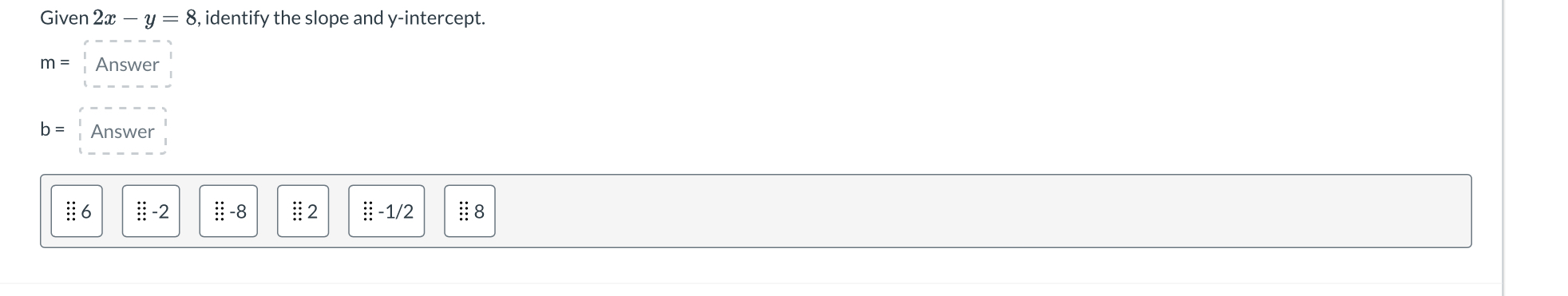
Given 2x - y = 8, identify the slope and y-intercept.
Understand the Problem
The question is asking to identify the slope (m) and y-intercept (b) of the linear equation given in standard form. We need to manipulate the equation into slope-intercept form (y = mx + b) to extract these values.
Answer
m = 2, b = -8
Answer for screen readers
m = 2
b = -8
Steps to Solve
- Rearranging the equation
Start with the given equation in standard form: $$ 2x - y = 8 $$
Next, isolate $y$ on one side of the equation. This can be done by subtracting $2x$ from both sides:
$$ -y = -2x + 8 $$
- Multiplying to eliminate negative sign
To get $y$ by itself, multiply both sides by $-1$:
$$ y = 2x - 8 $$
- Identifying slope and y-intercept
Now that the equation is in slope-intercept form ($y = mx + b$), we can identify the slope ($m$) and the y-intercept ($b$):
- The slope $m = 2$ (the coefficient of $x$)
- The y-intercept $b = -8$
m = 2
b = -8
More Information
The slope ($m$) indicates that for each increase of 1 in $x$, $y$ increases by 2. The y-intercept ($b$) tells us that the line crosses the y-axis at -8.
Tips
- Not changing the signs when isolating $y$. Remember to multiply through by -1.
- Confusing the slope and y-intercept in the final equation. Always check coefficients and constant terms.