Find the value of x.
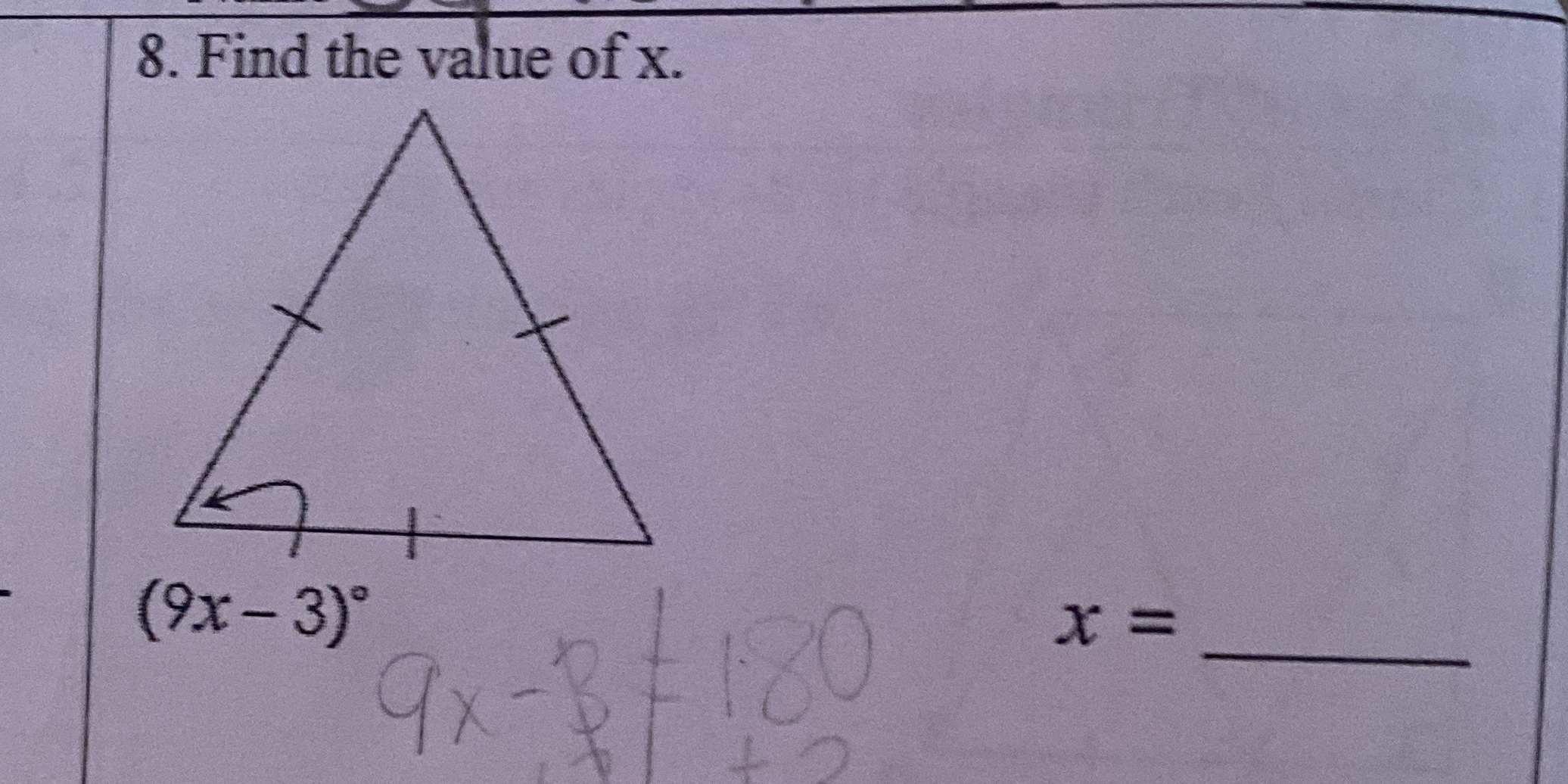
Understand the Problem
The question is asking to find the value of 'x' in a triangle based on the given angle expression (9x - 3) degrees and its relationship to the triangle's angles. We will use the property that the sum of angles in a triangle is 180 degrees to solve for 'x'.
Answer
$x = 7$
Answer for screen readers
The value of $x$ is $7$.
Steps to Solve
- Identify angle relationships in the triangle
The angle given is $(9x - 3)^\circ$. The other two angles in the triangle must also be defined. Since the triangle has two equal sides, it is an isosceles triangle, meaning the other two angles are equal.
- Set up the equation based on the triangle's angle sum property
We know that the sum of the angles in a triangle must equal $180^\circ$. Therefore, if we let each of the two equal angles be represented as $y$, we can write the equation: $$ (9x - 3) + y + y = 180 $$
This can be simplified to: $$ (9x - 3) + 2y = 180 $$
- Solve for $y$ in terms of $x$
Rearranging the equation gives us: $$ 2y = 180 - (9x - 3) $$
This simplifies to: $$ 2y = 183 - 9x $$ $$ y = \frac{183 - 9x}{2} $$
- Use the property of isosceles triangles
Since the triangle is isosceles, we can equate the angle $(9x - 3)^\circ$ to the angle $y$: $$ 9x - 3 = \frac{183 - 9x}{2} $$
- Eliminate the fraction
To eliminate the fraction, multiply both sides by 2: $$ 2(9x - 3) = 183 - 9x $$
Distributing gives us: $$ 18x - 6 = 183 - 9x $$
- Combine like terms
Adding $9x$ to both sides and $6$ to both sides gives: $$ 27x = 189 $$
- Solve for $x$
Dividing both sides by 27: $$ x = \frac{189}{27} = 7 $$
The value of $x$ is $7$.
More Information
In an isosceles triangle, two angles are equal, which was critical in setting up the equation. The angle measures rely on understanding the sum of angles in a triangle and leveraging symmetry for isosceles triangles.
Tips
- Assuming all triangle angles can be different without acknowledging the isosceles property.
- Not simplifying equations properly leading to calculation errors.
- Forgetting to check if $x$ falls within a reasonable range after solving.
AI-generated content may contain errors. Please verify critical information