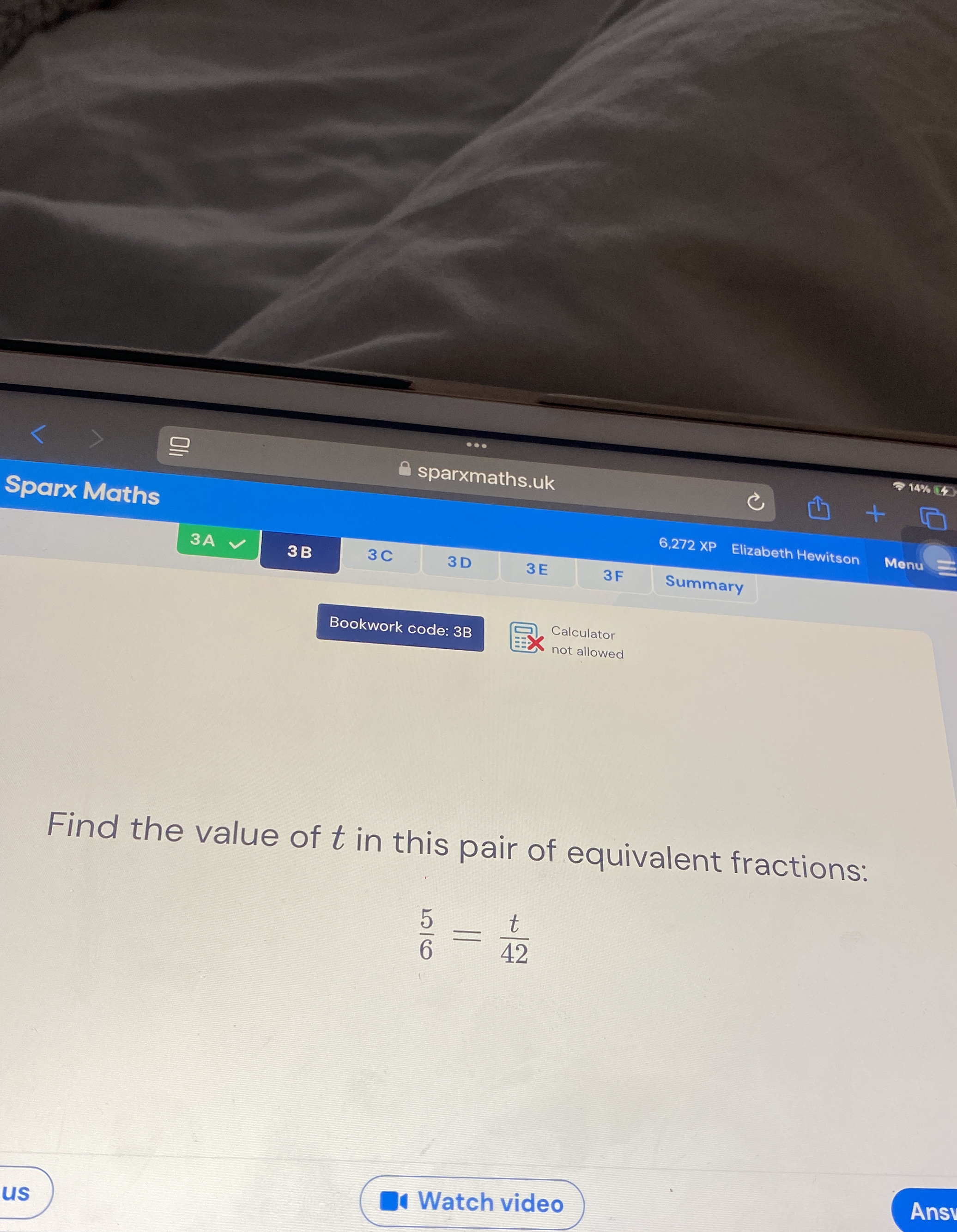
Find the value of t in this pair of equivalent fractions: 5/6 = t/42.

Understand the Problem

The question is asking to find the value of 't' in the equivalent fractions 5/6 and t/42. This involves setting up a proportion and solving for 't'.

Answer

The value of $ t $ is $ 35 $.

Steps to Solve

Set up the proportion

We are given the fractions ( \frac{5}{6} ) and ( \frac{t}{42} ). We can set up the equation as:

$$ \frac{5}{6} = \frac{t}{42} $$

Cross-multiply

To eliminate the fractions, we cross-multiply:

$$ 5 \cdot 42 = 6 \cdot t $$

This results in:

$$ 210 = 6t $$

Isolate 't'

To solve for ( t ), divide both sides by 6:

$$ t = \frac{210}{6} $$

Calculate the value of 't'

Now perform the division:

$$ t = 35 $$

The value of ( t ) is ( 35 ).

More Information

When comparing equivalent fractions, cross-multiplying helps to maintain equality. This method can be useful for solving problems with proportions in various contexts.

Tips

Incorrect cross-multiplication: Ensure both sides of the equation are multiplied correctly.
Not simplifying: When dividing, make sure to simplify the result properly.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!