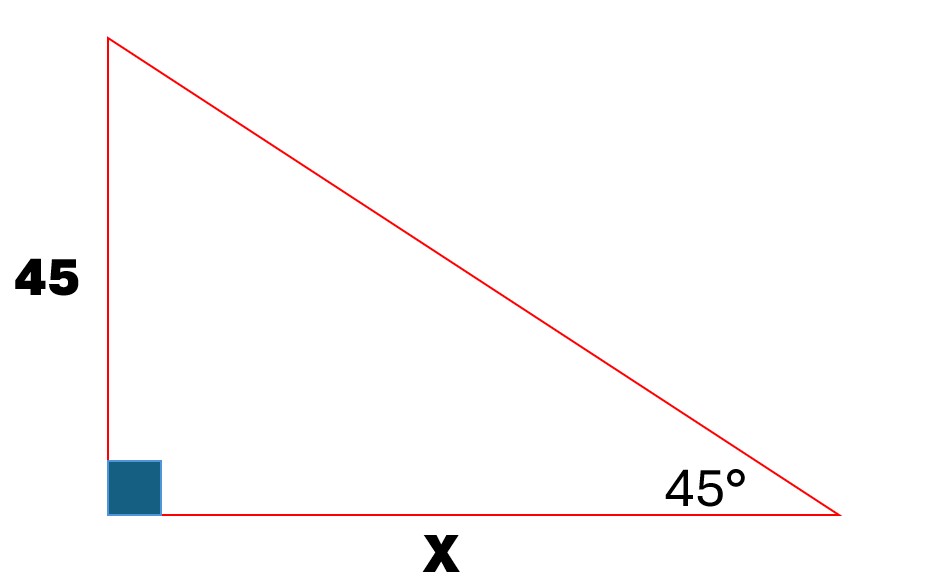
Find the length of side x in a right triangle with one leg of length 45 and an angle of 45 degrees.
Understand the Problem
The question is asking to find the length of side 'x' in a right triangle where one angle is 45 degrees, and the other leg has a length of 45. Since it is a 45-45-90 triangle, the lengths of the two legs are equal.
Answer
The length of side $x$ is $45$.
Answer for screen readers
The length of side 'x' is $45$.
Steps to Solve
- Identify the properties of a 45-45-90 triangle
In a 45-45-90 triangle, the legs are of equal length. Thus, if one leg measures 45, the other leg (which is side 'x') is also 45.
- State the relationship between the legs
In a 45-45-90 triangle, both legs have the same length. Therefore, we can express the length of side 'x' as: $$ x = 45 $$
- Write the final expression
Since both legs are equal in length, we conclude that: $$ x = 45 $$
The length of side 'x' is $45$.
More Information
In a 45-45-90 triangle, both legs are equal and the length of each leg can be derived from the hypotenuse, which is $x\sqrt{2}$ if known. If both legs are given as equal lengths, they can directly indicate each other's measurements.
Tips
- Assuming the lengths of the sides are different without recognizing the properties of the 45-45-90 triangle. To avoid this, always recall that in a 45-45-90 triangle, the legs must be equal.