Find the derivative of y with respect to x. y = 7 ln(cos x)
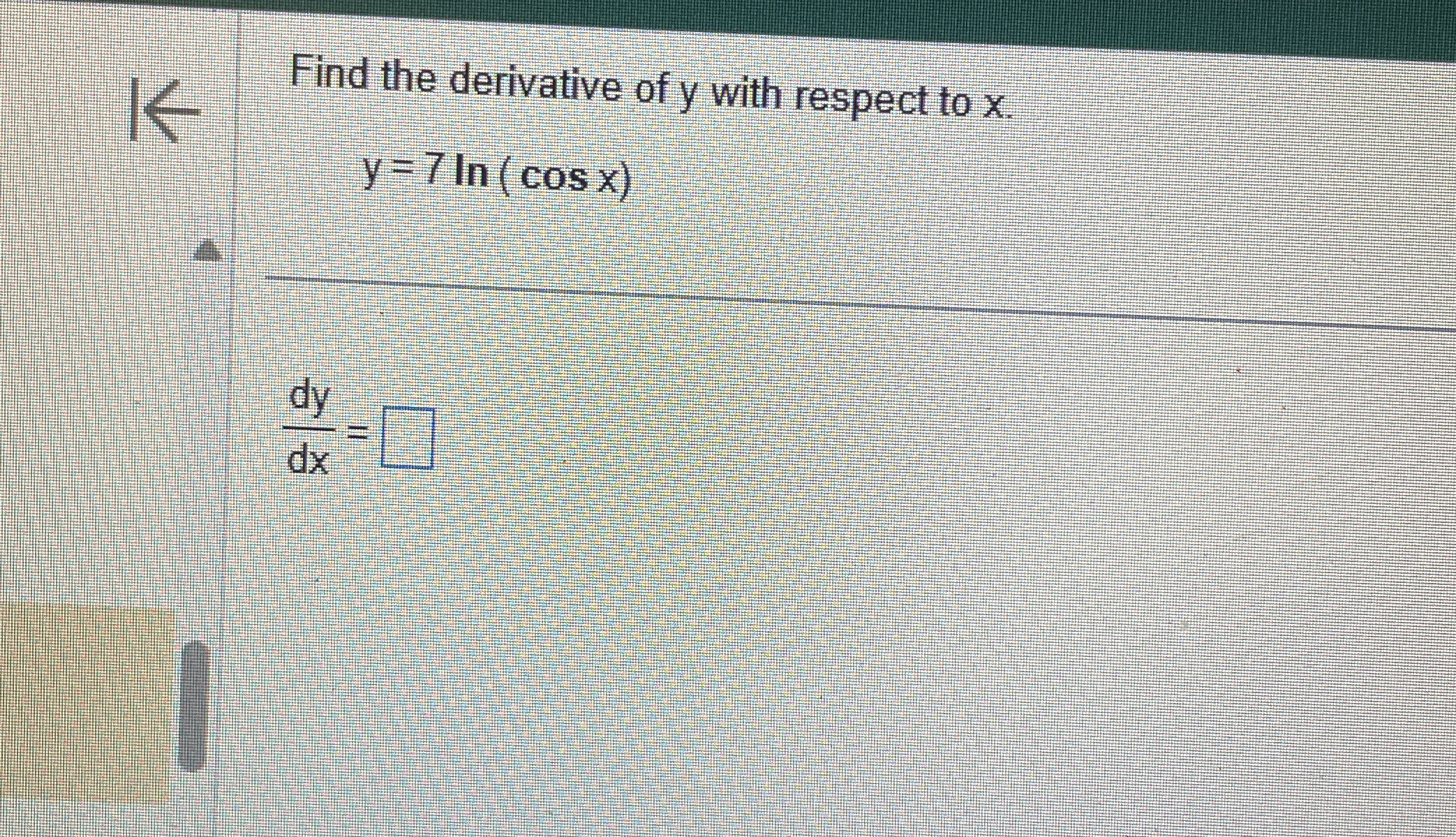
Understand the Problem
The question is asking to find the derivative of the function y = 7 ln(cos x) with respect to x, which involves applying the rules of differentiation.
Answer
$$ \frac{dy}{dx} = -7 \tan x $$
Answer for screen readers
The derivative of $y$ with respect to $x$ is $$ \frac{dy}{dx} = -7 \tan x $$
Steps to Solve
- Identify the function and apply the chain rule
The function is $y = 7 \ln(\cos x)$. To find the derivative, we’ll use the chain rule. The derivative of $\ln(u)$ is $\frac{1}{u} \frac{du}{dx}$ where $u = \cos x$.
- Differentiate the outer function
Differentiate $y$ with respect to $\cos x$: $$ \frac{dy}{du} = 7 \cdot \frac{1}{\cos x} $$
- Differentiate the inner function
Now, differentiate $u = \cos x$ with respect to $x$: $$ \frac{du}{dx} = -\sin x $$
- Combine the derivatives using the chain rule
Now use the chain rule to find $\frac{dy}{dx}$: $$ \frac{dy}{dx} = \frac{dy}{du} \cdot \frac{du}{dx} = 7 \cdot \frac{1}{\cos x} \cdot (-\sin x) $$
- Simplify the expression
Combine and simplify: $$ \frac{dy}{dx} = -7 \frac{\sin x}{\cos x} = -7 \tan x $$
The derivative of $y$ with respect to $x$ is $$ \frac{dy}{dx} = -7 \tan x $$
More Information
This derivative indicates the rate of change of the function $y$ with respect to $x$. The tangent function, $\tan x$, is commonly encountered in trigonometry and calculus, reflecting the slope of the tangent line at any point on the curve defined by $y$.
Tips
Common mistakes when finding this derivative include:
- Forgetting to apply the chain rule correctly.
- Neglecting to differentiate the inner function ($\cos x$).
- Confusing the signs when using the derivative of trigonometric functions.
AI-generated content may contain errors. Please verify critical information