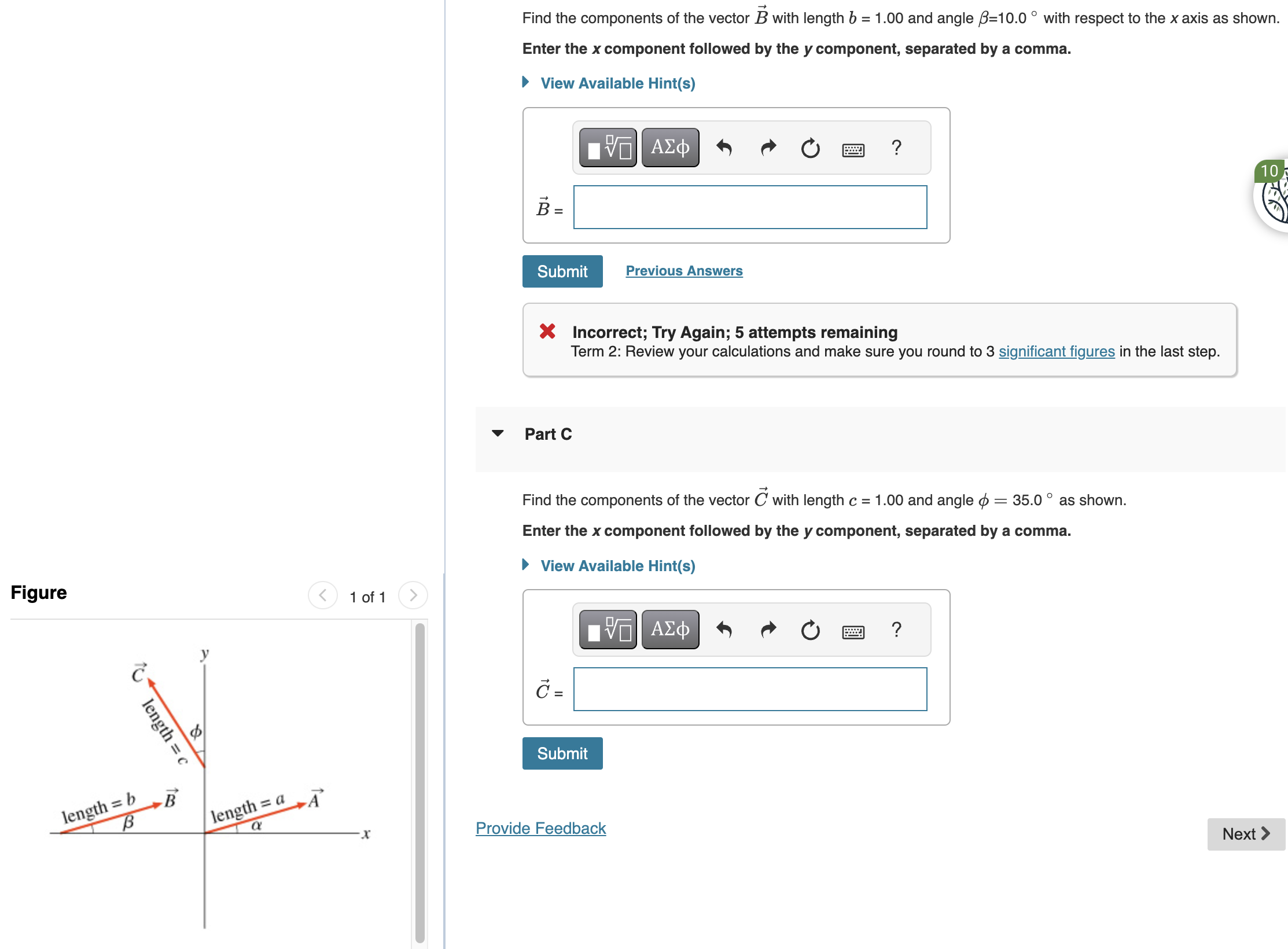
Find the components of the vector B with length b = 1.00 and angle β = 10.0° with respect to the x axis. Then, find the components of the vector C with length c = 1.00 and angle φ... Find the components of the vector B with length b = 1.00 and angle β = 10.0° with respect to the x axis. Then, find the components of the vector C with length c = 1.00 and angle φ = 35.0° as shown. Enter the x component followed by the y component, separated by a comma.
Understand the Problem
The question is asking to find the x and y components of two vectors based on their lengths and angles with respect to the x-axis. The calculation involves using trigonometric functions to resolve the components of the vectors.
Answer
For vector \( \vec{B} \): \( 0.985, 0.174 \); For vector \( \vec{C} \): \( 0.819, 0.573 \).
Answer for screen readers
The components of vector ( \vec{B} ) are approximately ( 0.985, 0.174 ) and for vector ( \vec{C} ), ( 0.819, 0.573 ).
Steps to Solve
- Identify the given values for vector ( \vec{B} )
The length of vector ( \vec{B} ) is ( b = 1.00 ) and the angle ( \beta = 10.0^\circ ).
- Calculate the x-component of vector ( \vec{B} )
The x-component can be found using the cosine function:
$$ B_x = b \cdot \cos(\beta) $$
Substituting the values:
$$ B_x = 1.00 \cdot \cos(10.0^\circ) $$
- Calculate the y-component of vector ( \vec{B} )
The y-component can be found using the sine function:
$$ B_y = b \cdot \sin(\beta) $$
Substituting the values:
$$ B_y = 1.00 \cdot \sin(10.0^\circ) $$
- Present the components of vector ( \vec{B} )
Round the results to three significant figures and enter the x and y components separated by a comma.
- Identify the given values for vector ( \vec{C} )
The length of vector ( \vec{C} ) is ( c = 1.00 ) and the angle ( \phi = 35.0^\circ ).
- Calculate the x-component of vector ( \vec{C} )
The x-component can be calculated using:
$$ C_x = c \cdot \cos(\phi) $$
Substituting the values:
$$ C_x = 1.00 \cdot \cos(35.0^\circ) $$
- Calculate the y-component of vector ( \vec{C} )
The y-component is given by:
$$ C_y = c \cdot \sin(\phi) $$
Substituting the values:
$$ C_y = 1.00 \cdot \sin(35.0^\circ) $$
- Present the components of vector ( \vec{C} )
Round the results to three significant figures and enter the x and y components separated by a comma.
The components of vector ( \vec{B} ) are approximately ( 0.985, 0.174 ) and for vector ( \vec{C} ), ( 0.819, 0.573 ).
More Information
Vector components are essential in physics and engineering as they allow us to analyze forces and motions in a two-dimensional plane. Using trigonometric functions helps to break down vectors into their horizontal and vertical parts.
Tips
- Not using degrees or radians correctly: Ensure that your calculator is set to the correct mode (degree for this problem).
- Rounding too early: It's important to maintain full precision in calculations and apply rounding only in the final answer.
AI-generated content may contain errors. Please verify critical information