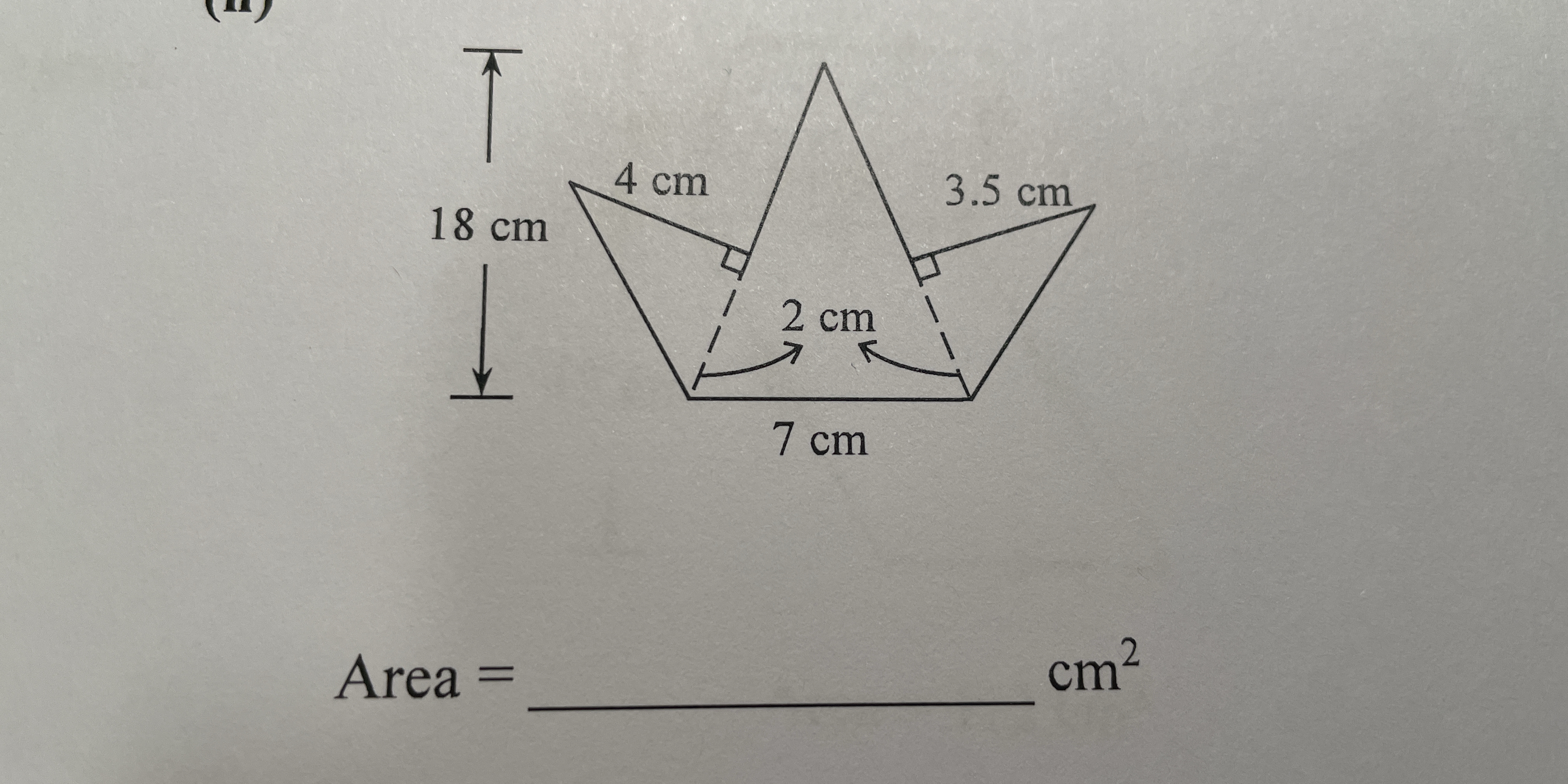
Calculate the area of the shape shown in the image.

Steps to Solve

1. Calculate the area of the trapezoid

The trapezoid has a height of (18 , \text{cm}) and bases of (7 , \text{cm}) and (2 + 2 = 4 , \text{cm}).

Using the trapezoidal area formula:

$$ A = \frac{1}{2} \times (b_1 + b_2) \times h $$

Where (b_1 = 7 , \text{cm}), (b_2 = 4 , \text{cm}), and (h = 18 , \text{cm}).

$$ A = \frac{1}{2} \times (7 , \text{cm} + 4 , \text{cm}) \times 18 , \text{cm} $$ $$ A = \frac{1}{2} \times 11 , \text{cm} \times 18 , \text{cm} $$ $$ A = 99 , \text{cm}^2 $$

2. Calculate the area of the triangles

There are two triangles, each with a base of (2 , \text{cm}) and heights of (4 , \text{cm}) and (3.5 , \text{cm}) respectively.

For the first triangle:

$$ A_1 = \frac{1}{2} \times \text{base} \times \text{height} = \frac{1}{2} \times 2 , \text{cm} \times 4 , \text{cm} = 4 , \text{cm}^2 $$

For the second triangle:

$$ A_2 = \frac{1}{2} \times 2 , \text{cm} \times 3.5 , \text{cm} = 3.5 , \text{cm}^2 $$

3. Add the areas

Now, add the area of the trapezoid and the areas of both triangles together:

$$ \text{Total Area} = A + A_1 + A_2 $$ $$ \text{Total Area} = 99 , \text{cm}^2 + 4 , \text{cm}^2 + 3.5 , \text{cm}^2 $$ $$ \text{Total Area} = 106.5 , \text{cm}^2 $$