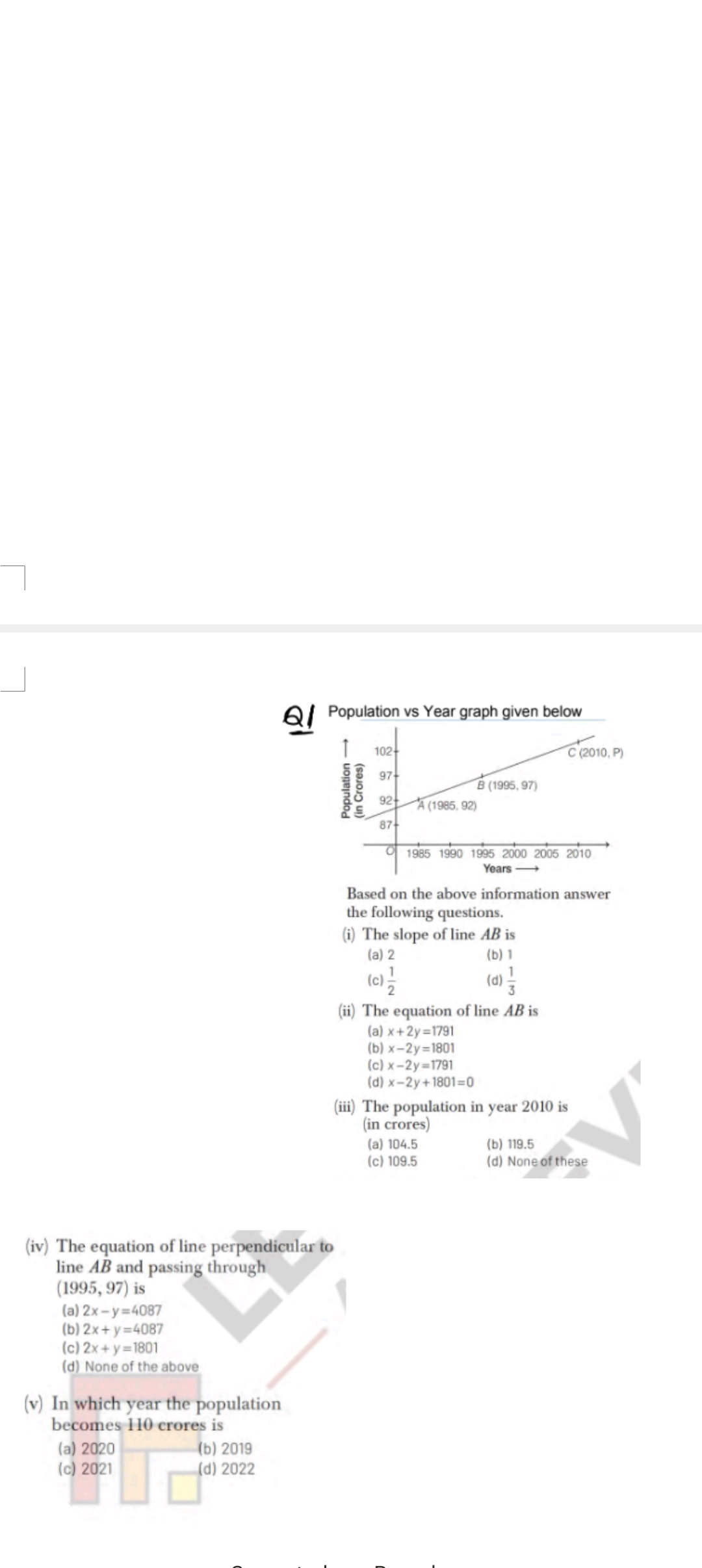
Based on the above information answer the following questions. (i) The slope of line AB is? (ii) The equation of line AB is? (iii) The population in year 2010 is (in crores)? (iv)... Based on the above information answer the following questions. (i) The slope of line AB is? (ii) The equation of line AB is? (iii) The population in year 2010 is (in crores)? (iv) The equation of line perpendicular to line AB and passing through (1995, 97) is? (v) In which year the population becomes 110 crores? Read more

Steps to Solve

1. Identifying Points on the Graph

From the graph, we have two points:

• Point A (1985, 92)
• Point B (1995, 97)

These points will be used to find the slope and the equation of the line.

2. Calculating the Slope of Line AB

The slope (m) of a line through two points $(x_1, y_1)$ and $(x_2, y_2)$ is given by the formula:

$$ m = \frac{y_2 - y_1}{x_2 - x_1} $$

Substituting the values for points A and B:

$$ m = \frac{97 - 92}{1995 - 1985} = \frac{5}{10} = \frac{1}{2} $$

3. Finding the Equation of the Line AB

Using the slope-intercept form $y = mx + b$, we need to find the y-intercept (b). We can use point A (1985, 92) to find b.

Rearranging the equation gives us:

$$ b = y - mx $$

Substituting known values:

$$ b = 92 - \frac{1}{2} \cdot 1985 $$

To find the exact value, we also convert it to standard form; we simplify further to get:

Since slope $m = \frac{1}{2}$,

The equation in standard form can be derived as:

$$ x - 2y + 1801 = 0 $$

4. Finding Population in Year 2010

The population for the year 2010 can be estimated using the slope-intercept form:

Using the calculated slope and equation, substitute ( x = 2010 ):

$$ y = \frac{1}{2} \times 2010 + b $$

Calculate ( b ) using one of the previous equations or point:

For example,

Using point B (1995, 97) gives us:

$$ y = \frac{1}{2} \cdot 2010 + b $$

Calculate the equation of line at 2010.

5. Finding Perpendicular Line

To find the equation of the line that is perpendicular to line AB and passing through (1995, 97):

The slope of the perpendicular line is the negative reciprocal of ( \frac{1}{2} ), which is -2.

Using point-slope form:

$$ y - y_1 = m (x - x_1) $$

Substituting the point (1995, 97):

$$ y - 97 = -2 (x - 1995) $$

Rearranging to standard form gives:

$$ 2x + y - 4087 = 0 $$

6. Finding Year for Population of 110 Crores

To find when the population becomes 110 crores, we set up the equation:

$$ 110 = \frac{1}{2}x + b $$

Solving this around the estimated year using derived linear function.