Understand the Problem
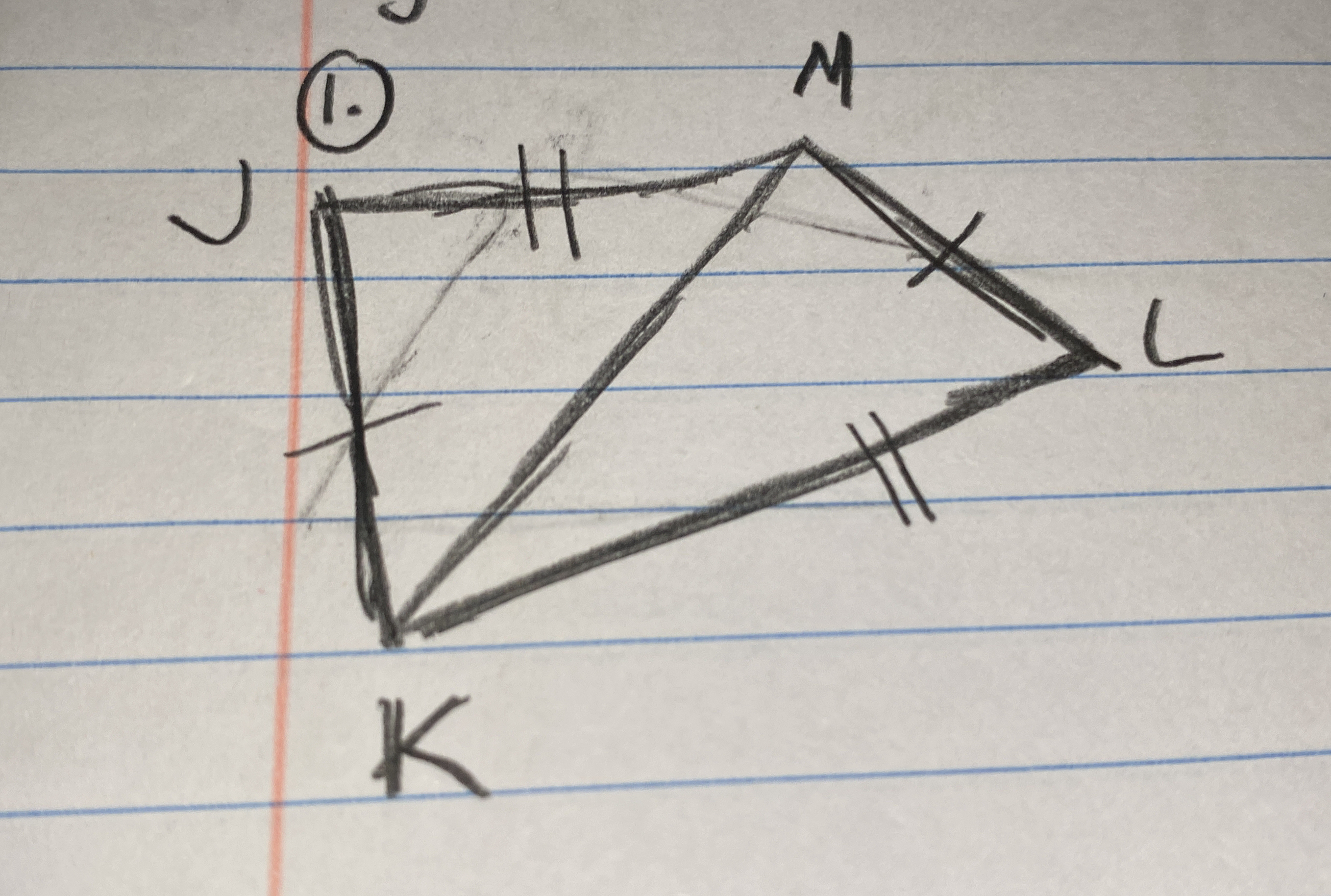
The question involves a geometric figure with points labeled and lines indicating equal lengths. The problem likely requires an analysis of the properties or relationships within the shape, which appears to be a quadrilateral based on the sketch.
Answer
The quadrilateral $JKLM$ is a parallelogram.
Answer for screen readers
The quadrilateral $JKLM$ is a parallelogram.
Steps to Solve
-
Identify the shapes and properties Examine the quadrilateral formed by points $J$, $K$, $L$, $M$, and $X$. Determine any properties based on the equal length markings.
-
Check for congruent segments Since marking indicates equal lengths (the lines with hashes), identify pairs of segments. For instance, if $JK \cong LM$ and $JL \cong MK$, then this could imply specific relationships between the angles or the type of quadrilateral.
-
Use properties of quadrilaterals Recall that if two pairs of opposite sides are equal, then the quadrilateral could be a parallelogram. Therefore, examine if $JK \parallel LM$ and $JL \parallel MK$.
-
Determine the type of quadrilateral Based on the properties and relationships established, conclude whether the quadrilateral is a parallelogram, rectangle, rhombus, or square.
The quadrilateral $JKLM$ is a parallelogram.
More Information
In a parallelogram, the opposite sides are both equal and parallel. The given markings indicate that $JK \cong LM$ and $JL \cong MK$, satisfying the properties of a parallelogram. This information can be useful in further geometric analyses or problem-solving.
Tips
- Confusing segments as congruent when they aren't marked or indicated. Always check for appropriate markings before concluding equal segments.
- Assuming that all quadrilaterals are parallelograms without verifying that both pairs of opposite sides are equal. Always confirm by checking parallelism.
AI-generated content may contain errors. Please verify critical information