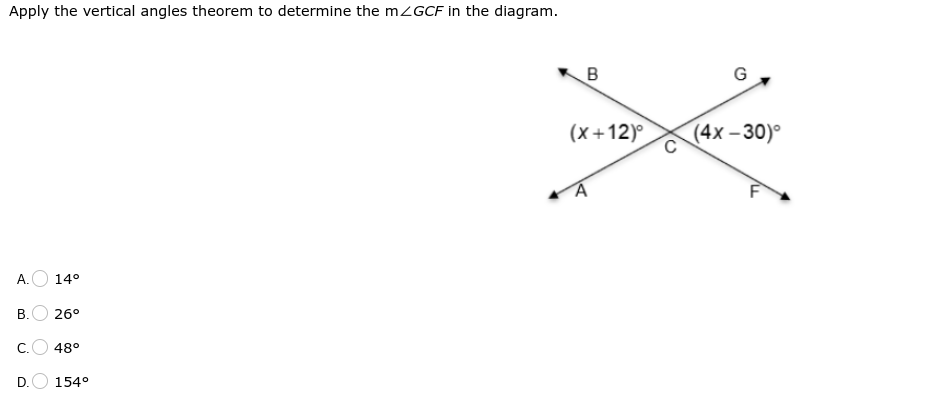
Apply the vertical angles theorem to determine the m∠GCF in the diagram.
Understand the Problem
The question is asking us to apply the vertical angles theorem to find the measure of angle GCF in the given diagram, where one angle is expressed as (x + 12)° and the other as (4x - 30)°, implying that these angles are equal because they are vertical angles. We will need to set up an equation and solve for x to find the measures of the angles.
Answer
$26°$
Answer for screen readers
The measure of angle GCF is $26°$.
Steps to Solve
- Set Up the Equation
Since vertical angles are equal, we can set the angles equal to each other: $$(x + 12) = (4x - 30)$$
- Rearrange the Equation
To isolate $x$, subtract $x$ from both sides: $$12 = 4x - x - 30$$ This simplifies to: $$12 = 3x - 30$$
- Add 30 to Both Sides
Next, add 30 to both sides to eliminate the constant on the right side: $$12 + 30 = 3x$$ This simplifies to: $$42 = 3x$$
- Solve for x
Now, divide both sides by 3 to find $x$: $$x = \frac{42}{3}$$ So, $$x = 14$$
- Find the Measure of Angle GCF
Now substitute $x$ back into the expression for angle GCF: $$m∠GCF = x + 12$$ This will be: $$m∠GCF = 14 + 12 = 26°$$
The measure of angle GCF is $26°$.
More Information
The vertical angles theorem states that angles opposite each other when two lines intersect are equal. Here, we found $x$ and then calculated the actual angle measure.
Tips
- Miscalculating when distributing terms or combining like terms.
- Forgetting to correctly interpret the vertical angles as equal, leading to setting up the wrong equation.
AI-generated content may contain errors. Please verify critical information