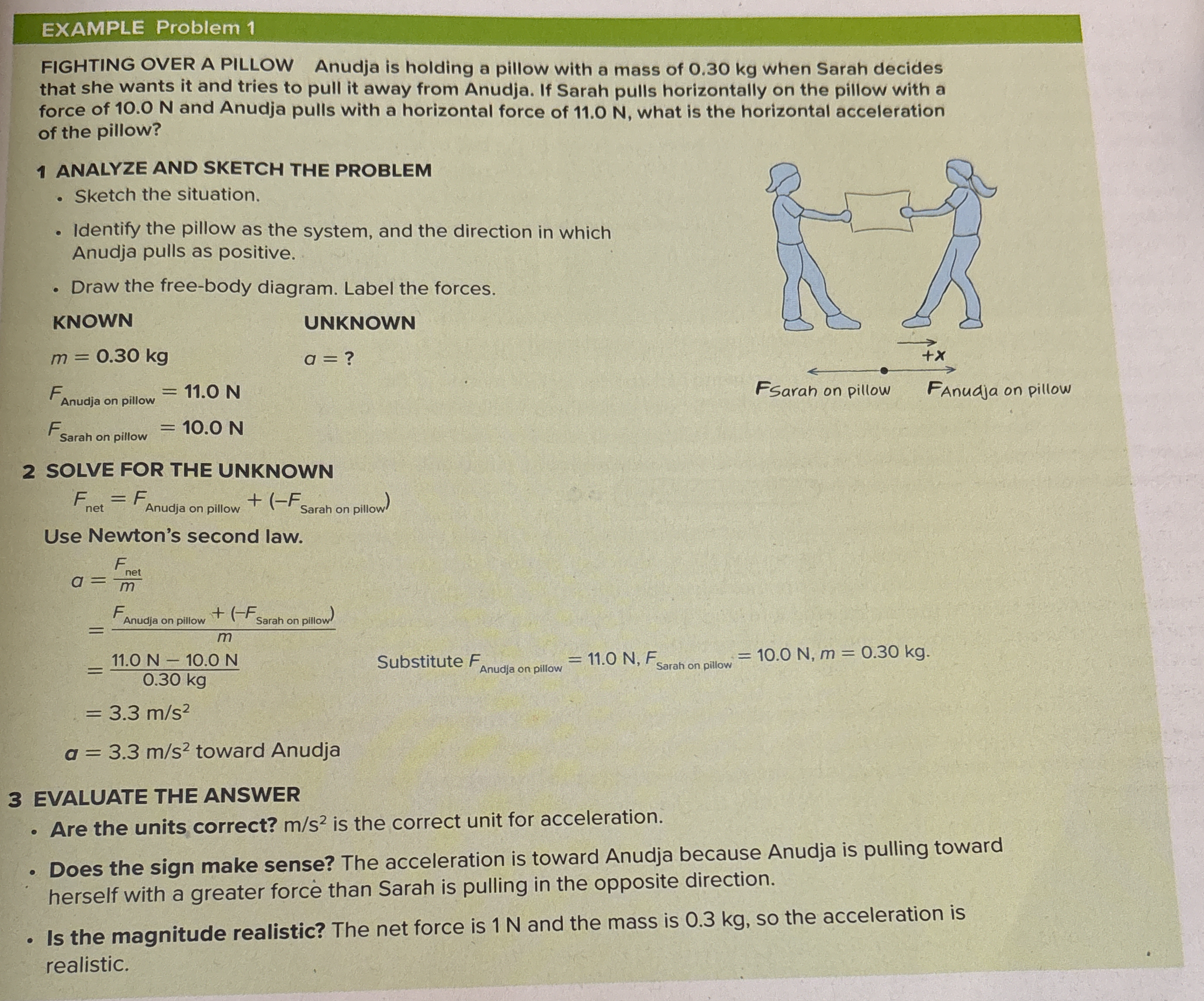
Anuja is holding a pillow with a mass of 0.30 kg. If Sarah pulls horizontally on the pillow with a force of 10.0 N and Anuja pulls with a force of 11.0 N, what is the horizontal ac... Anuja is holding a pillow with a mass of 0.30 kg. If Sarah pulls horizontally on the pillow with a force of 10.0 N and Anuja pulls with a force of 11.0 N, what is the horizontal acceleration of the pillow?
Understand the Problem
The question is asking to analyze a physics problem involving forces and acceleration acting on a pillow. It involves applying Newton's second law to solve for the horizontal acceleration of the pillow given the forces applied by two individuals.
Answer
The horizontal acceleration of the pillow is $a = 3.33 \text{ m/s}^2$.
Answer for screen readers
The horizontal acceleration of the pillow is $a = 3.33 \text{ m/s}^2$.
Steps to Solve
-
Identify known values We know the mass of the pillow $m = 0.30 \text{ kg}$, the force applied by Anudja $F_{Anudja \text{ on pillow}} = 11.0 \text{ N}$, and the force applied by Sarah $F_{Sarah \text{ on pillow}} = 10.0 \text{ N}$.
-
Calculate net force The net force acting on the pillow can be calculated using the equation: $$ F_{net} = F_{Anudja \text{ on pillow}} + \left(- F_{Sarah \text{ on pillow}}\right) $$ Substituting the known values: $$ F_{net} = 11.0 \text{ N} + \left(-10.0 \text{ N}\right) $$ This simplifies to: $$ F_{net} = 1.0 \text{ N} $$
-
Apply Newton's second law Using Newton's second law, we find the acceleration $a$: $$ a = \frac{F_{net}}{m} $$ Substituting the values we just calculated: $$ a = \frac{1.0 \text{ N}}{0.30 \text{ kg}} $$
-
Calculate the acceleration Now compute the value: $$ a = 3.33 \text{ m/s}^2 $$
The horizontal acceleration of the pillow is $a = 3.33 \text{ m/s}^2$.
More Information
In this scenario, the pillow accelerates toward Anudja at approximately $3.33 \text{ m/s}^2$. This means that Anudja is pulling the pillow toward herself with a greater force than Sarah is pulling it away.
Tips
- Not correctly determining the direction of forces. Always identify which direction is positive to avoid errors in summation.
- Confusing the unit conversion for mass and acceleration, ensure that mass is always in kilograms and acceleration in meters per second squared.
AI-generated content may contain errors. Please verify critical information