Analyze the system of equations represented in the graph and find the solution.

Understand the Problem

The question is likely asking to analyze or solve a system of linear equations graphically based on the provided graph. It involves understanding the intersection points of the lines represented and potentially determining solutions to the equations.

Answer

The solution is approximately $(3, 2)$.

Steps to Solve

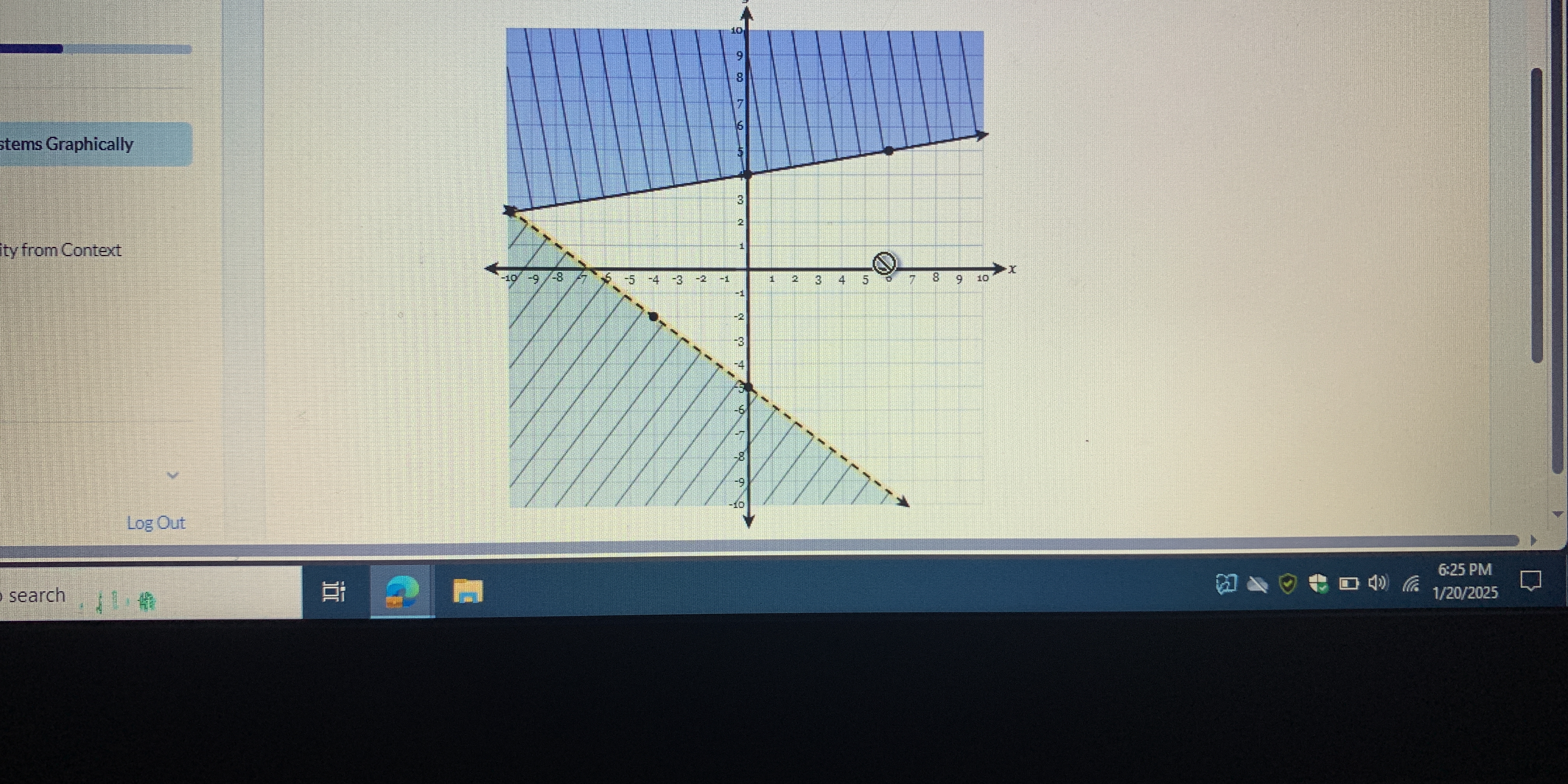
Identify the Lines Observe the two lines in the graph. One appears to be increasing (the black line) and the other decreasing (the orange line). Note their slopes and intercepts.
Determine the Intersection Point The solution to the system of equations formed by these lines is found at their intersection point. Look for the exact coordinates where the two lines cross each other.
Read Coordinates from the Graph Estimate the coordinates of the intersection point by analyzing the grid. You may find that the intersection occurs at approximately $(3, 2)$.
Verify the Solution Substitute the coordinates back into the equations of both lines to verify if they satisfy both equations.
Conclusion State that the intersection point is the solution to the system of equations represented by the two lines.

The solution to the system of equations, represented by the intersection point, is approximately $(3, 2)$.

More Information

The intersection point $(3, 2)$ means that both lines share this coordinate, confirming that this point is a solution to both linear equations. Graphically solving a system of equations helps visualize the relationships between functions.

Tips

Misreading the graph: Ensure to accurately identify the coordinates of the intersection point, as slight inaccuracies can lead to the wrong conclusion.
Forgetting to verify: Always substitute back into the original equations to confirm the intersection point is indeed a solution.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!