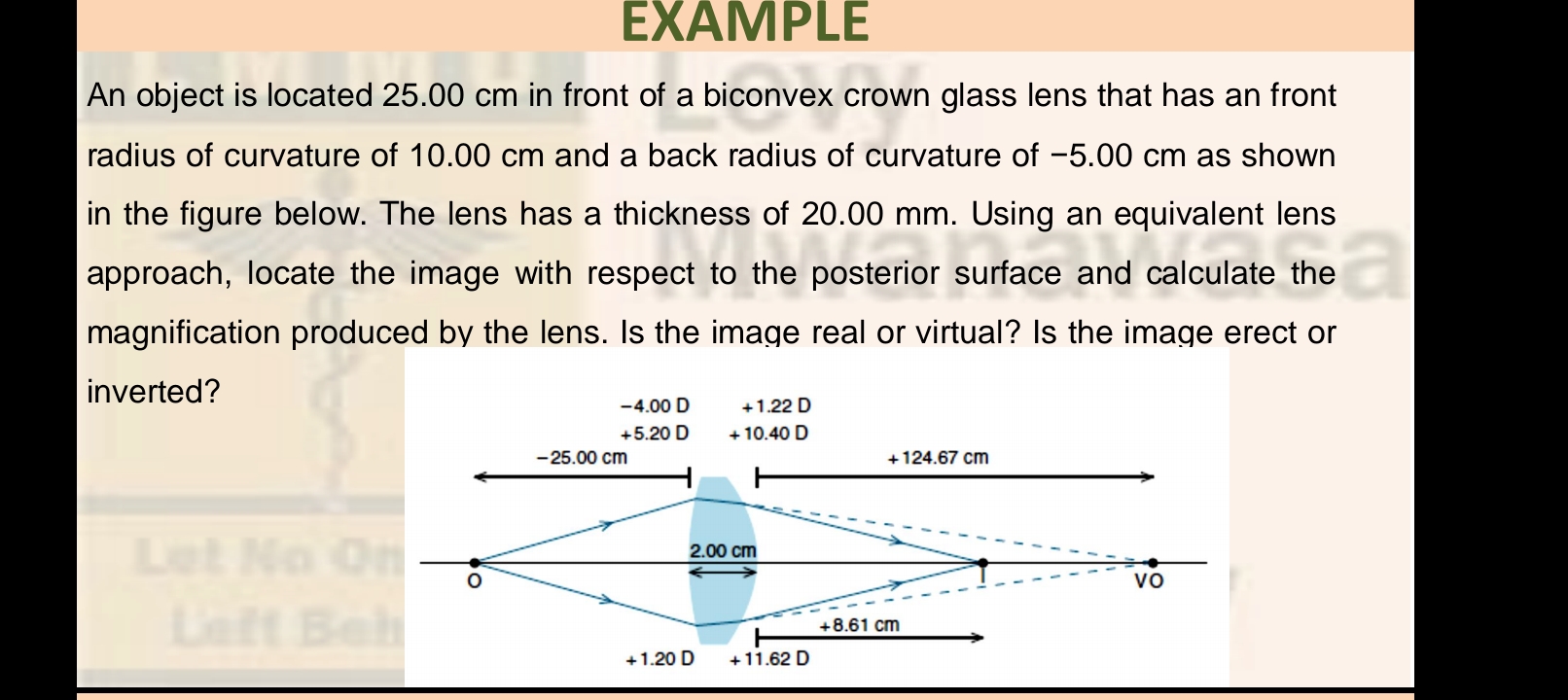
An object is located 25.00 cm in front of a biconvex crown glass lens that has an front radius of curvature of 10.00 cm and a back radius of curvature of -5.00 cm. The lens has a t... An object is located 25.00 cm in front of a biconvex crown glass lens that has an front radius of curvature of 10.00 cm and a back radius of curvature of -5.00 cm. The lens has a thickness of 20.00 mm. Using an equivalent lens approach, locate the image with respect to the posterior surface and calculate the magnification produced by the lens. Is the image real or virtual? Is the image erect or inverted? Read more

Steps to Solve

1. Calculate the focal length of the lens To find the focal length ($f$) of the biconvex lens, we can use the lens maker's formula: $$ \frac{1}{f} = (n - 1) \left( \frac{1}{R_1} - \frac{1}{R_2} \right) $$ where:

• $n$ is the refractive index (for crown glass, approximately $1.5$)
• $R_1$ is the radius of curvature of the first surface (positive for convex, so $R_1 = +10.00 , \text{cm}$)
• $R_2$ is the radius of curvature of the second surface (negative for concave, so $R_2 = -5.00 , \text{cm}$)

Substituting the values: $$ \frac{1}{f} = (1.5 - 1) \left( \frac{1}{10} - \frac{1}{-5} \right) $$ $$ \frac{1}{f} = 0.5 \left( 0.1 + 0.2 \right) = 0.5 \times 0.3 = 0.15 $$ Thus, we find: $$ f = \frac{1}{0.15} = 6.67 , \text{cm} $$

2. Use the thin lens formula Next, apply the thin lens equation to find the image distance ($v$): $$ \frac{1}{f} = \frac{1}{d_o} + \frac{1}{d_i} $$ where:

• $d_o = 25.00 , \text{cm}$ (object distance)
• $d_i$ is the image distance

Rearranging gives: $$ \frac{1}{d_i} = \frac{1}{f} - \frac{1}{d_o} $$ Plugging in values: $$ \frac{1}{d_i} = \frac{1}{6.67} - \frac{1}{25} $$

Calculating: $$ \frac{1}{d_i} = 0.15 - 0.04 = 0.11 $$ So, $$ d_i = \frac{1}{0.11} \approx 9.09 , \text{cm} $$

3. Determine the magnification The magnification ($m$) can be calculated using: $$ m = -\frac{d_i}{d_o} $$ Substituting the values: $$ m = -\frac{9.09}{25.00} \approx -0.36 $$

4. Identify the nature of the image From the calculations:

• Since $d_i$ is positive, the image is real and located on the opposite side of the lens from the object.
• The negative value of the magnification indicates that the image is inverted.