A sine function completes one cycle from x = 7π/4 to x = -5π/4. What is its period?

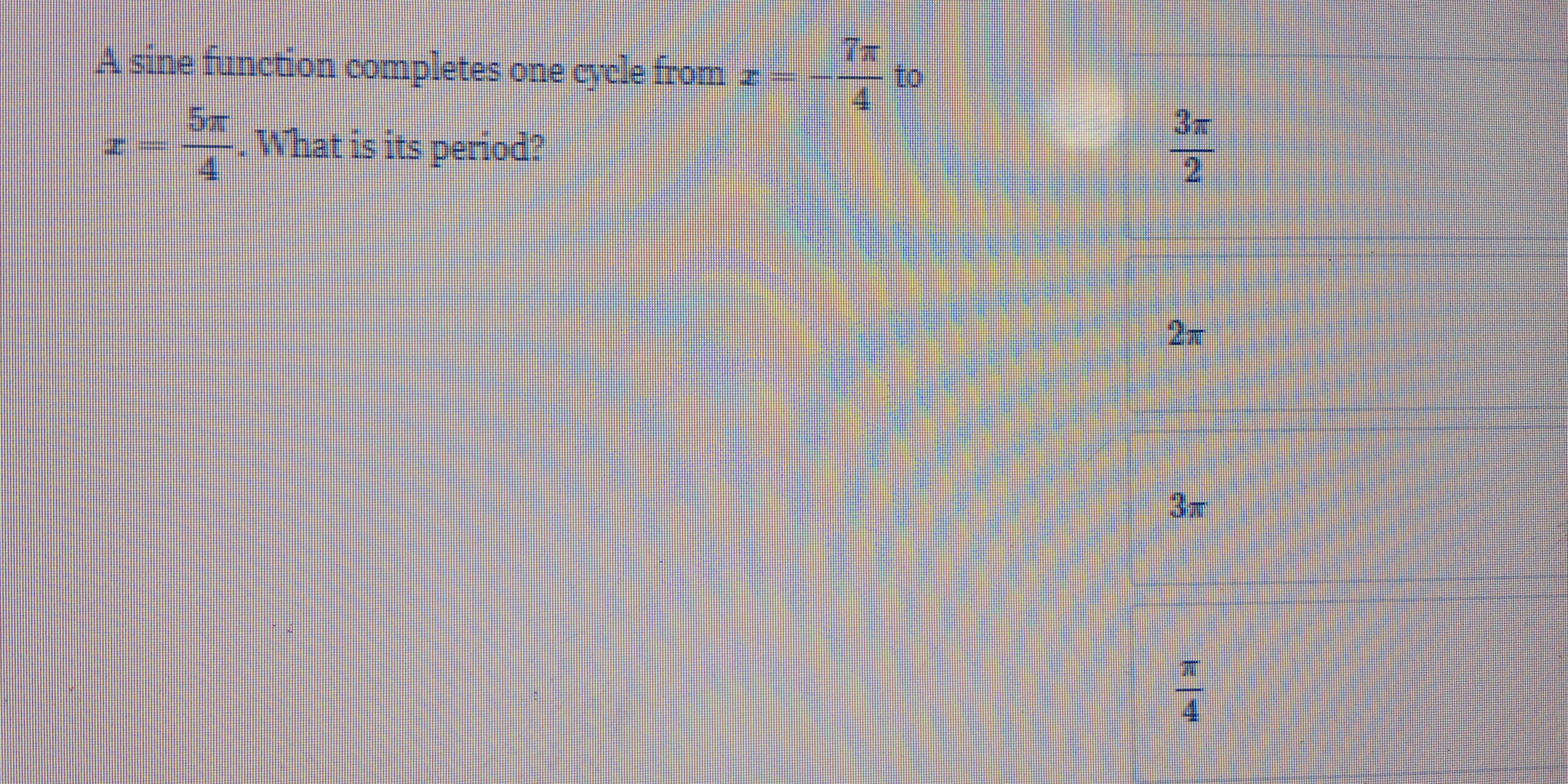
Understand the Problem

The question is asking for the period of a sine function that completes one full cycle between two given angles, specifically from ( x = \frac{7\pi}{4} ) to ( x = \frac{-5\pi}{4} ). We will calculate the distance between these two angles to determine the period.

Answer

The period is \( 3\pi \).

Steps to Solve

Identify the Given Angles

The sine function completes one cycle from ( x = \frac{7\pi}{4} ) to ( x = -\frac{5\pi}{4} ).

Convert the Angles to a Common Format

To better understand the interval, we can convert the angles to degrees if necessary, or keep them in radians for this calculation. The angles in radians are:

( \frac{7\pi}{4} = 315^\circ )
( -\frac{5\pi}{4} = -225^\circ )

Calculate the Distance Between Angles

To find the period, calculate the difference in radians between the two angles.

[ \text{Distance} = \left| \frac{7\pi}{4} - \left(-\frac{5\pi}{4}\right) \right| ]

Compute the Difference

Simplifying the expression gives:

[ \text{Distance} = \left| \frac{7\pi}{4} + \frac{5\pi}{4} \right| = \left| \frac{12\pi}{4} \right| = 3\pi ]

Conclusion about the Period

The calculated distance ( 3\pi ) represents the full period of the sine function over the specified interval.

The period of the sine function is ( 3\pi ).

More Information

The period of a sine function can vary depending on the specific function being analyzed. The standard period for ( \sin(x) ) is ( 2\pi ), but when specified over a particular range, such as in this case, the period can be different.

Tips

Forgetting to compute the absolute value of the difference when calculating the distance.
Not recognizing that the sine function repeats every full cycle, which can lead to confusion about finding the period.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!