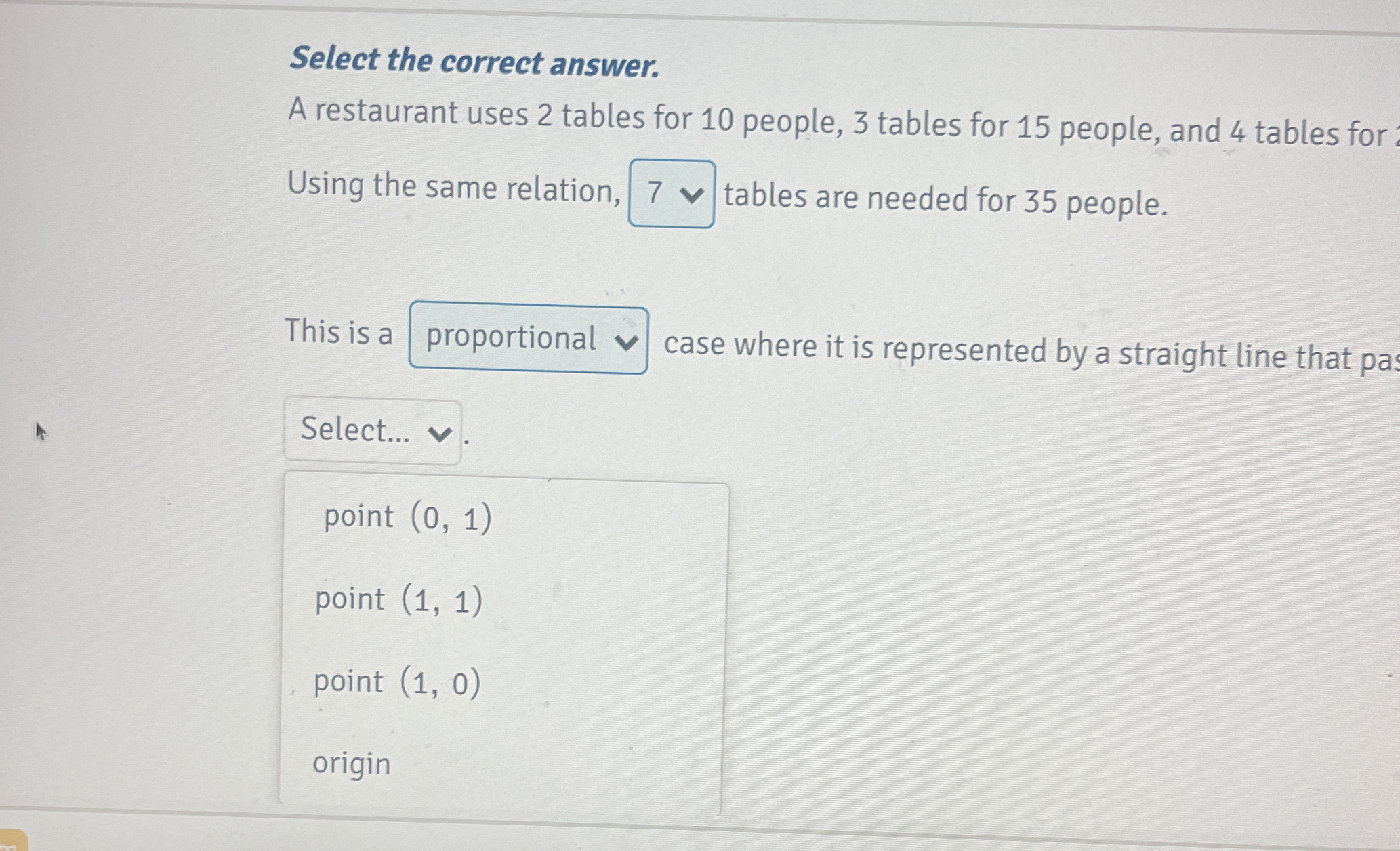
A restaurant uses 2 tables for 10 people, 3 tables for 15 people, and 4 tables for 20 people. Using the same relation, 7 tables are needed for 35 people. This is a proportional cas... A restaurant uses 2 tables for 10 people, 3 tables for 15 people, and 4 tables for 20 people. Using the same relation, 7 tables are needed for 35 people. This is a proportional case where it is represented by a straight line that passes through which point?
Understand the Problem
The question involves determining the amount of tables needed for a proportional relation. It states that a restaurant uses a certain number of tables for a given number of people and asks for tables needed for 35 people. Then, it requires selecting the correct representation of this relation from the provided options.
Answer
The answer is \(7\) tables needed for \(35\) people.
Answer for screen readers
The number of tables needed for 35 people is (7).
Steps to Solve
-
Identify the relationship The relationship between the number of tables and the number of people is proportional. This means that as the number of people increases, the number of tables required increases in a fixed ratio.
-
Calculate the ratio We can find the ratio of tables to people from the initial data:
- For 10 people, 2 tables are used.
- For 15 people, 3 tables are used.
- For 20 people, 4 tables are used. The consistent ratio can be calculated as follows: $$ \text{Ratio} = \frac{\text{Tables}}{\text{People}} $$
-
Use one of the ratios to find the constant Using 2 tables for 10 people: $$ k = \frac{2}{10} = 0.2 $$ This constant can be used to calculate the number of tables needed for any number of people.
-
Apply the constant to 35 people To find the number of tables needed for 35 people: $$ \text{Tables} = k \times \text{People} $$ $$ \text{Tables} = 0.2 \times 35 = 7 $$
-
Select the correct representation The proportional relationship is typically represented by coordinates. Since (7) tables for (35) people is the resulting ratio, you need to express this in coordinate form.
-
Determine the coordinate In a proportional relationship with a line passing through the origin, we can use the coordinates as points. The origin represents (0,0).
The number of tables needed for 35 people is (7).
More Information
In a proportional relationship, the ratio remains constant. For every increase in the number of people, the increase in tables is proportional, meaning the relationship is linear and can be visualized with a straight line through the origin.
Tips
- Confusing proportional relationships with non-proportional ones.
- Failing to simplify the ratio properly.
AI-generated content may contain errors. Please verify critical information