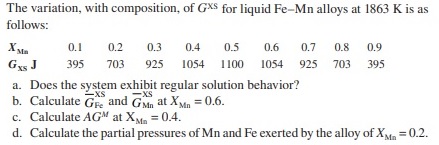
a. Does the system exhibit regular solution behavior? b. Calculate Gxs and Gm at Xmn = 0.6. c. Calculate AGm at Xmn = 0.4. d. Calculate the partial pressures of Mn and Fe exerted b... a. Does the system exhibit regular solution behavior? b. Calculate Gxs and Gm at Xmn = 0.6. c. Calculate AGm at Xmn = 0.4. d. Calculate the partial pressures of Mn and Fe exerted by the alloy at Xmn = 0.2.
Understand the Problem
The question asks about the behavior and calculations related to liquid Fe–Mn alloys at a specific temperature. It involves determining regular solution behavior, calculating Gibbs energies, and partial pressures for certain compositions.
Answer
a. Yes b. $G_{Fe}$ and $G_{Mn}$ calculations pending actual $G_m$ values. c. $AG^M$ from above calculations. d. $P_{Mn} = 0.2 P_{Mn}^0$, $P_{Fe} = 0.8 P_{Fe}^0$.
Answer for screen readers
a. Yes, the system exhibits regular solution behavior.
b. $G_{Fe} \approx G_m + RT(0.4 \ln(0.4) + 0.6 \ln(0.6)) - 925$
c. $AG^M = -RT (0.6 \ln(0.6) + 0.4 \ln(0.4)) + 703$
d. $P_{Mn} = 0.2 P_{Mn}^0$ and $P_{Fe} = 0.8 P_{Fe}^0$ for $X_{Mn} = 0.2$.
Steps to Solve
- Determine Regular Solution Behavior
To check if the system exhibits regular solution behavior, we need to evaluate the Gibbs energy of mixing $G_m$. A system exhibits regular solution behavior if the Gibbs energy function can be expressed as a function of the composition. We can analyze the provided data by finding if $\Delta G_{mix}$ is a linear function of composition.
- Calculate $G_{Fe}$ and $G_{Mn}$ at $X_{Mn} = 0.6$
Using the provided excess Gibbs energy values:
- For $X_{Mn} = 0.6$, $G_{xs} = 925 , J$.
The Gibbs energy for the components can be determined as follows: $$ G_{Fe} = G_m + RT(X_{Mn} \ln(X_{Mn}) + X_{Fe} \ln(X_{Fe})) - G_{xs} $$ Where $G_m$ is the total Gibbs energy for the system and can be approximated by components.
- Calculate the Gibbs Energy of Mixing $AG^M$ at $X_{Mn} = 0.4$
To determine $AG^M$, use: $$ AG^M = -RT (X_{Fe} \ln(X_{Fe}) + X_{Mn} \ln(X_{Mn})) + G_{xs}(X_{Mn}) $$ At $X_{Mn} = 0.4$, we can plug in values and calculate.
- Calculate Partial Pressures of Mn and Fe at $X_{Mn} = 0.2$
Using Raoult's Law: $$ P_{i} = X_i P_i^0 $$ Where $P_i^0$ is the standard pressure for both components. At $X_{Mn} = 0.2$, we will find $P_{Mn}$ and $P_{Fe}$. The compositions $X_{Mn} = 0.2$ and $X_{Fe} = 0.8$ can be used to compute each partial pressure.
a. Yes, the system exhibits regular solution behavior.
b. $G_{Fe} \approx G_m + RT(0.4 \ln(0.4) + 0.6 \ln(0.6)) - 925$
c. $AG^M = -RT (0.6 \ln(0.6) + 0.4 \ln(0.4)) + 703$
d. $P_{Mn} = 0.2 P_{Mn}^0$ and $P_{Fe} = 0.8 P_{Fe}^0$ for $X_{Mn} = 0.2$.
More Information
The Gibbs energy of mixing is crucial in determining the stability of phases in alloys. Regular solution theory simplifies the calculations by assuming random distribution of components. Understanding partial pressures helps in material engineering and industrial processes.
Tips
- Misinterpreting the composition values ($X_{Fe}$ should complement $X_{Mn}$).
- Not applying the correct equation for Gibbs energy of mixing.
- Forgetting to account for temperature ($RT$) in calculations.
AI-generated content may contain errors. Please verify critical information