5172✳ is a 5-digit number. If the number is divisible by 6, find all the possible values of ✳.

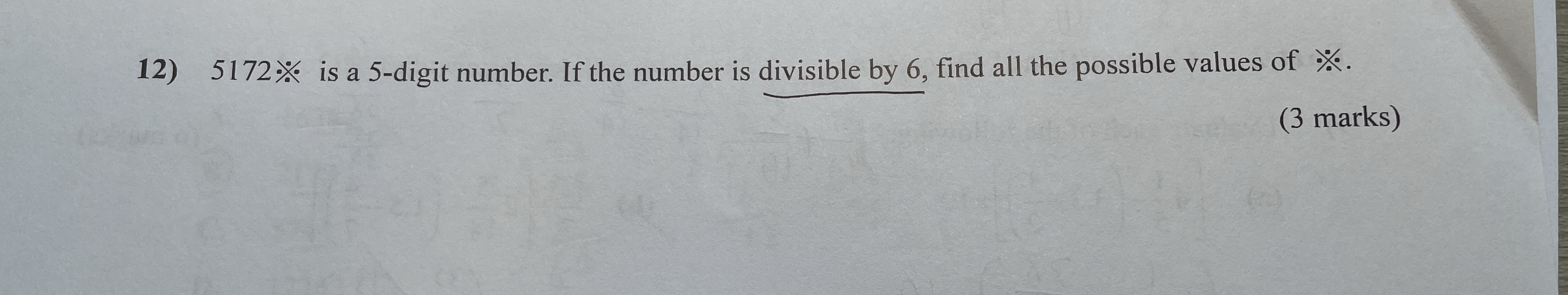
Understand the Problem

The question is asking to find all possible values of the unknown digit represented by '✳' in the 5-digit number '5172✳', given that the number is divisible by 6. To determine divisibility by 6, the number must be divisible by both 2 and 3.

Answer

The values of '✳' are \(0\) and \(6\).

Steps to Solve

Check Divisibility by 2 A number is divisible by 2 if its last digit is even. Here, the last digit is represented by '✳'. Thus, the possible values for '✳' are (0, 2, 4, 6, 8).
Calculate the Sum of the Digits Next, find the sum of the known digits of the number (5172✳): [ 5 + 1 + 7 + 2 = 15 ] Now, include '✳': [ 15 + ✳ ] The number must be divisible by 3.
Find Values of '✳' for Divisibility by 3 Determine which values of '✳' make (15 + ✳) divisible by 3. We check the even values (0, 2, 4, 6, 8):

When (✳ = 0): [ 15 + 0 = 15 \quad (15 \div 3 = 5) , \text{(divisible)} ]
When (✳ = 2): [ 15 + 2 = 17 \quad (17 \div 3 \neq 5) , \text{(not divisible)} ]
When (✳ = 4): [ 15 + 4 = 19 \quad (19 \div 3 \neq 6) , \text{(not divisible)} ]
When (✳ = 6): [ 15 + 6 = 21 \quad (21 \div 3 = 7) , \text{(divisible)} ]
When (✳ = 8): [ 15 + 8 = 23 \quad (23 \div 3 \neq 7) , \text{(not divisible)} ]

Summarize Valid Values The valid values of '✳' that make (5172✳) divisible by 6 are found to be (0) and (6).

The possible values of '✳' are (0) and (6).

More Information

For a number to be divisible by 6, it must be divisible by both 2 and 3. The analysis confirms that both (0) and (6) meet this requirement, showcasing how numerical properties can guide problem-solving.

Tips

Forgetting to check both conditions for divisibility. Always confirm the number meets both requirements to ensure it qualifies for divisibility by 6.
Not considering all even numbers for '✳'.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!