-2 - 2x > -8

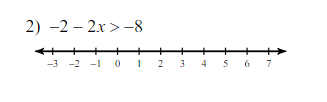
Understand the Problem

The question involves solving an inequality, specifically -2 - 2x > -8. We need to rearrange the inequality and isolate the variable x to find its possible values.

Answer

The solution to the inequality is $ x < 3 $.

Steps to Solve

Isolate the term with the variable x

Start with the original inequality: $$ -2 - 2x > -8 $$

Add 2 to both sides to isolate the term with $x$: $$ -2x > -8 + 2 $$

This simplifies to: $$ -2x > -6 $$

Divide by the coefficient of x

Now, divide both sides by -2. Remember, when you divide or multiply an inequality by a negative number, you must flip the inequality sign: $$ x < \frac{-6}{-2} $$

This simplifies to: $$ x < 3 $$

Solution Interpretation

The solution to the inequality is: $$ x < 3 $$

This means any value of $x$ less than 3 will satisfy the inequality.

The solution to the inequality is ( x < 3 ).

More Information

Understanding inequalities is essential in algebra, as it helps us determine the range of values that a variable can take. Graphically, the solution can be represented on a number line with an open circle at 3, indicating that 3 is not included in the solution.

Tips

Forgetting to flip the inequality sign when dividing by a negative number. Always remember to reverse the inequality direction in such cases.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!