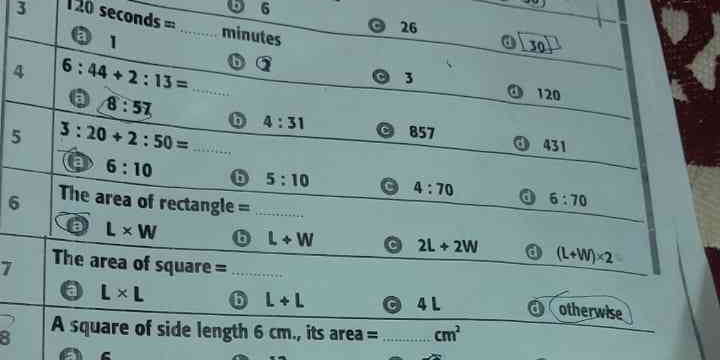
120 seconds = ? minutes, 6:44 + 2:13 = ?, 3:20 + 2:50 = ?, The area of rectangle = ?, The area of square = ?, A square of side length 6 cm, its area = ?

Steps to Solve

1. Convert seconds to minutes
   To convert 120 seconds to minutes, divide by 60.
   $$ \text{minutes} = \frac{120 \text{ seconds}}{60} = 2 \text{ minutes} $$

2. Calculate the time
   For the equation $6:44 + 2:13$, add the minutes and seconds separately.
   $$ 44 + 13 = 57 \text{ minutes} $$
   Add the hours:
   $$ 6 + 2 = 8 \text{ hours} $$
   Thus, the total time is $8:57$.

3. Perform the addition
   For $3:20 + 2:50$, add minutes and seconds:
   $$ 20 + 50 = 70 \text{ minutes} \Rightarrow 1 \text{ hour} + 10 \text{ minutes} $$
   Add the hours:
   $$ 3 + 2 + 1 = 6 \text{ hours} $$
   So, the total is $6:10$.

4. Identify the area of a rectangle
   The area of a rectangle is calculated as:
   $$ \text{Area} = L \times W $$

5. Identify the area of a square
   The area of a square is calculated as:
   $$ \text{Area} = L \times L \text{ (or } L^2\text{)} $$

6. Calculate the area of a square with side length 6 cm
   Substituting $L = 6$ into the area formula:
   $$ \text{Area} = 6 \times 6 = 36 \text{ cm}^2 $$