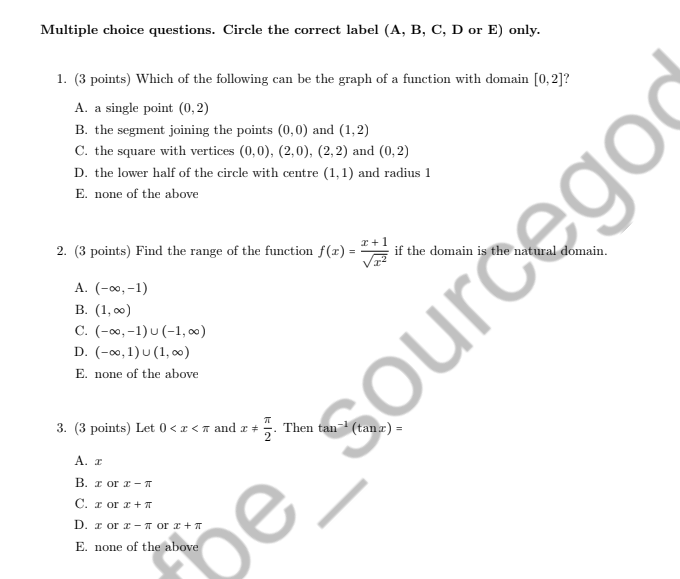
1. Which of the following can be the graph of a function with domain [0,2]? A. a single point (0,2) B. the segment joining the points (0,0) and (1,2) C. the square with vertices... 1. Which of the following can be the graph of a function with domain [0,2]? A. a single point (0,2) B. the segment joining the points (0,0) and (1,2) C. the square with vertices (0,0), (2,0), (2,2) and (0,2) D. the lower half of the circle with centre (1,1) and radius 1 E. none of the above 2. Find the range of the function f(x) = x + 1 / √x^2 if the domain is the natural domain. A. (-∞,-1) B. (1,∞) C. (-∞,-1) ∪ (-1,∞) D. (-∞,1) ∪ (1,∞) E. none of the above 3. Let 0 < x < π and x ≠ π/2. Then tan^(-1)(tan x) = A. x B. x or x - π C. x or x + π D. x or x - π or x + π E. none of the above Read more

Steps to Solve

1. Identify the first question's requirements

The first question checks which of the options can represent a function with the specified domain $[0, 2]$. A valid function must pass the vertical line test, meaning that no vertical line can intersect the graph at more than one point.

2. Analyze the options for Question 1

• A. A single point $(0, 2)$ is valid since it defines a function.

• B. The segment joining $(0, 0)$ and $(1, 2)$ is valid since it passes the vertical line test.

• C. The square with vertices $(0, 0), (2, 0), (2, 2), (0, 2)$ fails, as vertical lines will intersect it at multiple points.

• D. The lower half of a circle will also fail the vertical line test.

3. Determine the correct option for Question 1

The valid choices are A and B. Based on the domain $[0, 2]$, either option can represent the graph of a function. More than one valid answer here implies "none of the above" might be considered inaccurate.

4. Identify the second question's requirements

The second question requires finding the range of the function $f(x) = \frac{x + 1}{\sqrt{x^2}}$. Simplifying this function first is necessary.

5. Simplify the function for Question 2

Since $\sqrt{x^2} = |x|$, we rewrite the function as: $$ f(x) = \begin{cases} \frac{x + 1}{x}, & x > 0 \ \frac{x + 1}{-x}, & x < 0 \end{cases} $$

6. Analyze the function for the range

For $x > 0$, $f(x) = 1 + \frac{1}{x}$, and as $x \to 0^+$, $f(x) \to \infty$. For $x < 0$, the function $f(x) = -1 - \frac{1}{|x|}$ approaches $-\infty$ as $x \to 0^-$. Thus, the function ranges from $(-\infty, -1) \cup (1, \infty)$.

7. Identify the correct option for Question 2

The correct option is C: $(-\infty, -1) \cup (1, \infty)$.

8. Identify the third question's requirements

The question asks for the value of $\tan^{-1}(\tan x)$ for $0 < x < \pi$ and $x \neq \frac{\pi}{2}$. We need to apply the properties of the inverse tangent and tangent functions.

9. Evaluate $\tan^{-1}(\tan x)$

The result is $x$ when $x$ is within the range of $\tan^{-1}$. Since $0 < x < \pi$, we adjust for the discontinuities of $\tan$ in that interval.

10. Identify the correct option for Question 3

The value of $\tan^{-1}(\tan x)$ yields different results depending on where $x$ falls in terms of multiples of $\pi$. For $0 < x < \pi$, our correct answer aligns with option B, which states the two possibilities for when x lies in intervals appropriately adjusted for quadrant checks.