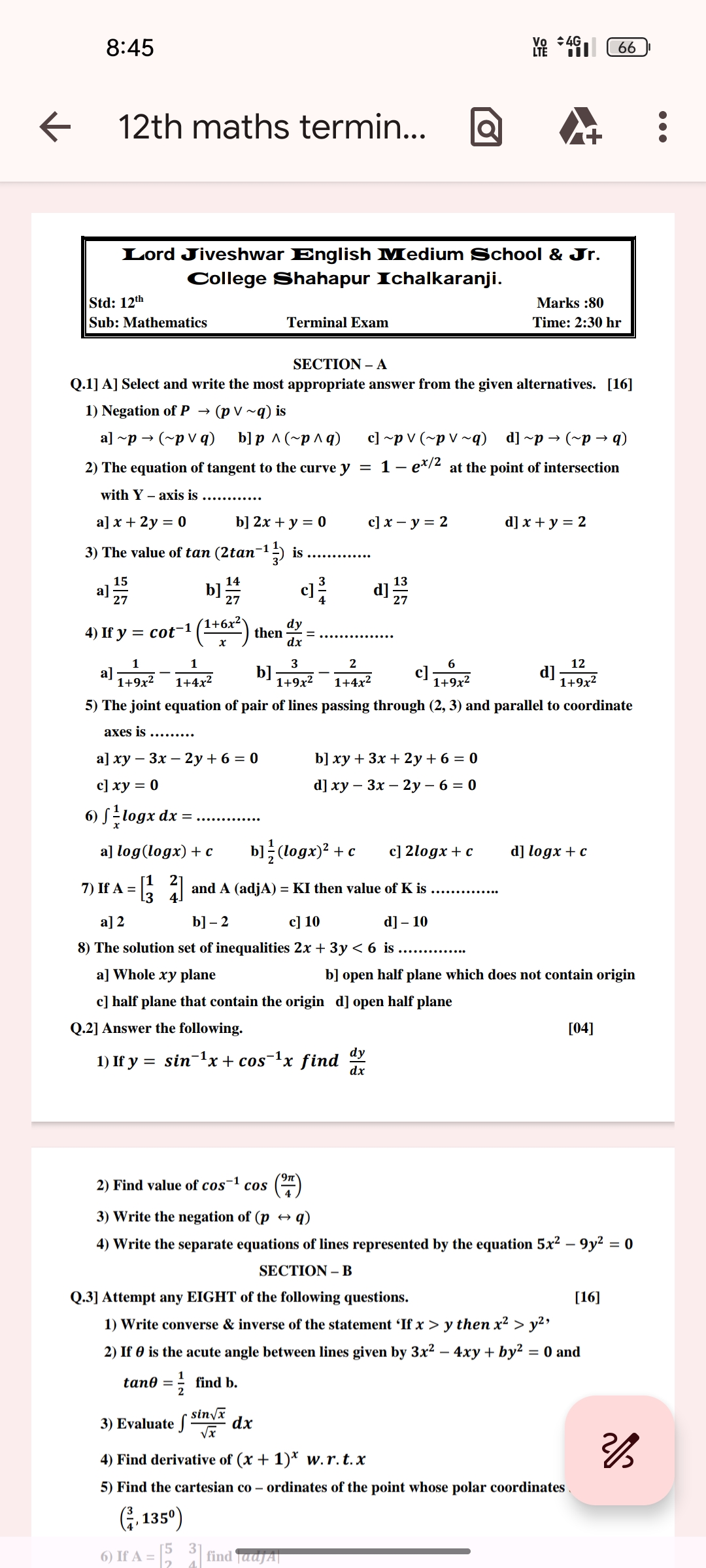
1) Negation of P → (p v ~q) is a) ~p → (~p v q) b) p ∧ (~p ∧ q) c) ~p v (~p v ~q) d) ~p → (~p v q) 2) The equation of tangent to the curve y = 1 - e^(x/2) at the point of intersect... 1) Negation of P → (p v ~q) is a) ~p → (~p v q) b) p ∧ (~p ∧ q) c) ~p v (~p v ~q) d) ~p → (~p v q) 2) The equation of tangent to the curve y = 1 - e^(x/2) at the point of intersection with Y-axis is ……….. 3) The value of tan(2tan^{-1}(3)) is ……….. 4) If y = cot^{-1}(1 + 6x^2) then dy/dx = ……….. 5) The joint equation of pair of lines passing through (2, 3) and parallel to coordinate axes is ………. 6) ∫(1/x) logx dx = ………. 7) If A = [3 2; 4 0] and A (adjA) = K then value of K is ……… 8) The solution set of inequalities 2x + 3y < 6 is ……… 1) If y = sin^{-1}x + cos^{-1}x find dy/dx 2) Find the value of cos^{-1}(cos(9π/4)) 3) Write the negation of (p ↔ q) 4) Write the separate equations of lines represented by the equation 5x^2 - 9y^2 = 0. Read more

Steps to Solve

1. Negation of ( P \to (P \lor \neg Q) )

The logical statement ( P \to (P \lor \neg Q) ) can be rewritten using the implication equivalence: $$ P \to Q \equiv \neg P \lor Q $$ Thus, $$ \neg (P \to (P \lor \neg Q)) \equiv \neg (\neg P \lor (P \lor \neg Q)) $$

2. Understanding the Geometry of the Tangent Equation

To find the equation of the tangent to the curve ( y = 1 - e^{-\frac{x}{2}} ), we need to find the derivative ( \frac{dy}{dx} ). Using the rules of differentiation, $$ \frac{dy}{dx} = \frac{1}{2} e^{-\frac{x}{2}} $$

3. Finding the Value of ( \tan\left(\tan^{-1}(\frac{1}{3})\right) )

To find ( \tan(2 \tan^{-1}(\frac{1}{3})) ), we use the double angle formula: $$ \tan(2\theta) = \frac{2\tan(\theta)}{1 - \tan^2(\theta)} $$ Setting ( \theta = \tan^{-1}(\frac{1}{3}) ): $$ \tan(2 \tan^{-1}(\frac{1}{3})) = \frac{2 \cdot \frac{1}{3}}{1 - (\frac{1}{3})^2} = \frac{\frac{2}{3}}{1 - \frac{1}{9}} = \frac{\frac{2}{3}}{\frac{8}{9}} = \frac{2 \cdot 9}{3 \cdot 8} = \frac{6}{8} = \frac{3}{4} $$

4. Finding ( \frac{dy}{dx} ) for ( y = \cot^{-1}\left(\frac{1 + 6x^2}{x}\right) )

Using the derivative of the inverse cotangent: $$ \frac{d}{dx}(\cot^{-1}(u)) = -\frac{1}{1 + u^2} \cdot \frac{du}{dx} $$ Let ( u = \frac{1 + 6x^2}{x} ), then calculate ( \frac{du}{dx} ) and substitute into the derivative formula.

5. Finding the Joint Equation of Lines

A joint equation for two lines passing through (2,3) and being parallel to coordinate axes can be expressed as: $$ (y - 3) = m(x - 2) $$ Separate this into horizontal and vertical components to get equations like ( y - 3 = 0 ) for horizontal and ( x - 2 = 0 ) for vertical lines.

6. Evaluating ( \int \frac{1}{x} \log{x} ,dx )

This can be solved using integration by parts: Let ( u = \log{x} ) and ( dv = \frac{1}{x}dx ) leading to: $$ du = \frac{1}{x}dx, \quad v = \log{x} $$

7. Finding the Determinant and Adjusting

For the matrix ( A ): $$ A = \begin{bmatrix} 3 & 2 \ 4 & K \end{bmatrix} $$ To find (|A|) set ( \text{det}(A) = 3K - 8 = 1 ) and solve for ( K ).