1. Integrate the following by using tabular integration: an = (2/1) ∫ x^2(1 - x)cos(nπx) dx. 2. By using trigonometric substitution, integrate ∫ √(1 - x^2) dx. 3. Find all minors o... 1. Integrate the following by using tabular integration: an = (2/1) ∫ x^2(1 - x)cos(nπx) dx. 2. By using trigonometric substitution, integrate ∫ √(1 - x^2) dx. 3. Find all minors of the matrix A = [3 4 -1; 1 0 3; 2 -5 -4].
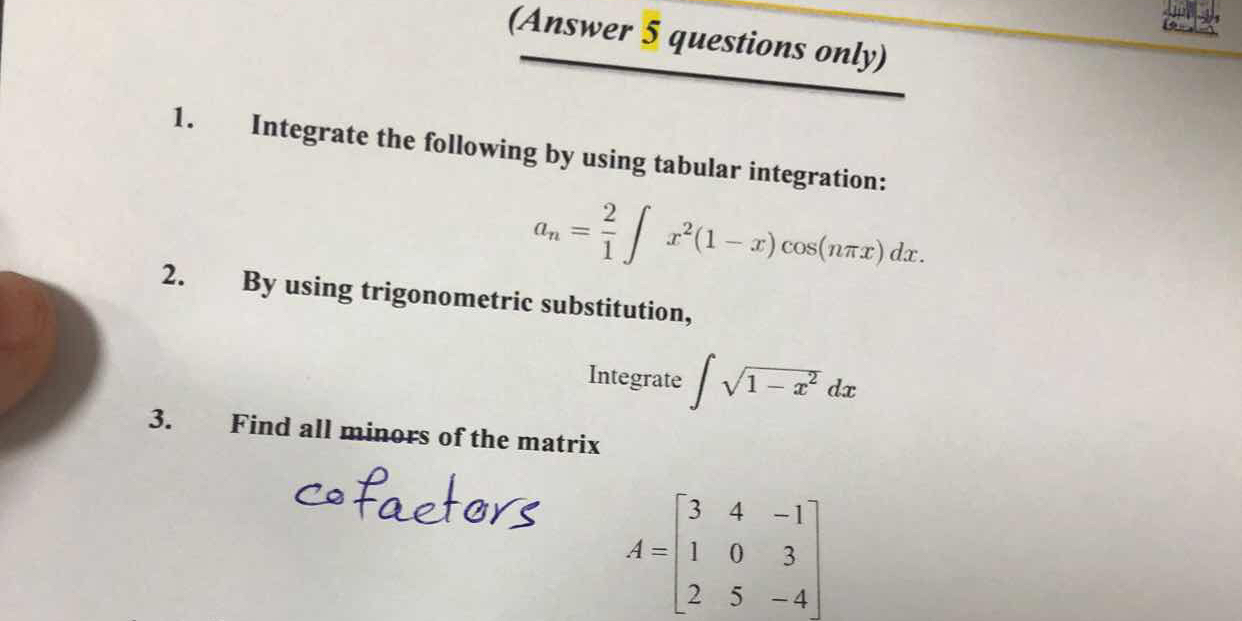
Understand the Problem
The question contains three parts related to calculus and linear algebra. It asks to integrate a function using tabular integration and trigonometric substitution, and to find the minors of a given matrix.
Answer
The first integral is complex and requires multiple steps. The second integral yields an expression in $x$. Minors calculated from matrix $A$.
Answer for screen readers
- The result of the first integral using tabular integration is a more complex expression that may require simplification.
- The result of the second integral using trigonometric substitution is expressed in terms of $x$ from the substituted variable.
- The minors of the matrix $A$ are calculated based on the corresponding determinants.
Steps to Solve
- Tabular Integration Setup
For the integral $a_n = \frac{2}{1} \int x^2 (1 - x) \cos(n \pi x) , dx$, we identify parts for integration by parts (IBP):
Let:
- $u = x^2 (1 - x) \Rightarrow du = (2x - 3x^2) , dx$
- $dv = \cos(n \pi x) , dx \Rightarrow v = \frac{1}{n \pi} \sin(n \pi x)$
- Applying Integration by Parts
Using the integration by parts formula $\int u , dv = uv - \int v , du$:
Calculate: $$ \int x^2(1 - x) \cos(n \pi x) , dx = \frac{x^2(1 - x)}{n \pi} \sin(n \pi x) - \int \frac{\sin(n \pi x)}{n \pi} (2x - 3x^2) , dx $$
- Integrate Again as Necessary
Continue integrating the remaining integral, applying IBP as needed, until reaching simpler integrals.
- Trigonometric Substitution
For $\int \sqrt{1 - x^2} , dx$, we use the substitution $x = \sin(\theta) \Rightarrow dx = \cos(\theta) , d\theta$.
Thus, the integral becomes: $$ \int \sqrt{1 - \sin^2(\theta)} \cos(\theta) , d\theta = \int \cos^2(\theta) , d\theta $$
- Integrate Using Trigonometric Identity
Utilize the identity $\cos^2(\theta) = \frac{1 + \cos(2\theta)}{2}$: $$ \int \cos^2(\theta) , d\theta = \frac{1}{2} \left( \theta + \frac{1}{2} \sin(2\theta) \right) + C $$
Convert back to $x$ to express the result in terms of $x$.
- Finding Minors of the Matrix
Given matrix $A = \begin{bmatrix} 3 & 4 & -1 \ 1 & 0 & 3 \ 2 & 5 & -4 \end{bmatrix}$, find minors for each element.
The minor for element $a_{ij}$ is calculated as the determinant of the submatrix obtained by removing the $i^{th}$ row and $j^{th}$ column.
- Calculate Determinants for Minors
For example, the minor $M_{11}$ (removing the first row and first column): $$ M_{11} = \begin{vmatrix} 0 & 3 \ 5 & -4 \end{vmatrix} = (0)(-4) - (3)(5) = -15 $$
Repeat to find all other minors.
- The result of the first integral using tabular integration is a more complex expression that may require simplification.
- The result of the second integral using trigonometric substitution is expressed in terms of $x$ from the substituted variable.
- The minors of the matrix $A$ are calculated based on the corresponding determinants.
More Information
- The tabular integration is effective for polynomials multiplied by trigonometric functions.
- Trigonometric substitution is useful for integrals involving square roots of quadratics.
- Minors are used particularly when calculating the determinant or eigenvalues of the matrix.
Tips
- Forgetting to simplify the result after applying integration by parts.
- Not correctly addressing the limits of integration.
- Confusing the calculation of minors with cofactors (remember minors use simpler determinants).
AI-generated content may contain errors. Please verify critical information