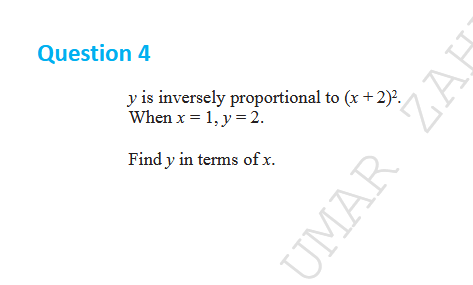
y is inversely proportional to (x + 2)^2. When x = 1, y = 2. Find y in terms of x.
Understand the Problem
The question is asking us to express y in terms of x, given that y is inversely proportional to the square of (x + 2) and a specific value for y when x is 1. We will use the proportionality relationship to derive the equation.
Answer
$$ y = \frac{18}{(x + 2)^2} $$
Answer for screen readers
The expression for $y$ in terms of $x$ is: $$ y = \frac{18}{(x + 2)^2} $$
Steps to Solve
-
Set up the relationship Since $y$ is inversely proportional to $(x + 2)^2$, we can write this relationship as: $$ y = \frac{k}{(x + 2)^2 $$ where $k$ is a constant.
-
Determine the constant of proportionality (k) We use the provided value of $y$ when $x = 1$. Substituting these values into the equation: $$ 2 = \frac{k}{(1 + 2)^2} $$ Calculating $(1 + 2)^2$ gives us $3^2 = 9$. Thus: $$ 2 = \frac{k}{9} $$
-
Solve for k To find $k$, multiply both sides by 9: $$ k = 2 \cdot 9 = 18 $$
-
Write the equation for y Now substitute $k$ back into the original proportionality equation: $$ y = \frac{18}{(x + 2)^2} $$
The expression for $y$ in terms of $x$ is: $$ y = \frac{18}{(x + 2)^2} $$
More Information
This result shows how the value of $y$ varies as $x$ changes. The factor of $18$ indicates the initial scaling of the inversely proportional relationship when $x = 1$.
Tips
- Confusing direct and inverse proportions: Make sure to recognize that "inversely proportional" means as one quantity increases, the other decreases.
- Incorrectly calculating the constant k: Always double-check calculations when determining the constant from given values.
AI-generated content may contain errors. Please verify critical information