y is directly proportional to the positive square root of x. When x = 9, y = 12. Find y when x = 1/4.

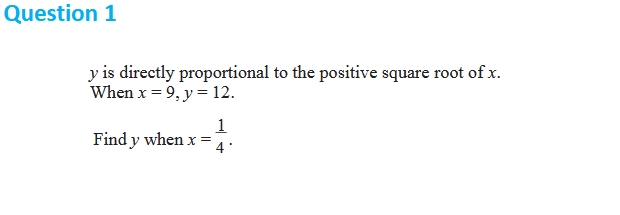
Understand the Problem

The question is asking to determine the value of y when x equals 1/4, given that y is directly proportional to the positive square root of x and an initial condition where x is 9 and y is 12.

Answer

$2$

Steps to Solve

Set up the proportionality equation

Since $y$ is directly proportional to the positive square root of $x$, we can express this relationship with the equation: $$ y = k \sqrt{x} $$ where $k$ is the constant of proportionality.

Find the constant of proportionality (k)

Using the initial condition where $x = 9$ and $y = 12$, substitute these values into the equation: $$ 12 = k \sqrt{9} $$ This simplifies to: $$ 12 = k \cdot 3 $$ Now, solve for $k$: $$ k = \frac{12}{3} = 4 $$

Update the equation with the constant (k)

Now that we know $k$, we can write the equation for $y$ as: $$ y = 4 \sqrt{x} $$

Calculate y when x = 1/4

Now substitute $x = \frac{1}{4}$ into the equation: $$ y = 4 \sqrt{\frac{1}{4}} $$ This simplifies to: $$ y = 4 \cdot \frac{1}{2} = 2 $$

The value of $y$ when $x = \frac{1}{4}$ is $2$.

More Information

This problem demonstrates the concept of direct proportionality, where one variable changes in relation to the square root of another. It's useful in various fields such as physics and statistics for understanding relationships between quantities.

Tips

Confusing direct proportionality with inverse proportionality.
Failing to correctly calculate the constant of proportionality $k$.
Not simplifying the square root correctly.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!