∫ (x^2 + 4x^3 + 2) / (6x) dx
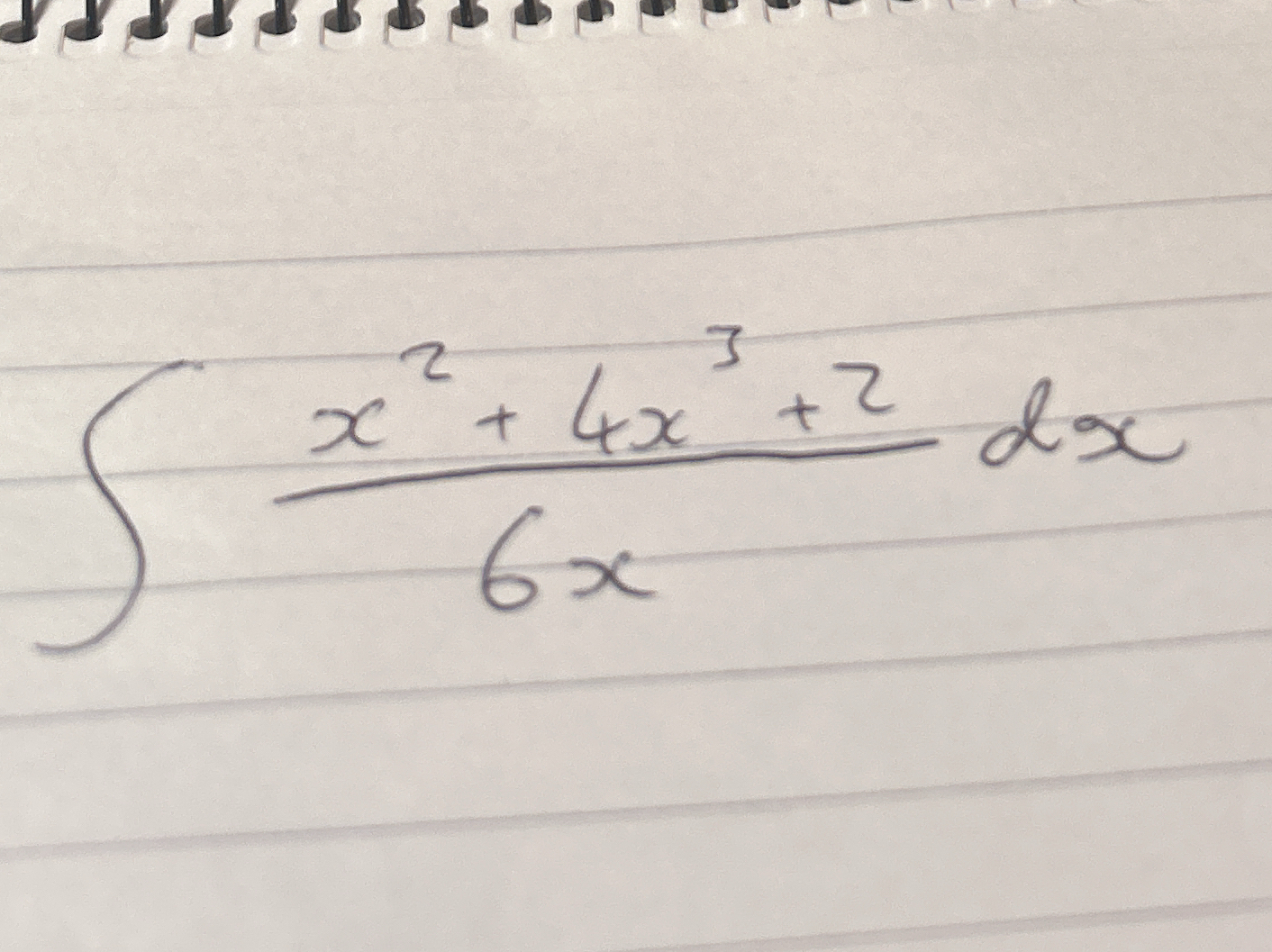
Understand the Problem
The question is asking to evaluate the integral of the expression given above, which involves polynomial terms in the numerator divided by a linear term in the denominator.
Answer
The evaluated integral is $$ \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
Answer for screen readers
The evaluated integral is
$$ \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
Steps to Solve
- Rewrite the Integral
First, simplify the expression by dividing each term in the numerator by the denominator $6x$:
$$ \int \frac{x^2}{6x} + \frac{4x^3}{6x} + \frac{2}{6x} , dx $$
This simplifies to:
$$ \int \left( \frac{x}{6} + \frac{2x^2}{3} + \frac{1}{3x} \right) , dx $$
- Separate the Integral
Now separate the integral into individual terms:
$$ \int \frac{x}{6} , dx + \int \frac{2x^2}{3} , dx + \int \frac{1}{3x} , dx $$
- Calculate Each Integral
Now calculate each integral one by one:
- For the first term:
$$ \int \frac{x}{6} , dx = \frac{1}{6} \cdot \frac{x^2}{2} = \frac{x^2}{12} $$
- For the second term:
$$ \int \frac{2x^2}{3} , dx = \frac{2}{3} \cdot \frac{x^3}{3} = \frac{2x^3}{9} $$
- For the third term:
$$ \int \frac{1}{3x} , dx = \frac{1}{3} \ln |x| $$
- Combine the Results
Now combine the results of the integrals:
$$ \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
where $C$ is the constant of integration.
The evaluated integral is
$$ \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
More Information
This integral involves simplifying a rational function before integrating, which is a common technique in calculus. The results combine polynomial terms and a logarithmic term, reflecting the different forms of integration.
Tips
- Misdividing the terms in the numerator when simplifying.
- Forgetting to include the constant of integration $C$.
- Confusing the integration rules for polynomials and logarithmic functions.
AI-generated content may contain errors. Please verify critical information