∫ (x^2 + 4x^3 + 2) / (6x) dx
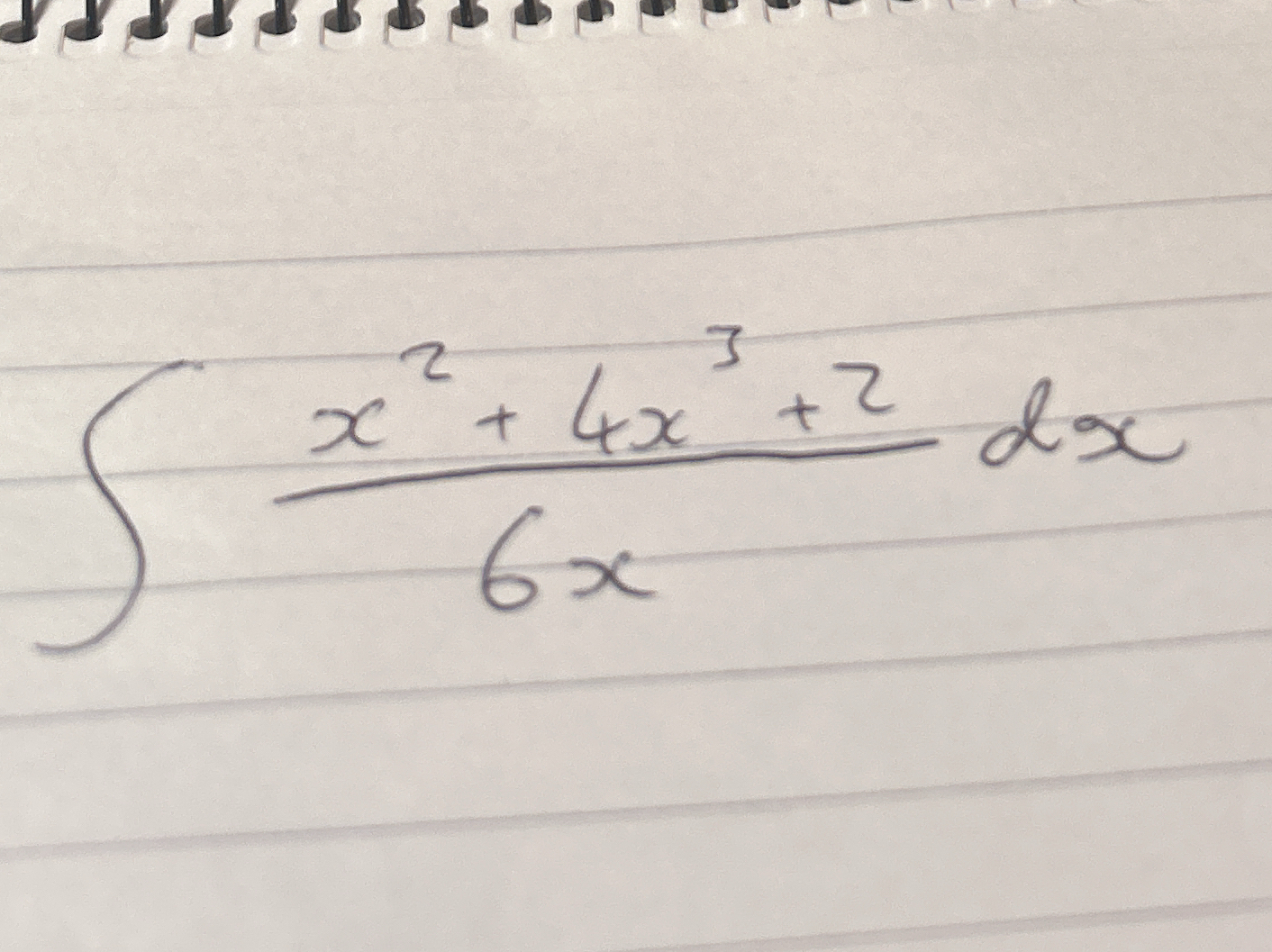
Understand the Problem
The question is asking for the integration of the expression (x^2 + 4x^3 + 2) divided by (6x). We will approach this by performing algebraic simplification before integrating.
Answer
$$ \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
Answer for screen readers
$$ \int \frac{x^2 + 4x^3 + 2}{6x} , dx = \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
Steps to Solve
-
Simplify the Expression First, we will divide each term in the numerator by the denominator $6x$: $$ \frac{x^2}{6x} + \frac{4x^3}{6x} + \frac{2}{6x} $$ This simplifies to: $$ \frac{x}{6} + \frac{2x^2}{3} + \frac{1}{3x} $$
-
Set Up the Integral Now we can set up the integral: $$ \int \left(\frac{x}{6} + \frac{2x^2}{3} + \frac{1}{3x}\right) dx $$
-
Integrate Each Term Integrate term by term:
- For $\frac{x}{6}$: $$ \int \frac{x}{6} dx = \frac{1}{6} \cdot \frac{x^2}{2} = \frac{x^2}{12} $$
- For $\frac{2x^2}{3}$: $$ \int \frac{2x^2}{3} dx = \frac{2}{3} \cdot \frac{x^3}{3} = \frac{2x^3}{9} $$
- For $\frac{1}{3x}$: $$ \int \frac{1}{3x} dx = \frac{1}{3} \ln |x| $$
- Combine the Results Now combine all integrated terms: $$ \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$ where $C$ is the constant of integration.
$$ \int \frac{x^2 + 4x^3 + 2}{6x} , dx = \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
More Information
This integral represents the accumulation of the area under the curve defined by the given function. The terms represent different powers of $x$, and the natural logarithm term corresponds to the integral of $\frac{1}{x}$.
Tips
- Forgetting to distribute the denominator to each term in the numerator.
- Not properly applying the power rule for integration.
- Neglecting to include the constant of integration $C$ after integrating.
AI-generated content may contain errors. Please verify critical information