∫ (x² + 4x³ + 2) / (6x) dx
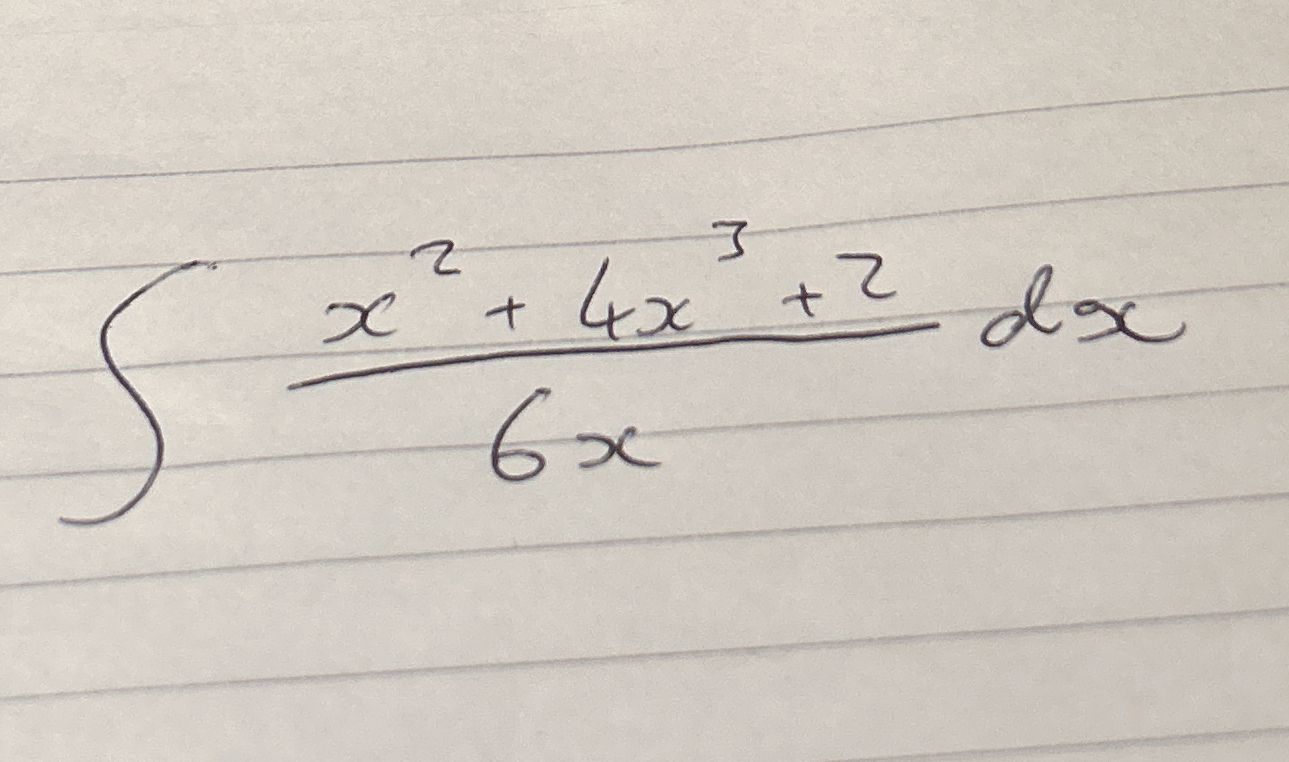
Understand the Problem
The question is asking to solve the integral of a rational function. We have to integrate (x² + 4x³ + 2) / (6x) with respect to x.
Answer
$$ \int \frac{x^2 + 4x^3 + 2}{6x} \, dx = \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
Answer for screen readers
The integral is given by:
$$ \int \frac{x^2 + 4x^3 + 2}{6x} , dx = \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
Steps to Solve
- Simplify the integrand
First, we divide each term in the numerator by the denominator $6x$:
$$ \frac{x^2}{6x} + \frac{4x^3}{6x} + \frac{2}{6x} $$
This simplifies to:
$$ \frac{x}{6} + \frac{2}{3}x^2 + \frac{1}{3x} $$
- Separate the integral
Next, express the integral as the sum of three separate integrals:
$$ \int \left( \frac{x}{6} + \frac{2}{3}x^2 + \frac{1}{3x} \right) dx = \int \frac{x}{6} dx + \int \frac{2}{3}x^2 dx + \int \frac{1}{3x} dx $$
- Compute each integral
Now, we compute each integral individually:
- For the first term:
$$ \int \frac{x}{6} dx = \frac{1}{6} \cdot \frac{x^2}{2} = \frac{x^2}{12} $$
- For the second term:
$$ \int \frac{2}{3}x^2 dx = \frac{2}{3} \cdot \frac{x^3}{3} = \frac{2x^3}{9} $$
- For the third term:
$$ \int \frac{1}{3x} dx = \frac{1}{3} \ln |x| $$
- Combine the results
The final integral result combines all computed integrals and includes a constant of integration $C$:
$$ \int \frac{x^2 + 4x^3 + 2}{6x} dx = \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
The integral is given by:
$$ \int \frac{x^2 + 4x^3 + 2}{6x} , dx = \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
More Information
This result demonstrates the application of basic integral calculus involving rational functions. When integrating, each term can be treated separately, simplifying calculations.
Tips
- Forgetting to separate the integral into simpler parts.
- Misapplying the power rule for integration, particularly with constants.
- Ignoring the absolute value in the logarithm term.