∫(x² + 4x³ + 2) / (6x) dx
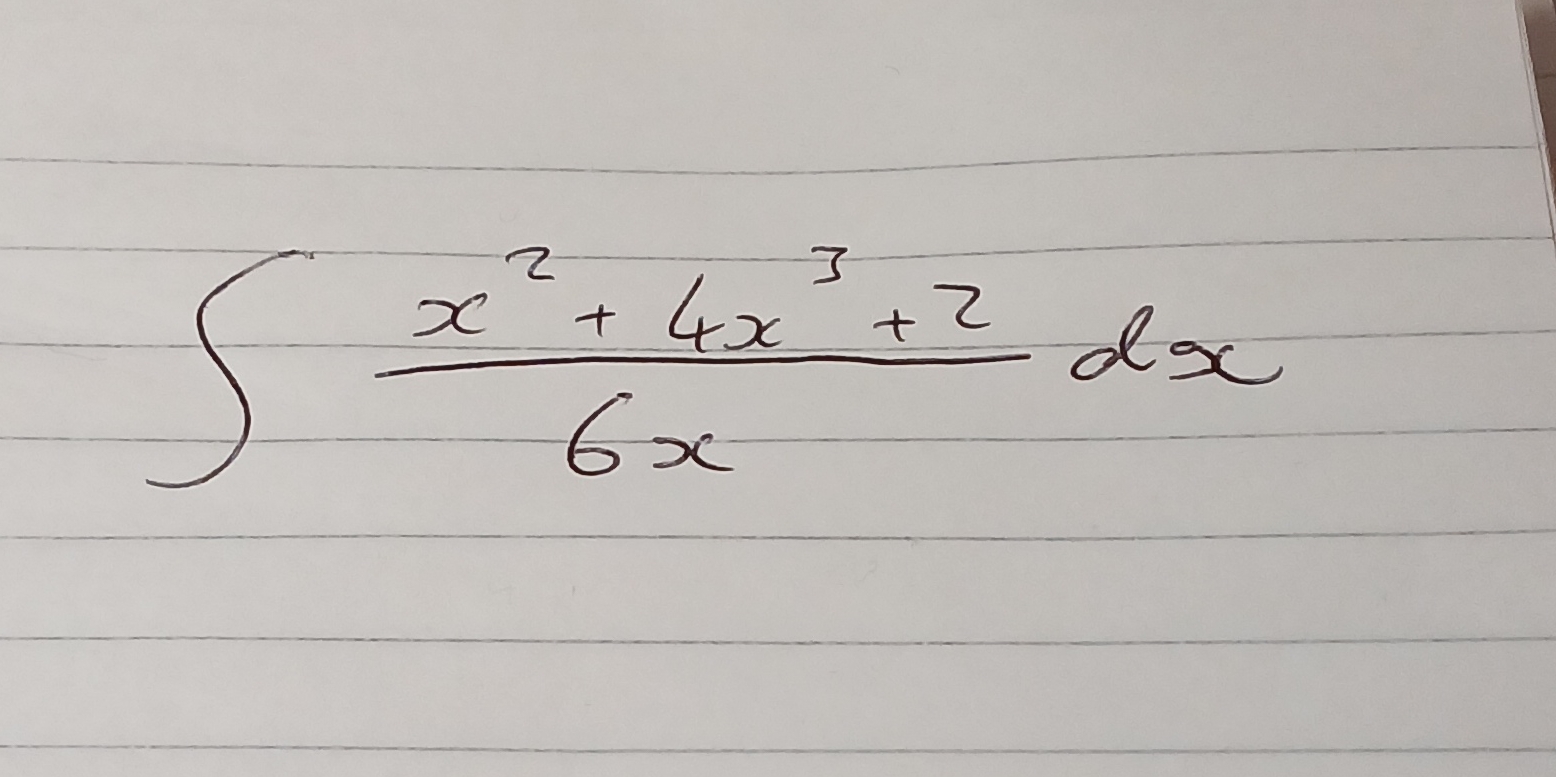
Understand the Problem
The question is asking for the evaluation of a definite integral involving a polynomial function divided by a linear function. This requires applying techniques from calculus to simplify and integrate the expression.
Answer
$$ \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
Answer for screen readers
The solution to the integral is:
$$ \int \frac{x^2 + 4x^3 + 2}{6x} , dx = \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
Steps to Solve
- Simplify the expression
To simplify the integral $\int \frac{x^2 + 4x^3 + 2}{6x} , dx$, divide each term in the numerator by the denominator $6x$:
$$ \frac{x^2}{6x} + \frac{4x^3}{6x} + \frac{2}{6x} = \frac{x}{6} + \frac{2}{3}x^2 + \frac{1}{3x} $$
Thus, the integral can be rewritten as:
$$ \int \left( \frac{x}{6} + \frac{2}{3} x^2 + \frac{1}{3x} \right) , dx $$
- Integrate term by term
Now we can integrate each term separately:
- The integral of $\frac{x}{6}$ is:
$$ \int \frac{x}{6} , dx = \frac{1}{6} \cdot \frac{x^2}{2} = \frac{x^2}{12} $$
- The integral of $\frac{2}{3} x^2$ is:
$$ \int \frac{2}{3} x^2 , dx = \frac{2}{3} \cdot \frac{x^3}{3} = \frac{2x^3}{9} $$
- The integral of $\frac{1}{3x}$ is:
$$ \int \frac{1}{3x} , dx = \frac{1}{3} \ln |x| $$
So, combining these results, we have:
$$ \int \frac{x^2 + 4x^3 + 2}{6x} , dx = \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
- Write the final expression
Thus, the final expression for the integral is:
$$ \int \frac{x^2 + 4x^3 + 2}{6x} , dx = \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
The solution to the integral is:
$$ \int \frac{x^2 + 4x^3 + 2}{6x} , dx = \frac{x^2}{12} + \frac{2x^3}{9} + \frac{1}{3} \ln |x| + C $$
More Information
This integral combines polynomial functions with a logarithmic function in its solution. The use of properties of logarithms and basic integration rules are vital to solving such problems.
Tips
- Overlooking constants: Remember to always add the constant of integration, $C$, at the end of indefinite integrals.
- Ignoring the absolute value in logarithmic functions: When integrating $\frac{1}{x}$, always include the absolute value to account for $x$ being negative.
AI-generated content may contain errors. Please verify critical information