∫ (x^2 + 4x^3 + 2) / (6x) dx

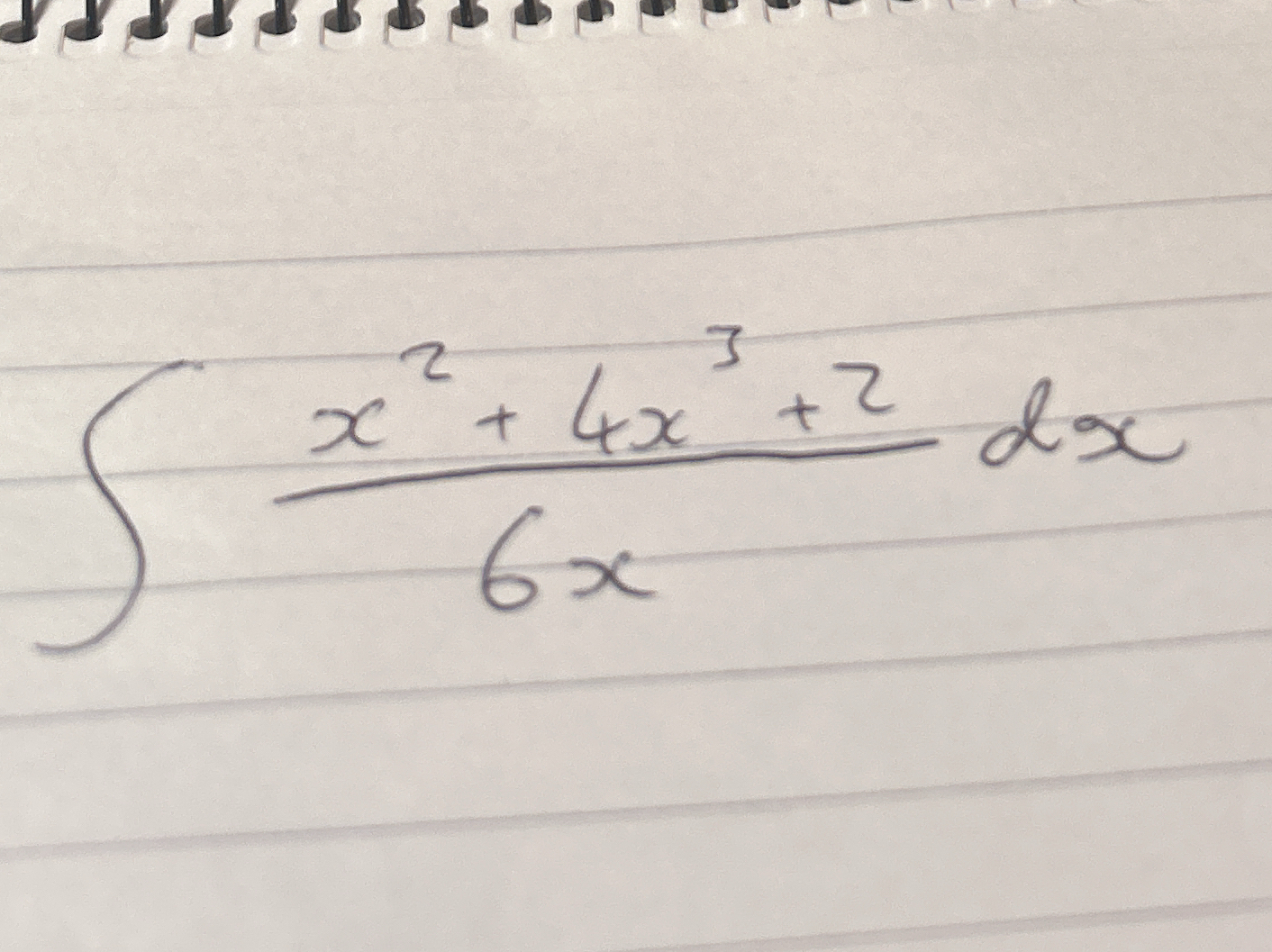
Understand the Problem

The question is asking for the integral of the expression (x^2 + 4x^3 + 2) divided by (6x) with respect to x. This involves both polynomial division and integration.

Answer

The integral is given by: $$ \frac{1}{12}x^2 + \frac{2}{9}x^3 + \frac{1}{3} \ln |x| + C $$

Steps to Solve

Simplify the Expression First, we divide the polynomial in the numerator by the term in the denominator:

$$ \frac{x^2}{6x} + \frac{4x^3}{6x} + \frac{2}{6x} $$

This simplifies to:

$$ \frac{1}{6}x + \frac{2}{3}x^2 + \frac{1}{3x} $$

Write the Integral Now we can express the integral with the simplified terms:

$$ \int \left( \frac{1}{6}x + \frac{2}{3}x^2 + \frac{1}{3x} \right) , dx $$

Integrate Each Term Separately Integrate each term of the integrand:

For $\frac{1}{6}x$, the integral is: $$ \frac{1}{6} \cdot \frac{x^2}{2} = \frac{1}{12}x^2 $$
For $\frac{2}{3}x^2$, the integral is: $$ \frac{2}{3} \cdot \frac{x^3}{3} = \frac{2}{9}x^3 $$
For $\frac{1}{3x}$, the integral is: $$ \frac{1}{3} \ln |x| $$

Combine the Results Now, combine all the results and add the constant of integration $C$:

$$ \int \left( \frac{1}{6}x + \frac{2}{3}x^2 + \frac{1}{3x} \right) , dx = \frac{1}{12}x^2 + \frac{2}{9}x^3 + \frac{1}{3} \ln |x| + C $$

The integral of the given expression is:

$$ \frac{1}{12}x^2 + \frac{2}{9}x^3 + \frac{1}{3} \ln |x| + C $$

More Information

This integral combines polynomial and logarithmic terms. It is essential to isolate each term for proper integration. The constant of integration ($C$) accounts for any constant that could be present in the antiderivative.

Tips

Forgetting to add the constant of integration $C$ at the end.
Not correctly simplifying the division at the beginning.
Misapplying the power rule when integrating.

Thank you for voting!