∫(x^2 + 4x + 2)/(6x) dx
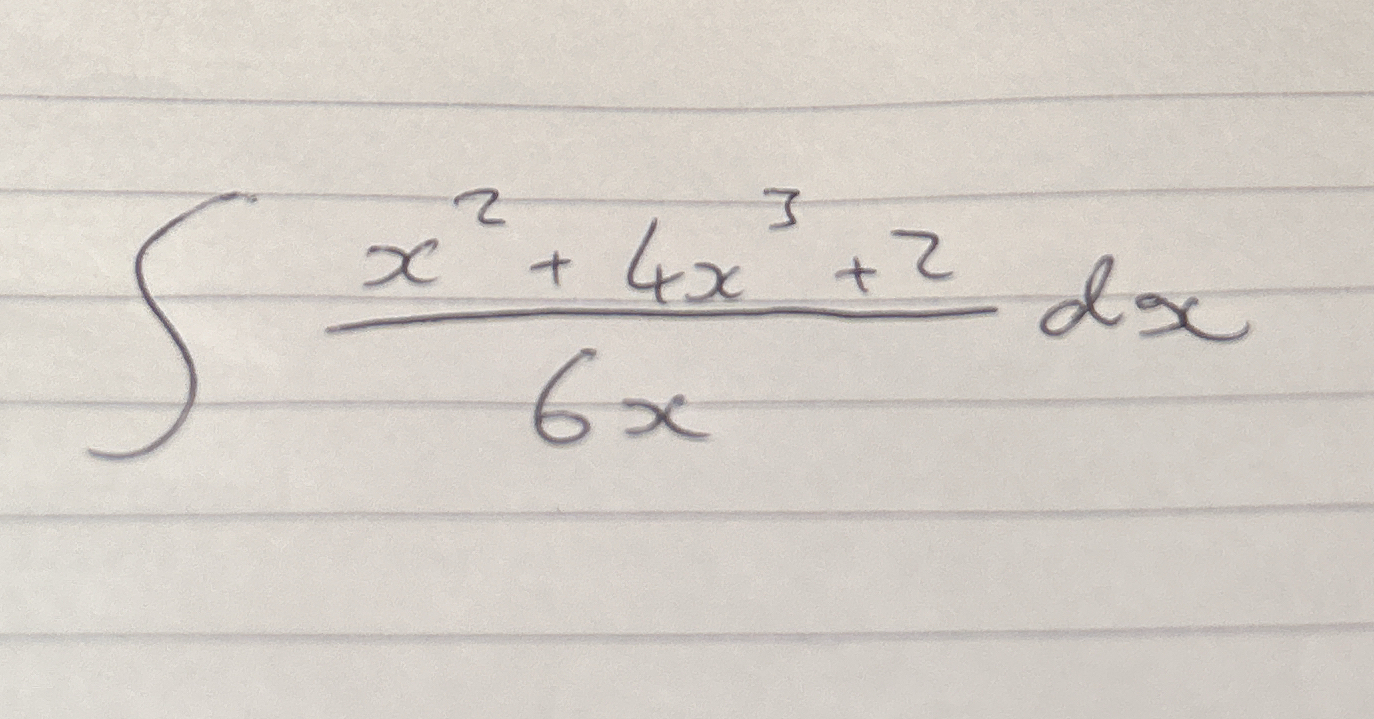
Understand the Problem
The question is asking to evaluate the integral of the expression \( \frac{x^2 + 4x + 2}{6x} \) with respect to \( dx \). This involves performing integration techniques to find the function that represents the area under the curve defined by the given expression.
Answer
The integral evaluates to: $$ \frac{x^2}{12} + \frac{2}{3} x + \frac{1}{3} \ln|x| + C $$
Answer for screen readers
The result of the integral is:
$$ \frac{x^2}{12} + \frac{2}{3} x + \frac{1}{3} \ln|x| + C $$
Steps to Solve
- Simplify the integrand
To simplify the expression ( \frac{x^2 + 4x + 2}{6x} ), we divide each term in the numerator by ( 6x ):
[ \frac{x^2}{6x} + \frac{4x}{6x} + \frac{2}{6x} = \frac{x}{6} + \frac{2}{3} + \frac{1}{3x} ]
- Rewrite the integral
With the simplified expression, we can rewrite the integral:
[ \int \left( \frac{x}{6} + \frac{2}{3} + \frac{1}{3x} \right) dx ]
- Integrate each term
Now, we integrate each term separately:
-
For ( \frac{x}{6} ): [ \int \frac{x}{6} , dx = \frac{1}{6} \cdot \frac{x^2}{2} = \frac{x^2}{12} ]
-
For ( \frac{2}{3} ): [ \int \frac{2}{3} , dx = \frac{2}{3} x ]
-
For ( \frac{1}{3x} ): [ \int \frac{1}{3x} , dx = \frac{1}{3} \ln|x| ]
- Combine the results
Combining all the integrated terms, we get:
[ \int \frac{x^2 + 4x + 2}{6x} , dx = \frac{x^2}{12} + \frac{2}{3} x + \frac{1}{3} \ln|x| + C ]
where ( C ) is the constant of integration.
The result of the integral is:
$$ \frac{x^2}{12} + \frac{2}{3} x + \frac{1}{3} \ln|x| + C $$
More Information
This integral combines basic polynomial integration and a logarithmic integral. Understanding how to simplify rational expressions is crucial for efficient integration.
Tips
- Not simplifying the integrand: Skipping the simplification can lead to more complex integration steps.
- Misapplying integration rules: Ensure each term is integrated properly according to its form.
AI-generated content may contain errors. Please verify critical information