∫ (x² + 4x + 2) / (6x) dx
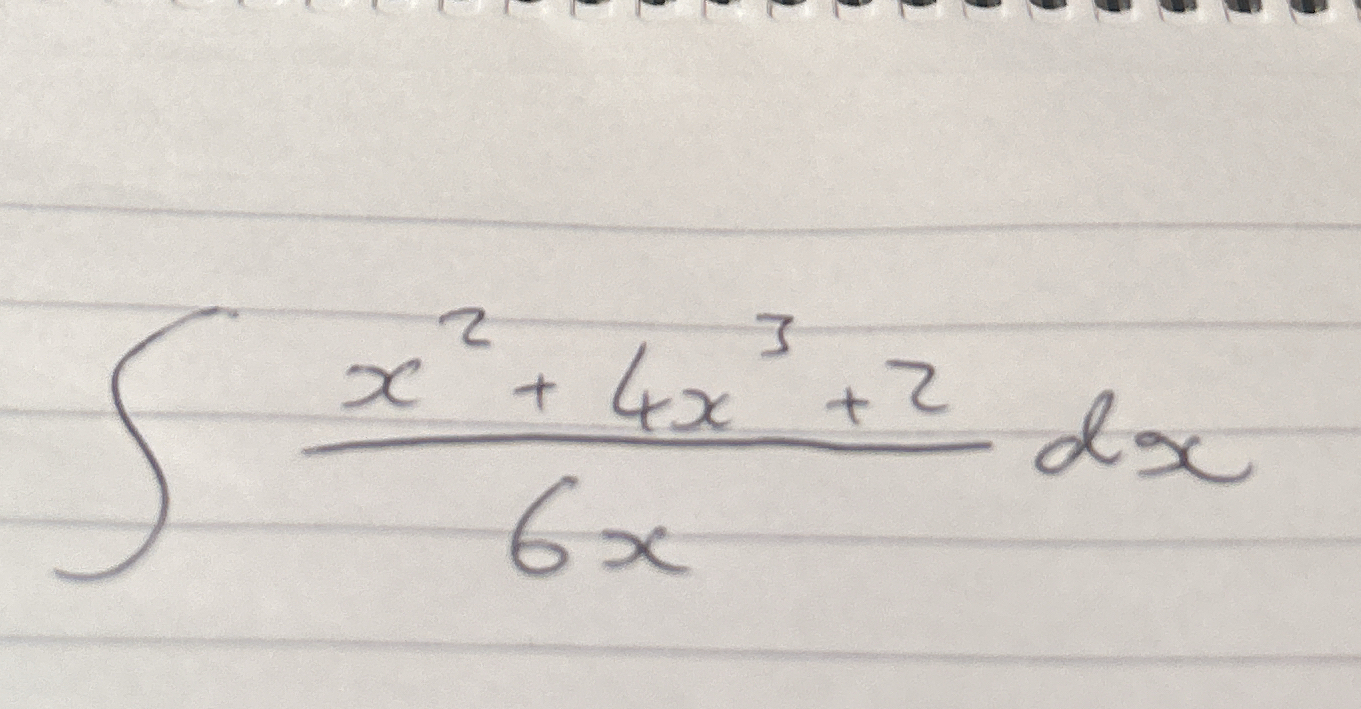
Understand the Problem
The question involves calculating the integral of a rational function expressed as the fraction of a polynomial divided by another polynomial, specifically the integral of (x² + 4x + 2) / (6x) with respect to x.
Answer
$$ \frac{x^2}{12} + \frac{2}{3}x + \frac{1}{3} \ln |x| + C $$
Answer for screen readers
$$ \frac{x^2}{12} + \frac{2}{3}x + \frac{1}{3} \ln |x| + C $$
Steps to Solve
- Simplify the Integrand
First, we will simplify the expression $\frac{x^2 + 4x + 2}{6x}$. We can separate the terms in the numerator:
$$ \frac{x^2}{6x} + \frac{4x}{6x} + \frac{2}{6x} $$
This simplifies to:
$$ \frac{x}{6} + \frac{2}{3} + \frac{1}{3x} $$
- Set Up the Integral
Now, we can set up the integral based on our simplified expression:
$$ \int \left( \frac{x}{6} + \frac{2}{3} + \frac{1}{3x} \right) , dx $$
- Integrate Each Term
Next, we will integrate each term separately:
- The integral of $\frac{x}{6}$ is $\frac{1}{6} \cdot \frac{x^2}{2} = \frac{x^2}{12}$.
- The integral of $\frac{2}{3}$ is $\frac{2}{3}x$.
- The integral of $\frac{1}{3x}$ is $\frac{1}{3} \ln|x|$.
Therefore, the combined integral is:
$$ \int \left( \frac{x}{6} + \frac{2}{3} + \frac{1}{3x} \right) , dx = \frac{x^2}{12} + \frac{2}{3}x + \frac{1}{3} \ln |x| + C $$
- Write the Final Result
Thus, the final result of the integral is:
$$ \frac{x^2}{12} + \frac{2}{3}x + \frac{1}{3} \ln |x| + C $$
$$ \frac{x^2}{12} + \frac{2}{3}x + \frac{1}{3} \ln |x| + C $$
More Information
This integral represents the area under the curve of the function $\frac{x^2 + 4x + 2}{6x}$ over a specified interval. Integrating rational functions often involves simplifying the integrand to make it easier to handle, which is a common technique in calculus.
Tips
- Forgetting to separate the terms in the numerator when simplifying the integrand.
- Miscalculating the integrals of individual terms, especially logarithmic functions.
- Not including the constant of integration (C).
