∫ (x² + 4) / x² dx
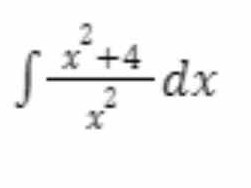
Understand the Problem
The question is asking to evaluate the integral of the function (x² + 4) / x² with respect to x. To solve this, we'll rewrite the expression and perform integration.
Answer
The integral evaluates to $x - \frac{4}{x} + C$.
Answer for screen readers
The final answer for the integral is: $$ x - \frac{4}{x} + C $$
Steps to Solve
- Rewrite the Integral
Start by simplifying the integrand: $$ \frac{x^2 + 4}{x^2} = \frac{x^2}{x^2} + \frac{4}{x^2} = 1 + \frac{4}{x^2} $$ Thus, the integral becomes: $$ \int \left(1 + \frac{4}{x^2}\right) dx $$
- Separate the Integral
Now, separate the integral for easier computation: $$ \int \left(1 + \frac{4}{x^2}\right) dx = \int 1 , dx + \int \frac{4}{x^2} , dx $$
- Integrate Each Term
Now, compute the integrals separately:
- The integral of $1$ is: $$ \int 1 , dx = x $$
- The integral of $\frac{4}{x^2}$ can be rewritten as: $$ \int \frac{4}{x^2} , dx = 4 \int x^{-2} , dx = 4 \left(-\frac{1}{x}\right) = -\frac{4}{x} $$
- Combine the Results
Now, combine the results of the integrals: $$ x - \frac{4}{x} + C $$ where $C$ is the constant of integration.
The final answer for the integral is: $$ x - \frac{4}{x} + C $$
More Information
The integral evaluates a function over the variable $x$. This involves breaking down the function and integrating each part individually, which is a common technique in calculus.
Tips
- Not simplifying the integrand: Always simplify the function before integrating to make the process easier.
- Forgetting the constant of integration: It's essential to include the constant $C$ when performing indefinite integrals.
AI-generated content may contain errors. Please verify critical information