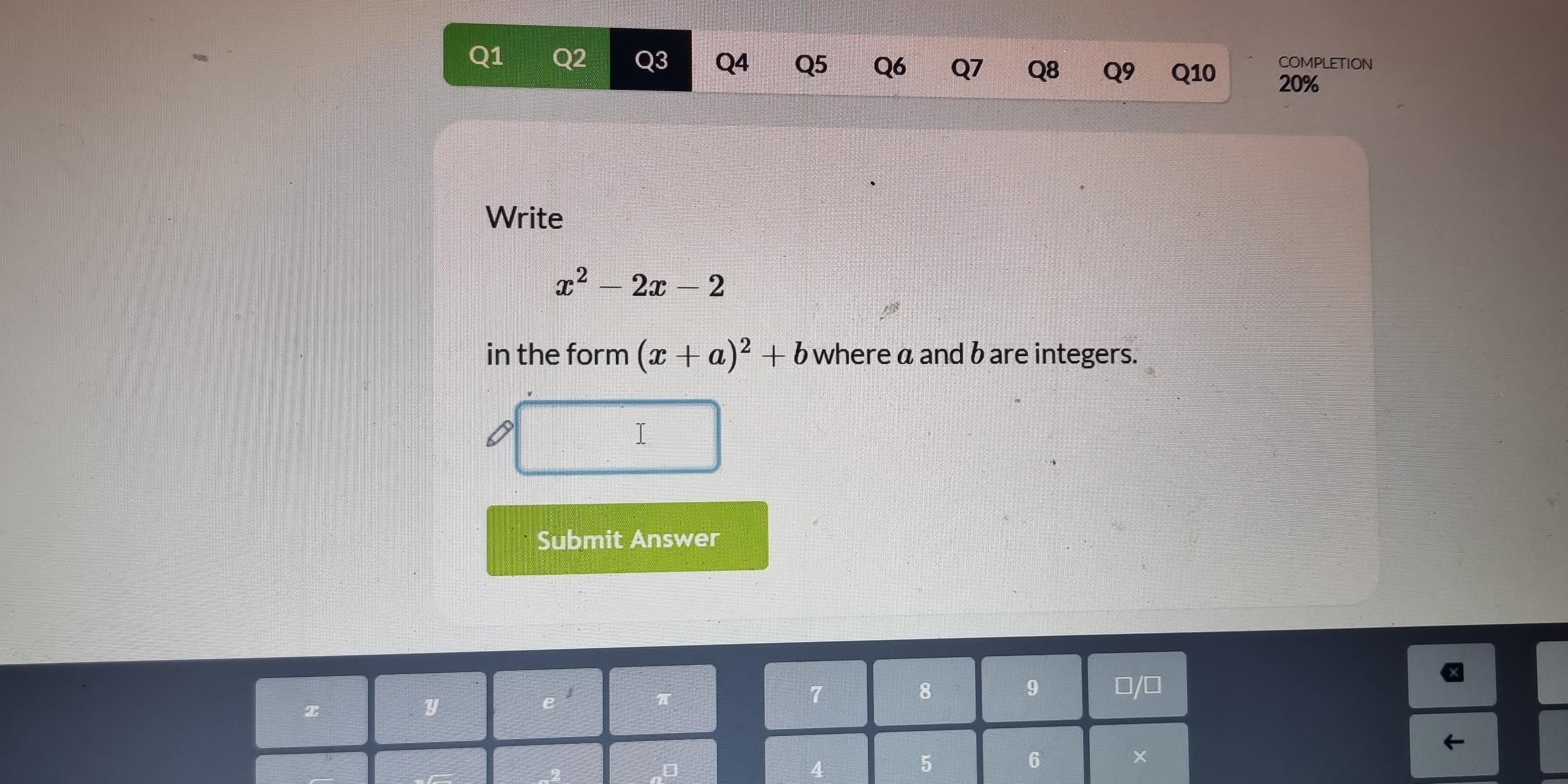
Write x^2 - 2x - 2 in the form (x + α)^2 + b where a and b are integers.
Understand the Problem
The question is asking us to rewrite the quadratic expression x² - 2x - 2 in the form (x + α)² + b, where α and b are integers. This involves completing the square to transform the expression.
Answer
The expression is $(x - 1)^2 - 3$ with $\alpha = -1$ and $b = -3$.
Answer for screen readers
The final expression is
$$(x - 1)^2 - 3$$
with $\alpha = -1$ and $b = -3$.
Steps to Solve
- Identify and Rearrange the Quadratic Expression
Start with the expression:
$$x^2 - 2x - 2$$
We want to complete the square for the terms involving $x$.
- Complete the Square
To complete the square, take the coefficient of $x$, which is $-2$, divide it by $2$ to get $-1$, and then square it:
$$(-1)^2 = 1$$
Now, we can rewrite the quadratic:
$$x^2 - 2x = (x - 1)^2 - 1$$
- Substitute Back into the Expression
Replace the original quadratic expression with the completed square form:
$$(x - 1)^2 - 1 - 2$$
- Simplify
Now, combine the constants:
$$-1 - 2 = -3$$
So we have:
$$(x - 1)^2 - 3$$
- Final Form
The expression is now in the form:
$$(x + \alpha)^2 + b$$
We have $\alpha = -1$ and $b = -3$.
The final expression is
$$(x - 1)^2 - 3$$
with $\alpha = -1$ and $b = -3$.
More Information
Rewriting quadratic expressions by completing the square is a valuable technique in algebra that helps in solving equations, understanding parabolas, and converting to vertex form for analysis.
Tips
- Forgetting to adjust the constant when completing the square. Ensure to subtract or add the squared value only after you rewrite the expression.
- Not recognizing that $\alpha$ corresponds to the linear term's coefficient when completing the square.
AI-generated content may contain errors. Please verify critical information