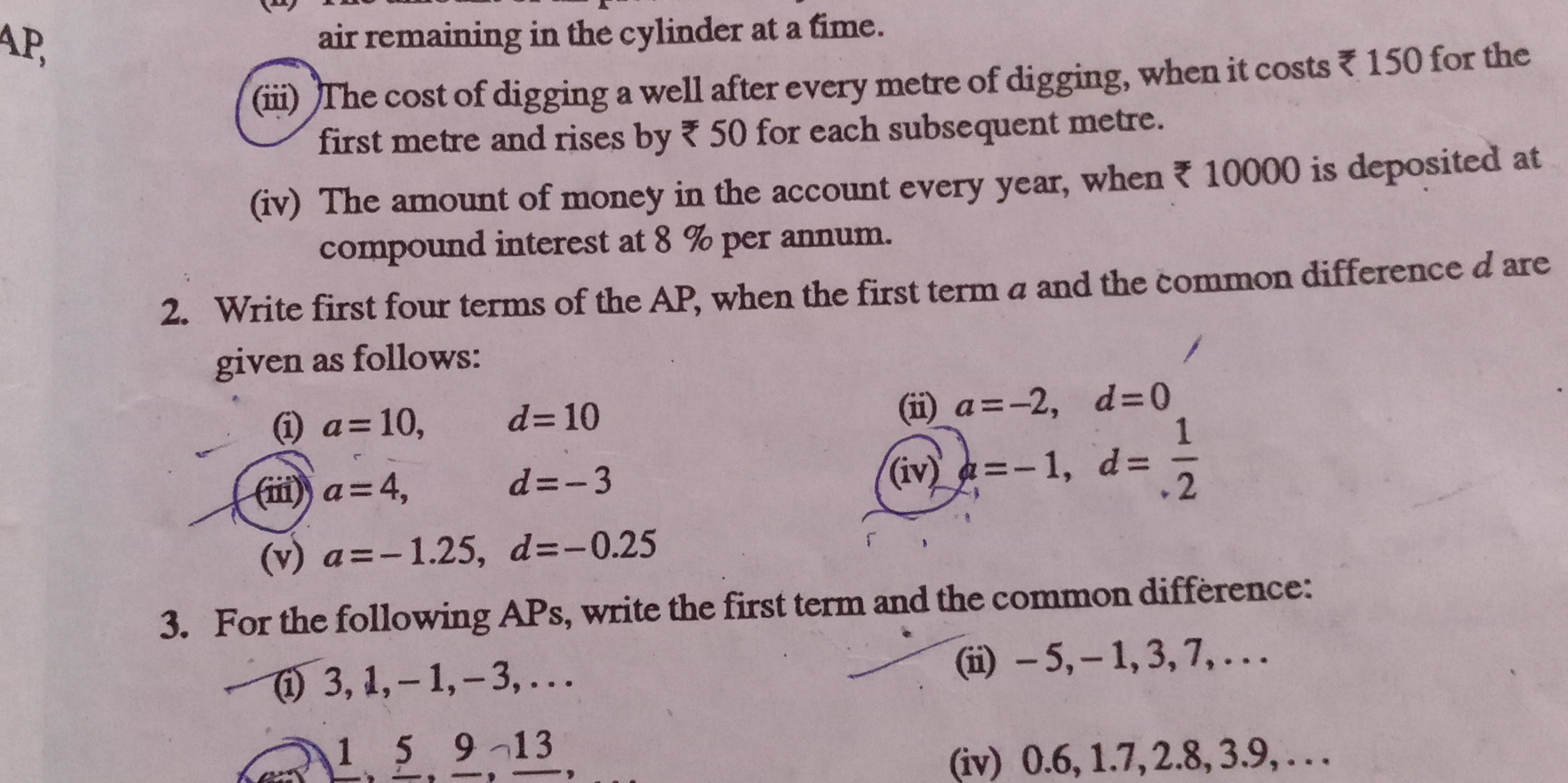
Write first four terms of the AP, when the first term a and the common difference d are given as follows: (i) a=10, d=10 (ii) a=-2, d=0 (iii) a=4, d=-3 (iv) a=-1, d=1/2 (v) a=-1.25... Write first four terms of the AP, when the first term a and the common difference d are given as follows: (i) a=10, d=10 (ii) a=-2, d=0 (iii) a=4, d=-3 (iv) a=-1, d=1/2 (v) a=-1.25, d=-0.25. Read more

Steps to Solve

1. Understanding the Arithmetic Progression (AP)

An Arithmetic Progression is defined by its first term ( a ) and common difference ( d ). The ( n^{th} ) term of an AP can be computed using the formula:
$$ T_n = a + (n-1)d $$

2. Finding the First Term and Common Difference

For each given case, identify the first term ( a ) and the common difference ( d ):

• (i) ( a = 10, d = 10 )
• (ii) ( a = 4, d = -3 )
• (iii) ( a = -1, d = \frac{1}{2} )

3. Calculating the First Four Terms

Now, calculate the first four terms of the AP for each given case as follows:

Case (i):

• First term: ( T_1 = a = 10 )
• Second term: ( T_2 = a + d = 10 + 10 = 20 )
• Third term: ( T_3 = a + 2d = 10 + 2(10) = 30 )
• Fourth term: ( T_4 = a + 3d = 10 + 3(10) = 40 )

Case (ii):

• First term: ( T_1 = a = 4 )
• Second term: ( T_2 = a + d = 4 - 3 = 1 )
• Third term: ( T_3 = a + 2d = 4 + 2(-3) = -2 )
• Fourth term: ( T_4 = a + 3d = 4 + 3(-3) = -5 )

Case (iii):

• First term: ( T_1 = a = -1 )
• Second term: ( T_2 = a + d = -1 + \frac{1}{2} = -0.5 )
• Third term: ( T_3 = a + 2d = -1 + 2 \times \frac{1}{2} = 0 )
• Fourth term: ( T_4 = a + 3d = -1 + 3 \times \frac{1}{2} = 0.5 )