Write an equation of the line below.
Understand the Problem
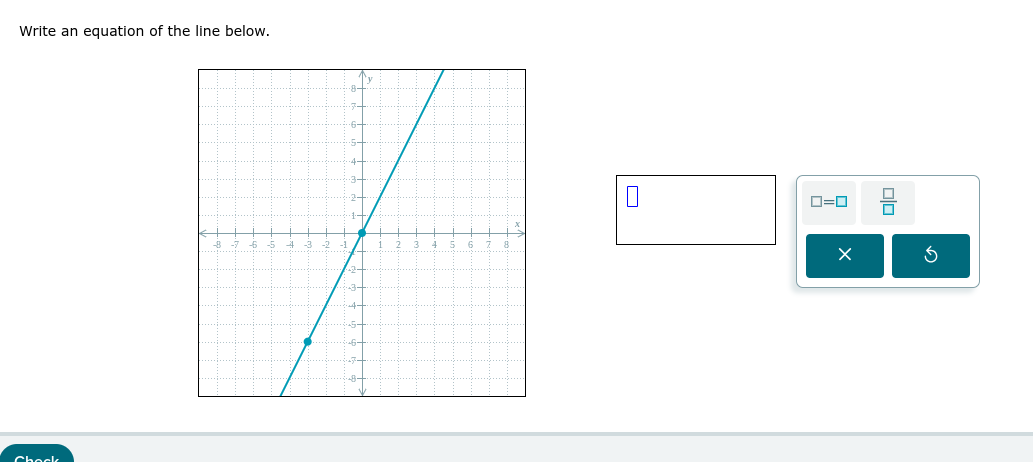
The question asks to write the equation of a line based on its graphical representation provided in the image. The focus is on identifying the slope and y-intercept of the line from the graph.
Answer
The equation of the line is \( y = x - 1 \).
Answer for screen readers
The equation of the line is ( y = x - 1 ).
Steps to Solve
-
Identify the points on the line
Look at the graph and identify at least two clear points. For instance, the points can be (2, 1) and (4, 3). -
Calculate the slope
The slope ($m$) of a line is calculated using the formula:
$$ m = \frac{y_2 - y_1}{x_2 - x_1} $$
Using our identified points (2, 1) and (4, 3):
$$ m = \frac{3 - 1}{4 - 2} = \frac{2}{2} = 1 $$ -
Identify the y-intercept
Find where the line crosses the y-axis. From the graph, it looks like the line crosses the y-axis at (0, -1). Therefore, the y-intercept ($b$) is -1. -
Write the equation of the line
The equation of the line can be expressed in slope-intercept form, which is:
$$ y = mx + b $$
Substituting the values we found:
$$ y = 1x - 1 $$
or simplified to:
$$ y = x - 1 $$
The equation of the line is ( y = x - 1 ).
More Information
In slope-intercept form, the equation of a line is written as ( y = mx + b ), where ( m ) is the slope and ( b ) is the y-intercept. This equation helps us understand how the line behaves in a coordinate system.
Tips
- Confusing the coordinates: Sometimes, people mix up x and y values when identifying points. Always ensure to carefully identify values based on the graph's axes.
- Forgetting to simplify the equation: After determining the line’s equation, it’s important to simplify it if necessary.
AI-generated content may contain errors. Please verify critical information