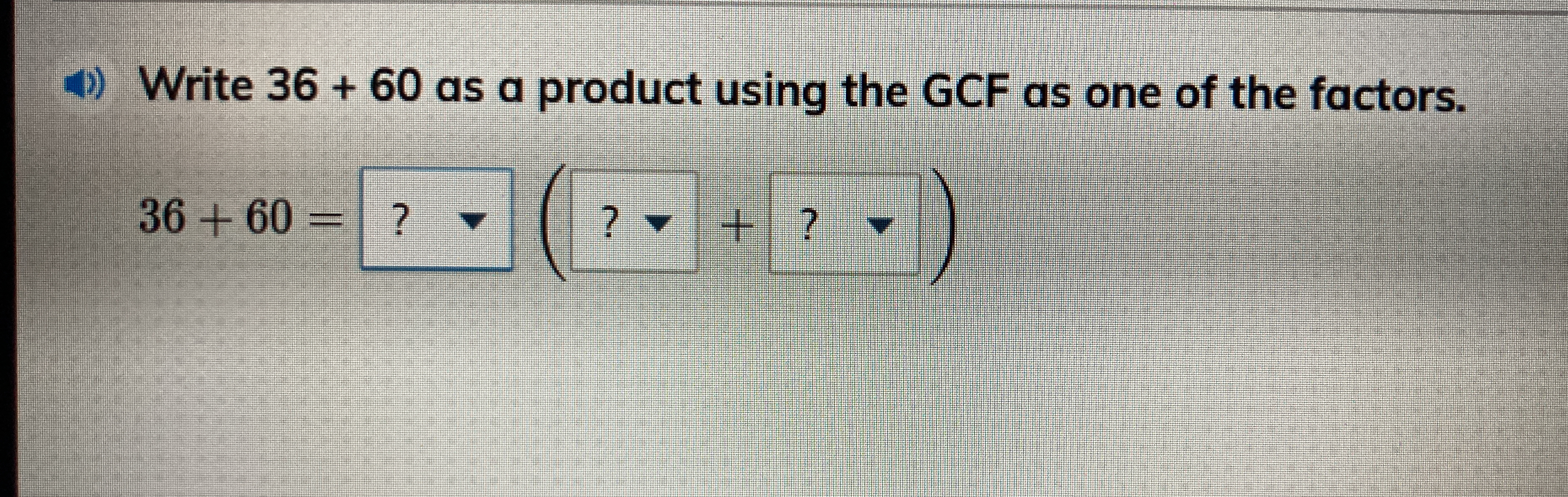
Write 36 + 60 as a product using the GCF as one of the factors.
Understand the Problem
The question is asking to express the sum of 36 and 60 as a product, utilizing the Greatest Common Factor (GCF) as one of the factors. To solve this, we first need to determine the GCF of 36 and 60, and then rewrite the expression in the form of GCF multiplied by the sum of the other factors.
Answer
$$ 36 + 60 = 12 \times (3 + 5) $$
Answer for screen readers
$$ 36 + 60 = 12 \times (3 + 5) $$
Steps to Solve
- Determine the GCF of 36 and 60
To find the GCF, we can list the factors of each number.
Factors of 36: 1, 2, 3, 4, 6, 9, 12, 18, 36
Factors of 60: 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, 30, 60
The greatest common factor is 12.
- Express the sum in terms of the GCF
We can express $36 + 60$ as $GCF \times (x + y)$ where $x$ and $y$ are the factors we need to determine.
- Find integers that work
We know: $$ 36 + 60 = 96 $$
Let’s factor this using the GCF: $$ 96 = 12 \times (x + y) $$
Thus, we can write: $$ x + y = \frac{96}{12} $$
- Calculate the values of $x$ and $y$
Dividing gives us: $$ x + y = 8 $$
A possible set of integers could be $x = 3$ and $y = 5$ (since $3 + 5 = 8$).
- Final expression
Now, we can rewrite the sum: $$ 36 + 60 = GCF \times (x + y) = 12 \times (3 + 5) $$
$$ 36 + 60 = 12 \times (3 + 5) $$
More Information
The GCF of 36 and 60 is 12. By expressing their sum this way, we can understand how to break down addition into a product using the GCF as a foundational factor.
Tips
- Misidentifying the GCF. Always list factors or use factorization techniques to ensure accuracy.
- Forgetting to simplify or express integers correctly in the sum once the GCF is found.
AI-generated content may contain errors. Please verify critical information