Which system of equations does the graph represent?
Understand the Problem
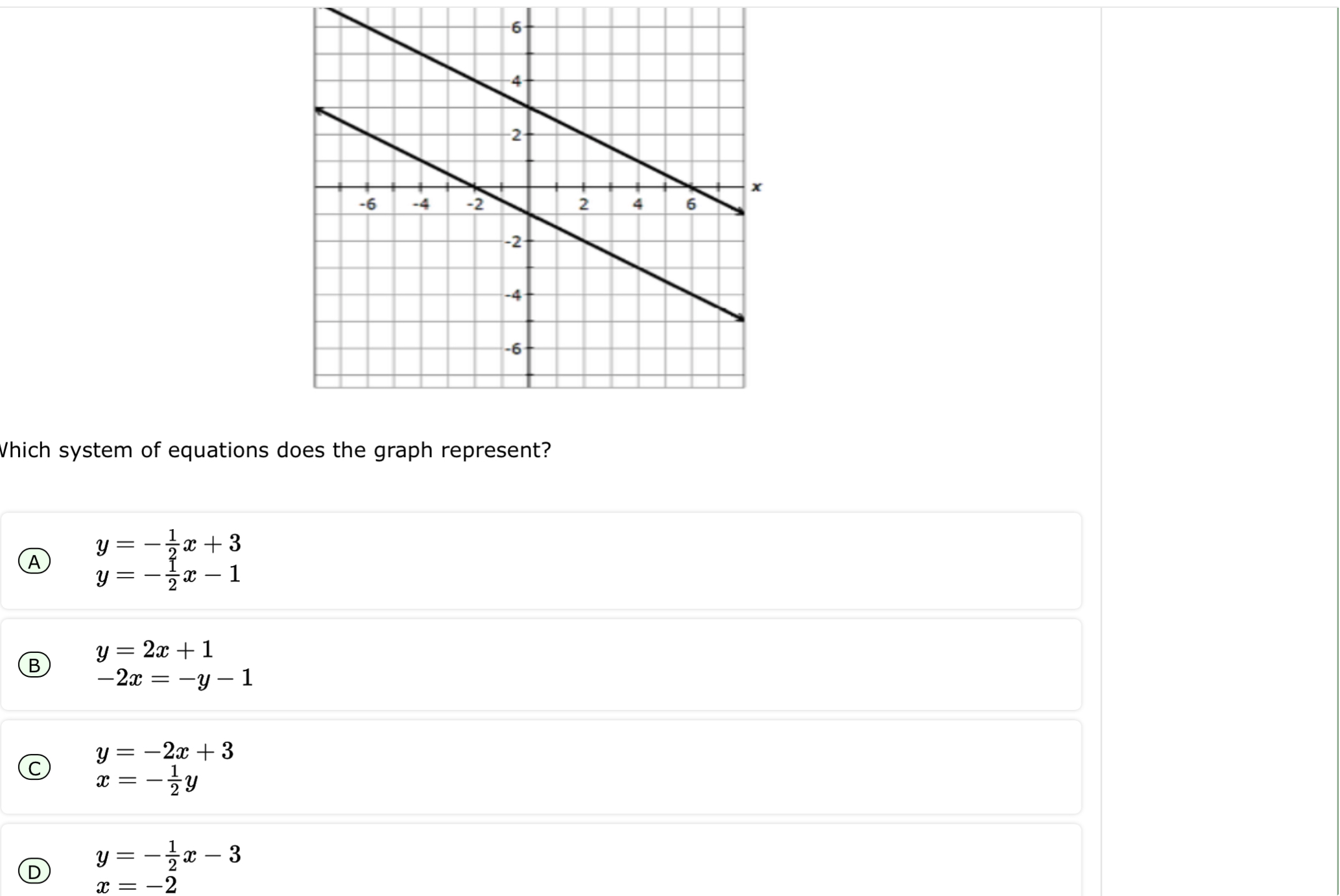
The question is asking which system of equations corresponds to the given graph. The approach involves analyzing the graph to determine the equations of the lines and matching them with the provided options.
Answer
The correct option is A: $$ y = -\frac{1}{2}x + 3 $$ $$ y = -\frac{1}{2}x - 1 $$
Answer for screen readers
The correct system of equations represented by the graph is: $$ y = -\frac{1}{2}x + 3 $$ $$ y = -\frac{1}{2}x - 1 $$
Steps to Solve
-
Identify the slope and y-intercept of the first line
- Observe the first line from the graph. It appears to pass through points such as (0, 3) and (2, 2).
- The slope ($m_1$) can be calculated as: $$ m_1 = \frac{y_2 - y_1}{x_2 - x_1} = \frac{2 - 3}{2 - 0} = -\frac{1}{2} $$
- Thus, the equation can be expressed in slope-intercept form as: $$ y = -\frac{1}{2}x + 3 $$
-
Identify the slope and y-intercept of the second line
- Now, observe the second line. It passes through points like (0, -1) and (2, -2).
- The slope ($m_2$) can be calculated as: $$ m_2 = \frac{-2 - (-1)}{2 - 0} = -\frac{1}{2} $$
- Thus, the equation in slope-intercept form becomes: $$ y = -\frac{1}{2}x - 1 $$
-
Compare with answer choices
- Now, we compare our derived equations with the provided options:
- Option A: $$ y = -\frac{1}{2}x + 3 $$ $$ y = -\frac{1}{2}x - 1 $$
- This matches our derived equations from the graph.
- Now, we compare our derived equations with the provided options:
-
Evaluate other options for verification
- Option B: $y = 2x + 1$ and $-2x = -y - 1$ does not produce matching equations for the slope and intercept observed in the graph.
- Option C and D are similarly mismatched on slopes and intercepts.
The correct system of equations represented by the graph is: $$ y = -\frac{1}{2}x + 3 $$ $$ y = -\frac{1}{2}x - 1 $$
More Information
Finding the equations of lines from their graphs involves determining their slopes and y-intercepts. This system reflects two parallel lines, each having the same slope but different y-intercepts.
Tips
- Mistaking the slope from the graph, leading to incorrect equations.
- Not checking all given options thoroughly, which can lead to overlooking the correct answer.
AI-generated content may contain errors. Please verify critical information