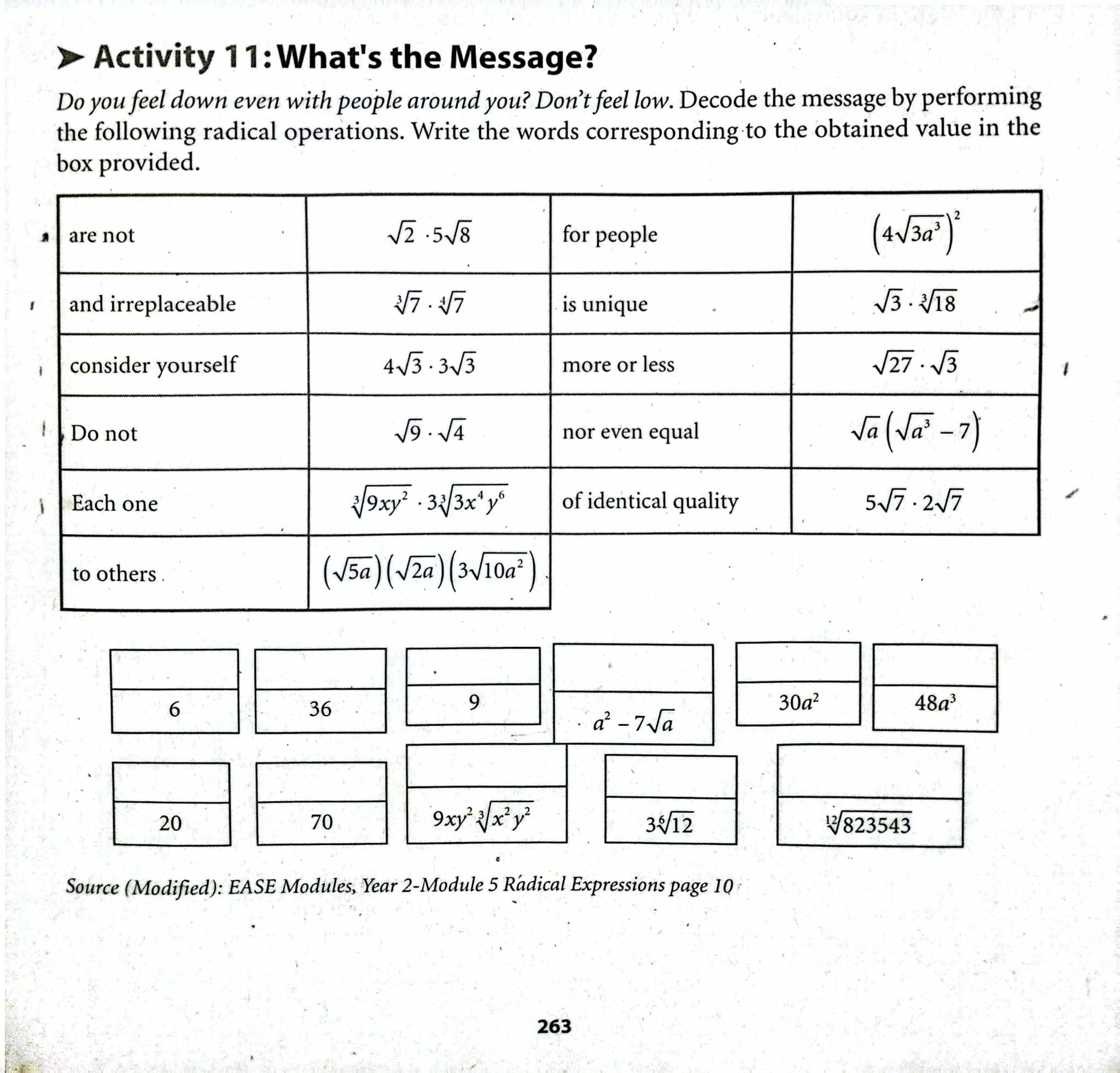
What's the message? Decode the message by performing the following radical operations. Write the words corresponding to the obtained value in the box provided.

Steps to Solve

1. Calculate ( \sqrt{2} \cdot \sqrt{5/8} )

First, multiply the square roots: $$ \sqrt{2} \cdot \sqrt{5/8} = \sqrt{2 \cdot \frac{5}{8}} = \sqrt{\frac{10}{8}} = \sqrt{\frac{5}{4}} = \frac{\sqrt{5}}{2} $$

2. Calculate ( \sqrt{7} \cdot \sqrt{7} )

Next, simplify: $$ \sqrt{7} \cdot \sqrt{7} = 7 $$

3. Calculate ( 4\sqrt{3} \cdot 3\sqrt{3} )

Now, multiply: $$ 4\sqrt{3} \cdot 3\sqrt{3} = 12 \cdot 3 = 36 $$

4. Calculate ( \sqrt{9} \cdot \sqrt{4} )

Then, simplify: $$ \sqrt{9} \cdot \sqrt{4} = 3 \cdot 2 = 6 $$

5. Calculate ( \sqrt{gxy^2} \cdot \sqrt{3} \cdot \sqrt{3x^6} )

Combine the square roots: $$ \sqrt{g \cdot x^2 \cdot 3 \cdot 3x^6} = \sqrt{9x^8y^6g} = 3x^4y^3\sqrt{g} $$

6. Calculate ( \left(\sqrt{5a}\right) \cdot \left(\sqrt{2}\right) \cdot \left(\sqrt{30a^2}\right) )

Now compute: $$ \sqrt{5a} \cdot \sqrt{2} \cdot \sqrt{30a^2} = \sqrt{5a \cdot 2 \cdot 30a^2} = \sqrt{300a^3} = 10\sqrt{3}a^{3/2} $$

7. Calculate ( \sqrt{27} \cdot \sqrt{3} )

Lastly, simplify: $$ \sqrt{27} \cdot \sqrt{3} = \sqrt{81} = 9 $$