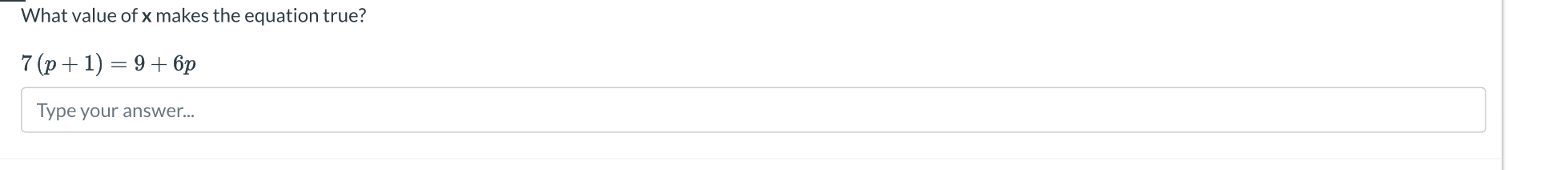
What value of p makes the equation true? 7(p + 1) = 9 + 6p
Understand the Problem
The question is asking for the value of p that satisfies the equation 7(p + 1) = 9 + 6p. This involves solving for the variable p by isolating it on one side of the equation.
Answer
The value of $p$ is $2$.
Answer for screen readers
The value of $p$ that satisfies the equation is $2$.
Steps to Solve
-
Distribute the left side of the equation
Multiply 7 with each term inside the parentheses:
$$ 7(p + 1) = 7p + 7 $$
So the equation becomes:
$$ 7p + 7 = 9 + 6p $$ -
Move the variable terms to one side
Subtract $6p$ from both sides to isolate the variable on one side:
$$ 7p + 7 - 6p = 9 $$
This simplifies to:
$$ p + 7 = 9 $$ -
Isolate the variable
Now, subtract 7 from both sides to solve for $p$:
$$ p = 9 - 7 $$
This simplifies to:
$$ p = 2 $$
The value of $p$ that satisfies the equation is $2$.
More Information
The equation represents a linear relationship, and solving for $p$ helps to show the point at which both sides of the equation are equal. This is a common type of problem in algebra, illustrating how to isolate a variable.
Tips
- Forgetting to distribute properly when expanding the left side.
- Not combining like terms accurately.
- Miscalculating when moving terms from one side to another.
AI-generated content may contain errors. Please verify critical information