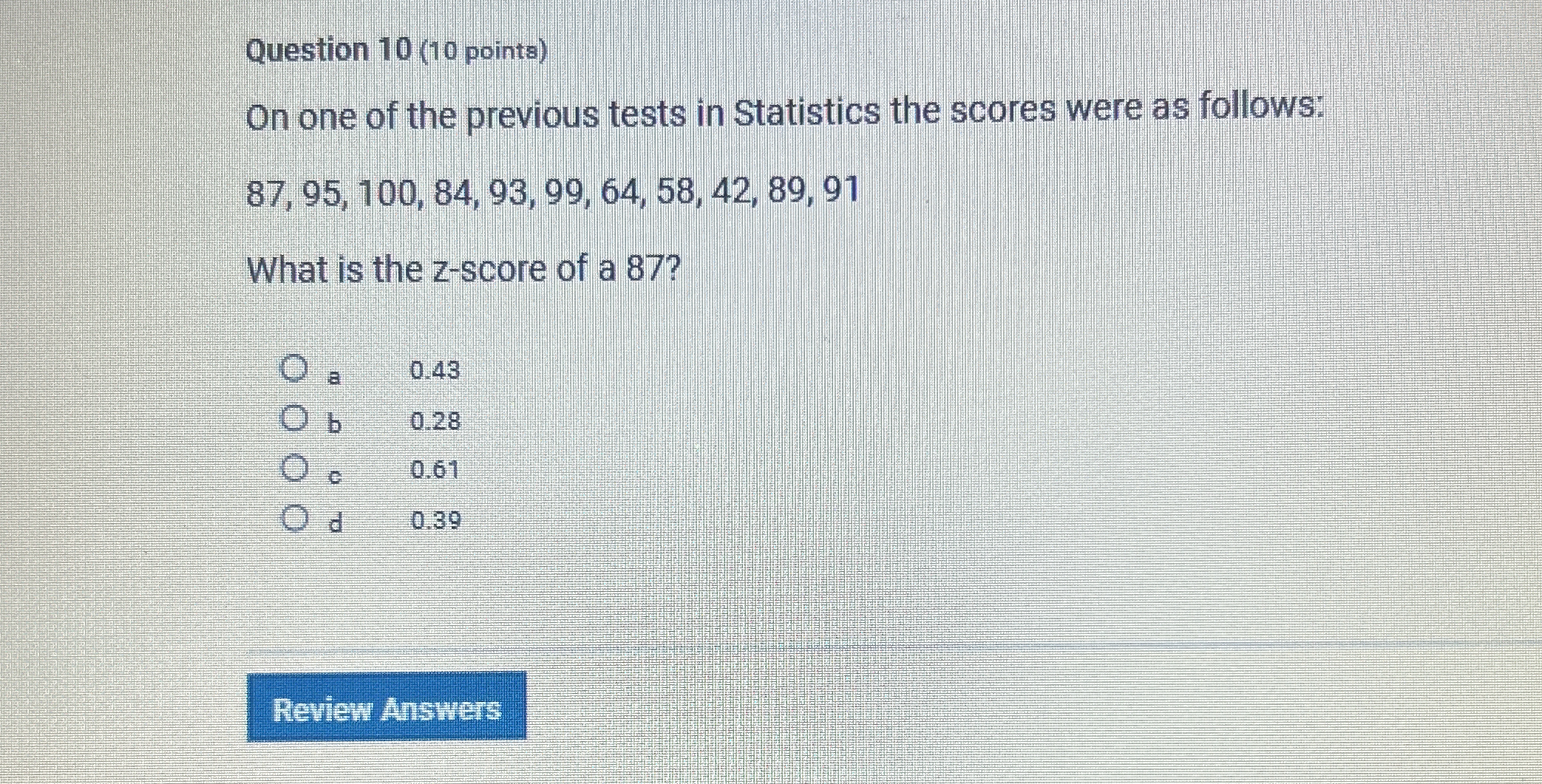
What is the z-score of a 87?

Steps to Solve

1. Calculate the Mean To find the mean ($\mu$), sum all the scores and divide by the number of scores.
   The scores are: 87, 95, 100, 84, 93, 99, 64, 58, 42, 89, 91.

$$ \mu = \frac{87 + 95 + 100 + 84 + 93 + 99 + 64 + 58 + 42 + 89 + 91}{11} $$ Calculating the sum:
$$ \text{Sum} = 87 + 95 + 100 + 84 + 93 + 99 + 64 + 58 + 42 + 89 + 91 = 1001 $$
Thus,
$$ \mu = \frac{1001}{11} = 91 $$

2. Calculate the Standard Deviation Next, find the standard deviation ($\sigma$). Subtract the mean from each score, square the result, sum these squares, then divide by the number of scores, and take the square root.

Calculating each squared difference:

• $(87 - 91)^2 = 16$
• $(95 - 91)^2 = 16$
• $(100 - 91)^2 = 81$
• $(84 - 91)^2 = 49$
• $(93 - 91)^2 = 4$
• $(99 - 91)^2 = 64$
• $(64 - 91)^2 = 729$
• $(58 - 91)^2 = 1089$
• $(42 - 91)^2 = 2401$
• $(89 - 91)^2 = 4$
• $(91 - 91)^2 = 0$

Now sum the squared differences:
$$ \text{Sum of squares} = 16 + 16 + 81 + 49 + 4 + 64 + 729 + 1089 + 2401 + 4 + 0 = 6359 $$

Calculate the variance:
$$ \text{Variance} = \frac{6359}{11} \approx 577.18 $$

And the standard deviation:
$$ \sigma = \sqrt{577.18} \approx 24.04 $$

3. Calculate the Z-Score Now apply the z-score formula:

$$ z = \frac{X - \mu}{\sigma} $$ Where $X = 87$, $\mu = 91$, and $\sigma \approx 24.04$.

Plug the values in:
$$ z = \frac{87 - 91}{24.04} \approx \frac{-4}{24.04} \approx -0.17 $$

4. Interpret the Result The calculated z-score indicates how many standard deviations the score of 87 is below the mean.