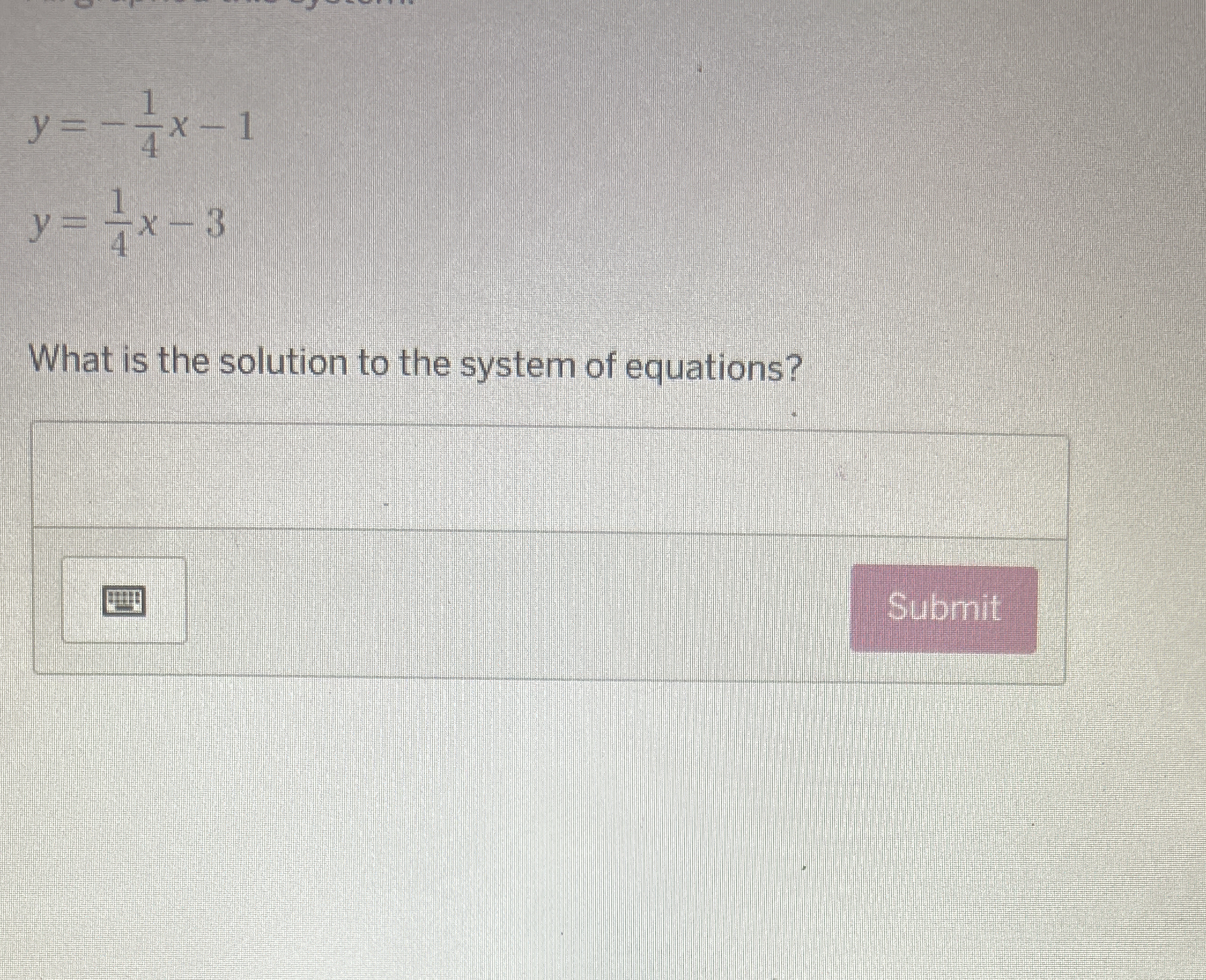
What is the solution to the system of equations? y = -1/4x - 1 and y = 1/4x - 3
Understand the Problem
The question is asking for the solution to a system of equations involving two linear equations. We will solve the equations either by substitution or elimination method to find the values of x and y.
Answer
The solution is $(x, y) = (8, -3)$.
Answer for screen readers
The solution to the system of equations is $(8, -3)$.
Steps to Solve
-
Set the equations equal to each other Since both equations are equal to $y$, we can set them equal to each other: $$ -\frac{1}{4}x - 1 = \frac{1}{4}x - 3 $$
-
Combine like terms Add $\frac{1}{4}x$ to both sides to eliminate $x$ on the right side: $$ -\frac{1}{4}x + \frac{1}{4}x - 1 = -3 $$
This simplifies to: $$ -1 = \frac{1}{4}x - 3 $$
Next, we'll add 3 to both sides: $$ -1 + 3 = \frac{1}{4}x $$
This gives us: $$ 2 = \frac{1}{4}x $$
-
Solve for $x$ To isolate $x$, multiply both sides by 4: $$ x = 8 $$
-
Substitute $x$ back to find $y$ Now, substitute $x = 8$ into one of the original equations. We'll use the first equation: $$ y = -\frac{1}{4}(8) - 1 $$
Calculating this: $$ y = -2 - 1 = -3 $$
- Final answer Thus, the solution to the system of equations is: $$(x, y) = (8, -3)$$
The solution to the system of equations is $(8, -3)$.
More Information
This solution is the point where the two lines represented by the equations intersect. The algebraic method of setting equations equal is a common approach when solving systems of equations.
Tips
- Mixing up the signs when combining like terms.
- Forgetting to isolate $x$ fully before substituting back to find $y$.
AI-generated content may contain errors. Please verify critical information