What is the solution for the equation?
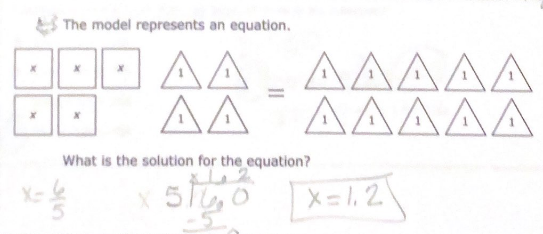
Understand the Problem
The question is asking for the solution to an equation represented by a model consisting of variables and numbers. The user is to determine the value of x based on the given representation.
Answer
The solution for the equation is $x = 4$.
Answer for screen readers
The solution for the equation is $x = 4$.
Steps to Solve
- Simplify the left side of the equation
The left side of the equation is given by $x + x + (1 + 1)$.
First, simplify $(1 + 1)$: $$ 1 + 1 = 2 $$ Now the left side becomes: $$ x + x + 2 = 2x + 2 $$
- Simplify the right side of the equation
The right side consists of ten 1s: $$ (1 + 1 + 1 + 1 + 1 + 1 + 1 + 1 + 1 + 1) = 10 $$
- Set up the equation
Equate the simplified left and right sides: $$ 2x + 2 = 10 $$
- Isolate the variable x
Subtract 2 from both sides: $$ 2x = 10 - 2 $$ $$ 2x = 8 $$
- Solve for x
Divide both sides by 2: $$ x = \frac{8}{2} $$ $$ x = 4 $$
The solution for the equation is $x = 4$.
More Information
This equation demonstrates basic algebraic principles, specifically the concept of combining like terms, simplifying expressions, and solving for a variable. It's a straightforward example of how to manipulate algebraic equations to isolate the unknown.
Tips
- Miscalculating the sum of the numbers on either side of the equation. Review addition and make sure to accurately add.
- Forgetting to divide by the coefficient of x when isolating it. Always double-check each step for accuracy.
AI-generated content may contain errors. Please verify critical information