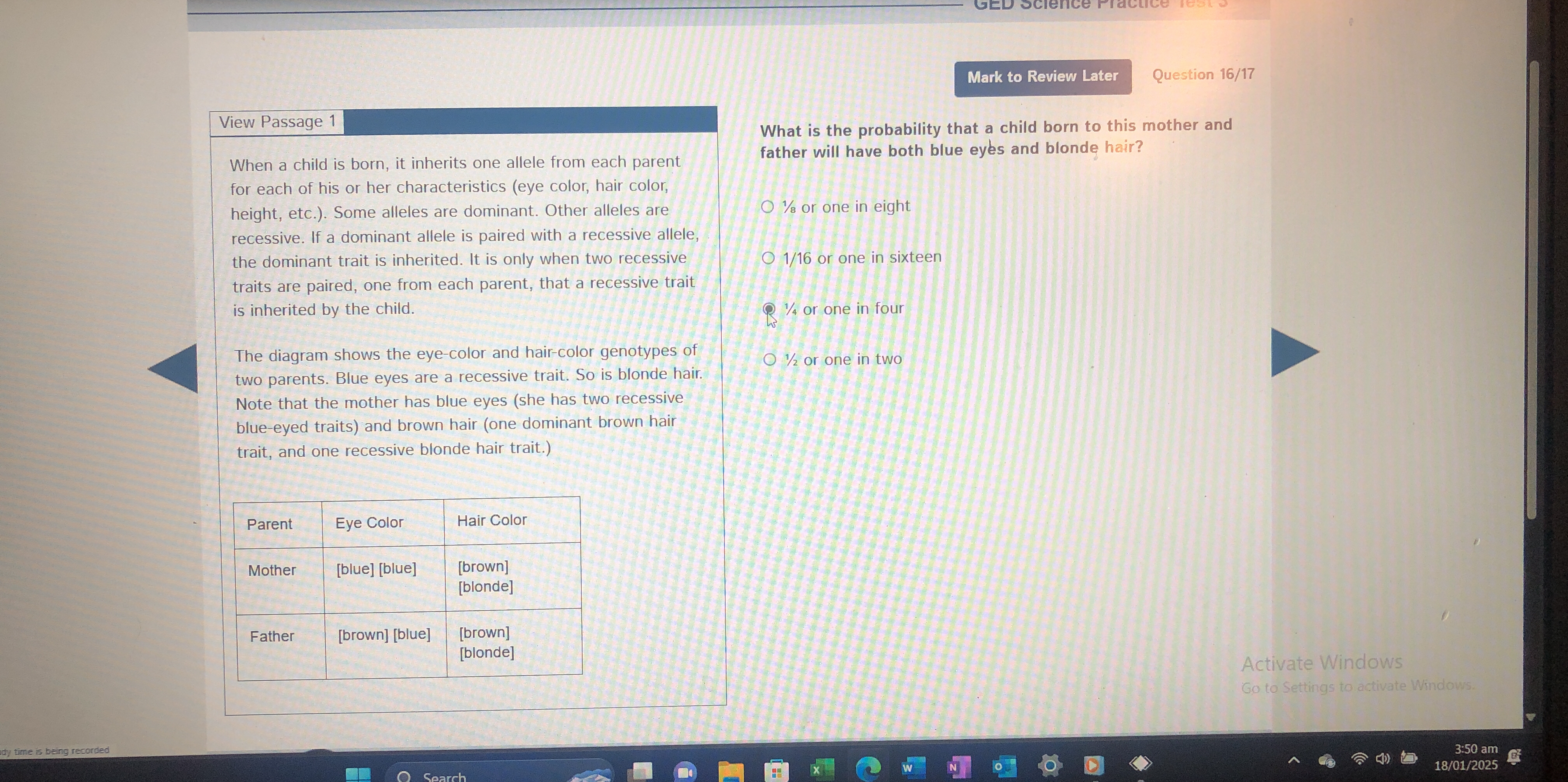
What is the probability that a child born to this mother and father will have both blue eyes and blonde hair?

Steps to Solve

1. Identify the Parents' Genotypes The mother has blue eyes (genotype: $bb$) and the hair color genotype is brown (dominant) and blonde (recessive). Thus, she can either be $Bb$ (brown hair) or $bb$ (blonde hair). The father has brown eyes (dominant) and can have the genotype $Bb$ (blue or blonde hair) or $BB$. But since he has blue eyes, he must be $Bb$. Therefore, the mother's genotype for hair color can be assumed to be $Bb$ for diversity.

2. Determine Possible Alleles for Each Trait

   • For eye color:

     • Mother: $bb$ (blue eyes)
     • Father: $Bb$ (could either pass $B$ or $b$)

   • For hair color:

     • Mother: $Bb$ (could either pass $B$ or $b$)
     • Father: $Bb$ (could either pass $B$ or $b$)

3. Calculate Possible Combinations for Eye Color

   • From the mother, only $b$ can be passed (blue eyes).
   • From the father, he can pass either $B$ or $b$:
     • $b$ (mother) + $B$ (father): $Bb$ (brown eyes)
     • $b$ (mother) + $b$ (father): $bb$ (blue eyes)

   The probability for blue eyes is therefore $1/2$.

4. Calculate Possible Combinations for Hair Color

   • Mother ($Bb$) can pass either $B$ (brown) or $b$ (blonde).
   • Father ($Bb$) can also pass either $B$ (brown) or $b$ (blonde).

   Possible combinations for hair color:

   • $B, B$: Brown hair
   • $B, b$: Brown hair
   • $b, B$: Brown hair
   • $b, b$: Blonde hair

   The probability for blonde hair is $1/4$.

5. Combine Probabilities for Both Traits We need both blue eyes and blonde hair. So, we multiply the probabilities:

   • Probability for blue eyes: $1/2$
   • Probability for blonde hair: $1/4$

   Therefore, the combined probability is: $$ P(\text{both}) = P(\text{blue eyes}) \times P(\text{blonde hair}) = \frac{1}{2} \times \frac{1}{4} = \frac{1}{8} $$